accounting for LED resistance

Question

I'm doing a simple lab (I'm a hobby EE) to reinforce my ohm's law math and learn a little about how to do proper measurements with a multimeter.

I have simple circuit with a 2.2k ohm resistor connected in series with an LED. Everything works fine up to the point I go to calculate voltage drop across the resistor and LED.

My initial calculations only accounted for the 2.2k ohm resistor. As such I got the full voltage dropped across the resistor. However, when I measured the circuit for real I found the result to be nearly half of the input voltage, which would indicate to me

1. My math is wrong
2. There's resistance left unaccounted for

The only thing left to account for is the LED. What is the best method for determining the resistance of a simple LED? I tried doing what I do with resistors (hold it up to the probes with my fingers) but I don't get a proper reading. Is there a technique I'm missing here?

Answer 1

LED's aren't best modeled as a pure resistor. As noted in some other answers, real LED's do have resistance, but often that's not the primary concern when modeling a diode. An LED's current/voltage relationship graph:

Now this behavior is quite difficult to calculate by hand (especially for complicated circuits), but there is a good "approximation" which splits the diode into 3 discrete modes of operation:

• If the voltage across the diode is greater than Vd, the diode behaves like a constant voltage drop (i.e. it will allow whatever current through to maintain V = Vd).

• If the voltage is less than Vd but greater than the breakdown voltage Vbr, the diode doesn't conduct.

• If the reverse bias voltage is above the breakdown voltage Vbr, the diode again becomes conducting, and will allow whatever current through to maintain V = Vbr.

So let's suppose we have some circuit:

^{simulate this circuit – Schematic created using CircuitLab}

First, we're going to assume that VS > Vd. That means the voltage across R is VR = VS - Vd.

Using Ohm's law, we can tell that the current flowing through R (and thus D) is:

\begin{equation} I = \frac{V_R}{R} \end{equation}

Let's plug some numbers in. Say VS = 5V, R=2.2k, Vd=2V (a typical red LED).

\begin{equation} V_R = 5V - 2V = 3V\\ I = \frac{3V}{2.2k\Omega} = 1.36 mA \end{equation}

Ok, what if VS = 1V, R = 2.2k, and Vd = 2V?

This time, VS < Vd, and the diode doesn't conduct. There's no current flowing through R, so VR = 0V. That means VD = VS = 1V (here, VD is the actual voltage across D, where-as Vd is the saturation voltage drop of the diode).

Answer 2

Contrary to some of the other answers, LEDs do have resistance. It's small, but not insignificant. The resistance alone isn't enough to characterize their behavior, but to say that LEDs have no resistance is a valid simplification only sometimes.

See, for example, this graph from the datasheet for LTL-307EE, which I picked for no reason other than it's the default diode in CircuitLab, and a pretty typical indicator LED:

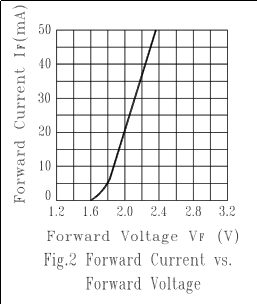
See how the line is essentially straight, and not vertical above 5mA? That's due to the LED's internal resistance. This is the sum of the resistance of the leads, the bond wires, and the silicon.

An LED without resistance has an exponential relationship between current \$I\$ and voltage \$V_D\$, according to the Schockley diode equation:

$$ I=I_S\left(e^{V_D/(nV_T)}-1\right) $$

I won't bore you with the definitions of all the terms: read more on Wikipedia if you want to know. Just know that they are constants for a given LED. Look at the \$I\$ and \$V_D\$ terms and see how they are exponentially related. For this example, I picked \$V_T=25.85\cdot10^{-3}\$, \$n=1\$, and \$i_s = 10^{-33}\$.

Consider the current-voltage relationship for a resistor, which is given by Ohm's law:

$$ I = \frac{V}{R} $$

Clearly they are linearly related. If you were to graph this current-voltage relationship for a resistor as the datasheet above does for the LED, you would get a straight line, passing through \$0V, 0A\$, and the slope of this line is the resistance \$R\$.

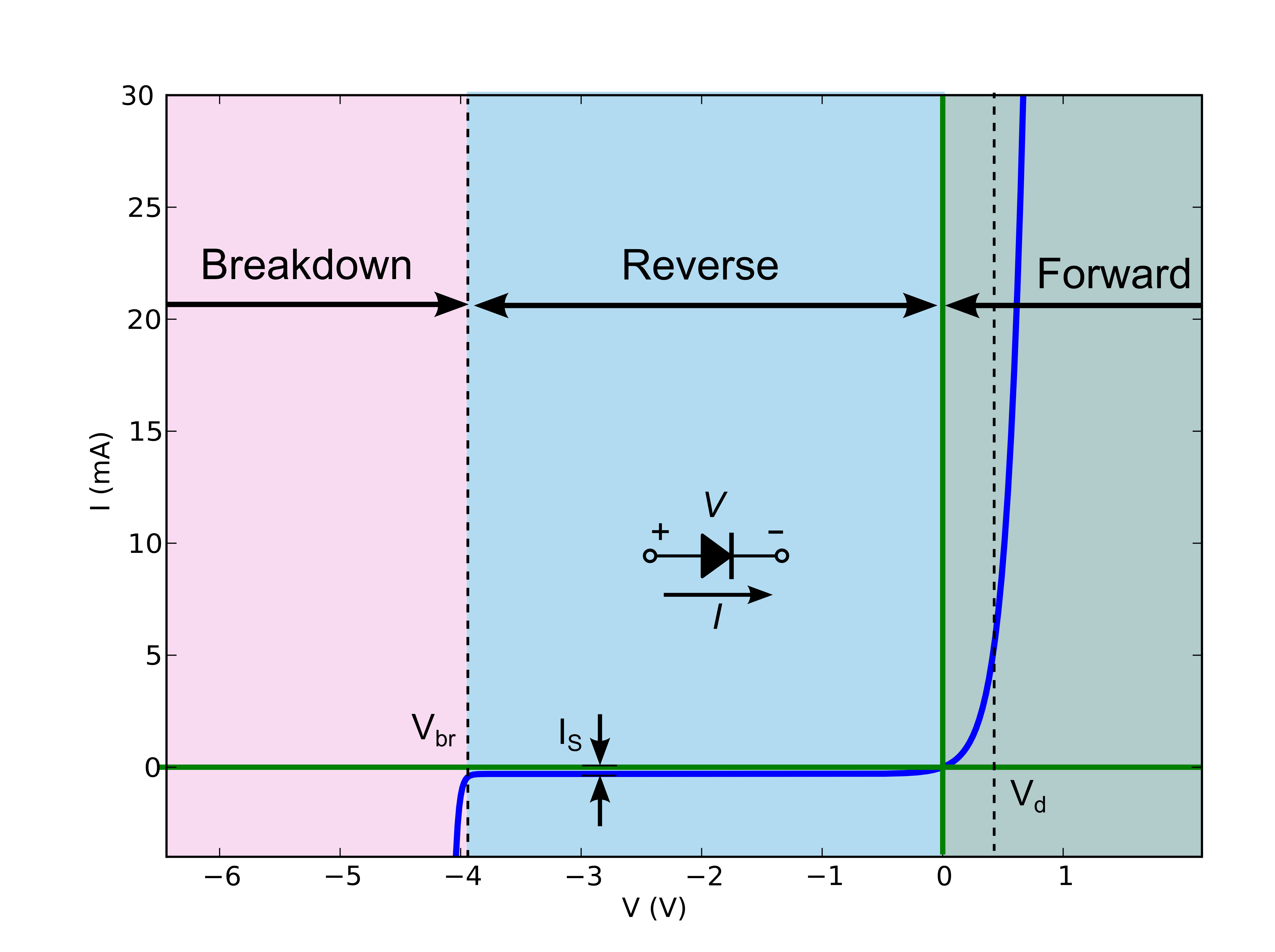
Here's such a graph with a resistor, an "ideal" diode according to the Schockley diode equation and no resistance, and a more realistic model of an LED that includes some resistance:

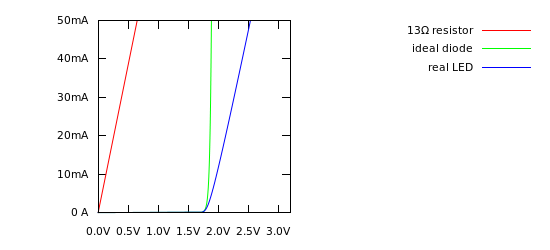
You can see that for values of current > 5 mA, the ideal diode looks like a vertical line. It's actually just very steep, but at this scale, looks vertical. But real LEDs don't do this, not even close. If you look at the slope of the line in the datasheet above, it looks like a straight line through (1.8 V, 5 mA) to (2.4 V, 50 mA). The slope of that line is:

$$ \frac {2.4\:\mathrm V - 1.8\:\mathrm V} {50\:\mathrm{mA}-5\:\mathrm{mA}} = \frac{0.6\:\mathrm V}{45\:\mathrm{mA}} = 13\:\Omega $$

Thus, the internal resistance of the LED is 13 Ω.

Of course, you must also include the forward voltage drop of the LED in your calculations, which is responsible for the shift to the right between the resistor and the real LED lines. But, others have already done a good job of explaining that.

At the end of the day, you only need to model those aspects of an LED that are significant to your application. 13 Ω of resistance isn't significant if you are going to add another 1000 Ω. The knee in the current-voltage curve isn't significant if the LED will only be on or off. But, in the interest of understanding what simplifying assumptions you are making, and when those simplifying assumptions are no longer valid, I wanted to explain: an LED does have resistance.

Answer 3

Diodes, in general, do not have resistance (besides the small amount from the conductors inside the package), they do, however, have a voltage drop across them, the amount of which depends on the semiconductor material used in its construction. For typical LED's this voltage drop is ~1.5V . The voltage drop is related to the band-gap in the semiconductor (the energy difference between the highest bound electron state and the "conduction band"). This voltage drop does depend slightly on temperature and on current, but not significantly for a simple LED application.

TO illustrate, here is the I-V curve for a typical diode, note that the current asymptotically increases after a certain threshold voltage is reached. Note that unlike a resistor, the IV curve is very non-linear.

If you connect the diode directly to your battery without a resistor, the current in the diode is determined only by the (very small) resistance in the wiring and the internal resistance of the battery, thus the current in the diode will be huge and it will (most likely) burn up, because the diode by itself offers no resistance but conducts current.

To answer your question, in order to calculate the current flowing through the diode you need to determine the supply voltage, subtract the diode voltage drop, and use this new lower voltage to calculate the current using your limiting resistor.

Answer 4

The LED has a built in voltage drop(Due to the nature of a LED). You can look at the spec sheet of the LED you bought to determine the drop. The color of the LED usually affects the voltage drop across it.

For a more detailed explanation:

https://en.wikipedia.org/wiki/LED_circuit

Answer 5

The notion of "resistance" has a couple of related meanings. The simplest meaning is simply to say that at any moment in time, the resistance of a path between two points is defined as the ratio of the voltage between those points and the amount of current flowing through that path. The reason such a definition was regarded as a "law" is that for paths constructed of many materials--especially those that were known when Geor Ohm coined the definition--the ratio of voltage to current will remain roughly constant as the voltage and current vary. Thus, if one knows how much current flows through a path at some voltage, one can use the derived quantity "resistance" to predict how much current would flow at other voltages, or how much voltage would be needed to cause some other amount of current to flow.

For paths made of many materials, Ohm's Law works well to describe the relationship between voltage and current, but for paths made of some others the ratio of voltage to current isn't constant. Even with many of those, however, if one were to plot a graph of voltage versus current, there would be regions of the graph where the line is reasonably straight. When voltage and current would be on such a region, it may be helpful to model the device as an ideal voltage source in series with an ideal resistor. A hypothetical LED might behave as an almost infinite resistance when the voltage across it is in the range +0 to +1.5 (meaning almost no current would flow), but as a 100 ohm resistor in series with a 1.7-volt current source when the voltage is greater than 2.0 volts. Plotting those behaviors on a graph would yield two disjoint line segments with a gap between them. The LED's behavior would be some curve that connects those two lines, though Ohm's Law wouldn't really be suitable for trying to predict what that curve would be in that region.