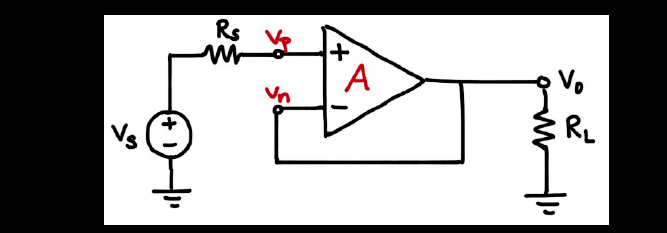
when Vs\$V_s\$ increases by delta_v\$\Delta V\$, Vn\$V_n\$ approaches Vp\$V_p\$ until the difference is delta_v / A\$\Delta V/ A\$ (where A is infinity)(where A is infinity).
Why is there the delta_v / A\$\Delta V/ A\$ difference and where does this difference come from? Why does it equal the specific value delta_v / A? Why doesn't V_n\$V_n\$ just equal V_p\$V_p\$ (so that the difference is 0)?