To generate a square wave, a free running multivibrator such as this will work:
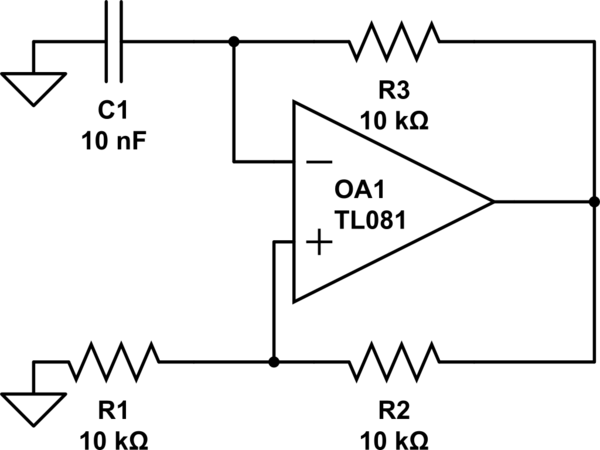
simulate this circuit – Schematic created using CircuitLab
The frequency of oscillation is
$$f_o=\frac{1}{2R_3C\ln{(1+2\frac{R1}{R2}})}$$
With the values on the diagram that would mean an \$f_o=4.56\$ KHZ wit 50% duty cycle.
The opamp displayed is a TL081, which might not be the best to achieve a sharp square wave, using faster opamps will achieve faster rise/decay times, even the cheap LM301 will do the job.
The link to the question you provided includes an output filter, do you need a sine wave? If so, you might want to consider using something like a Wien Bridge Oscillator instead.
