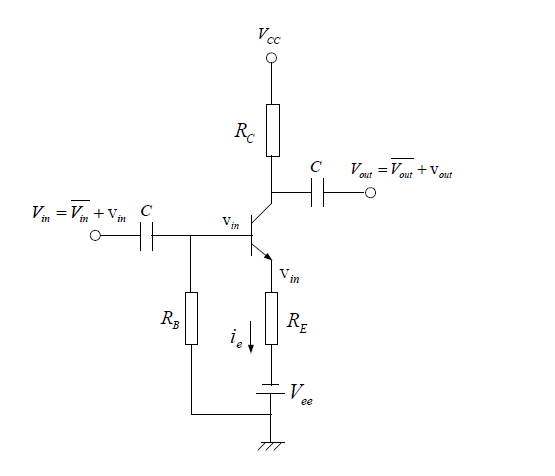
For an AC analysis we can draw the following circuit:
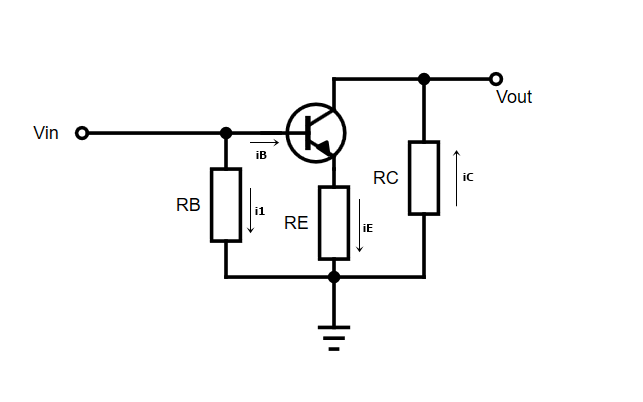
Now my job is to find \$v_{out}\$, which can be easily found by: $$ i_E=\frac{v_in}{R_E} \\ i_C=-\frac{v_{out}}{R_C} \\ i_E=i_C \\ v_{out}=-\frac{R_C}{R_E} v_in $$$$ i_E=\frac{v_{in}}{R_E} \\ i_C=-\frac{v_{out}}{R_C} \\ i_E=i_C \\ v_{out}=-\frac{R_C}{R_E} v_{in} $$
And this is reasonable, but one could also argue: $$ i_B=-i_1 \\ i_B=\frac{i_C}{\beta} \\ i_C=-\frac{v_{out}}{R_C} \\ i_1=\frac{v_{in}}{R_B} \\ v_{out}=\beta \frac{R_C}{R_B} v_{in} $$
The second one I think is wrong but I can't find the loophole in the reasoning.