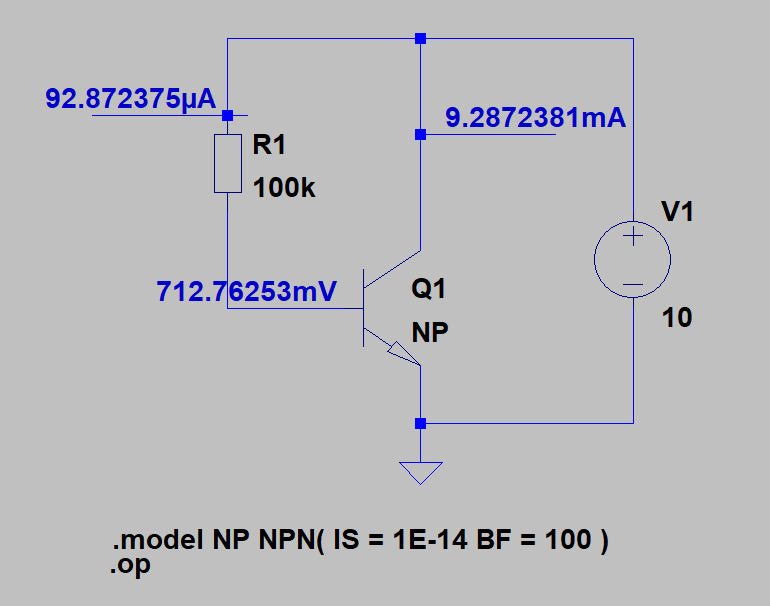
Let me examine this simple circuit
We know that for an ideal BJT the collector current will follow this equation:
$$I_C = I_S \times \left(e^{\frac{V_{BE}}{V_T}}-1 \right)$$
As you can see in this case we have \$I_S = 1E-14 = 10\text{fA}\$ and by default the ambient temperature is equal to \$ 27°C\$ thus \$V_T = 25.8649\text{mV}\$ and \$\beta = 100\$. And the emission coefficient/ideality factor \$NF = 1\$ by default.
And we can try to solve this circuit using the old method known as an iterative method.
First, we as usually assume some \$V_{BE}\$ value and solve for \$I_B\$ current.
$$I_B(1) = \frac{10V - 0.6V}{100k\Omega} = 94\mu A$$
Now I will use this equation to solve for the new \$V_{BE}\$ value.
$$ V_{BE} = V_T \ln \left(\frac{I_C}{I_S}+1\right)$$
But because you want to find the base current we must modify the equation to:
$$I_{SB} = \frac{I_S}{\beta} = 1E-16 = 0.1\text{fA}$$
$$ V_{BE} = V_T \ln \left(\frac{I_B}{I_{SB}}+1\right)$$
So, we finally can find the new \$V_{BE}\$
$$ V_{BE}(2) = V_T \ln \left(\frac{I_B}{I_{SB}}+1\right) = 0.7131V$$
Now I will use this new \$V_{BE}\$ value to find the new base current value.
$$ I_B(2) = \frac{10V - 0.7131V}{100k\Omega} = 92.869 \mu A$$
And we continue and find new \$V_{BE}\$ value and base current value.
$$ V_{BE}(3) = 25.8649\text{mV} \ln \left(\frac{ 92.869 \mu A}{0.1\text{fA}}+1\right) = 0.71276V$$
$$ I_B(3) = \frac{10V - 0.7128V}{100k\Omega} = 92.872 \mu A$$
$$ V_{BE}(4) = 25.8649\text{mV} \ln \left(\frac{ 92.872 \mu A}{0.1\text{fA}}+1\right) = 0.71276V$$
AS you can see because we are getting almost the same numbers we can conclude that.
\$V_{BE} = 0.7128V\$ and \$I_B = 92.872 \mu A\$ and \$I_C = \beta I_B = 9.2872mA\$
Of course, sometimes the equation does not converge, then we need to use for example the average value he average of the previous estimate and the calculated value.