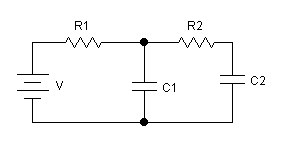
I'm trying to find the stored charge on capacitor1 at time t\$t\$
So, I think I have to find the voltage on the capacitor first. In terms of Current, the total current I(t)\$I(t)\$ should be the sum of the current on C1\$C_1\$ which is I1(t)\$I_1(t)\$ and the current on R2 (=dQ2\$R_2\$ (t)/dt\$=\mathrm{d}Q_2(t)/\mathrm{d}t\$).
I
I mean... I(t) = I1(t)+I2(t).
And $$ I(t) = I_1(t)+I_2(t). $$ And when we look at the outer loop, the total voltage V\$V\$ should be the sum of the voltage on R1\$R_1\$, R2\$R_2\$, and C2
So, V-R1I(t) - R2dQ2(t)/dt - Q2(t)/C2 = 0
Similarly\$C_2\$, so $$ V-R_1\cdot I(t) - R_2\cdot\frac{\mathrm{d}Q_2(t)}{\mathrm{d}t} - \frac{Q_2(t)}{C_2} = 0 $$ Similarly, at the inner loop, the total voltage V\$V\$ should be the sum of the voltage on R1 and C1
So, V - R1*I(t)\$R_1\$ and - Q1(t)/C1 = 0
But\$C_1\$, so $$ V - R_1\cdot I(t) - \frac{Q_1(t)}{C_1} = 0 $$ But here, I don't know what to do....
Plz help me :(
PS)PS
I didn't learn about the Thevenin theorem
when t=0\$t=0\$, there was no charge stored on C1\$C_1\$ and C2\$C_2\$