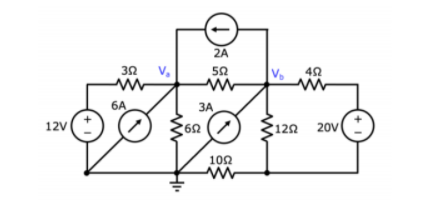
I have to find Va\$V_a\$ and Vb\$V_b\$, when iI tried to do so, using node analysis I had the Equation Va/6 + (Va-12)/3+(Va-Vb)/5 - 6 - 2=0\$\frac{V_a}{6} + \frac{V_a-12}{3}+\frac{V_a-V_b}{5} - 6 - 2 = 0\$ and Vb/22+(Vb-20)/4+(Vb-Va)/5 +2 -3=0\$\frac{V_b}{22}+\frac{V_b-20}{4}+\frac{V_b-V_a}{5} +2-3=0\$. Solving it simultaneously, iI got Va = 23.29V\$V_a = 23.29 \text{V}\$ and Vb=21.51V\$V_b=21.51 \text{V}\$. When I simulated it using Circuit simulator applet, the simulation says that Va = 24.4V\$V_a = 24.4 \text{V}\$ and Vb\$V_b\$ is 25.4V\$25.4 \text{V}\$. Can someone point out where did I go wrong? Thanks!