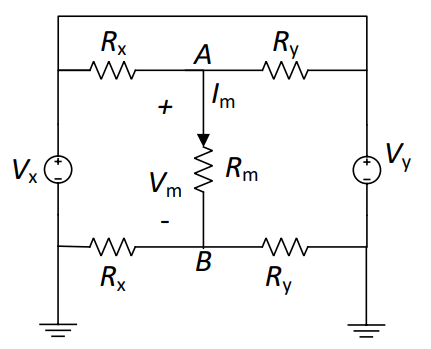
How to find Thevenin's Equivalent Resistance
Because of the two voltage sources, \$R_Z\$ is of no consequence and can be shorted out: -
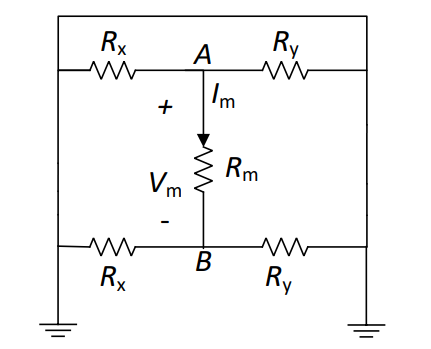
Then you can short all the voltage sources out and see that \$R_{TH}\$ is simply the resistance looking into node A plus the resistance looking into node B: -
Given that both those nodes have the same value of resistors associated with them, the Thevenin resistance is simply \$R_X||R_Y + R_X||R_Y = \dfrac{2\cdot R_X\cdot R_Y}{R_X+R_Y}\$.
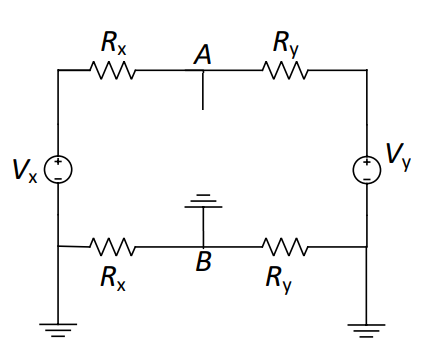
For the voltage, try seeing what I've done here: -
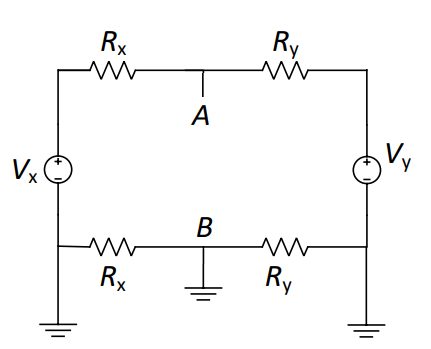
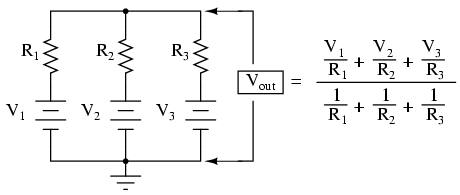
I'll leave the OP to solve this (easy to do using Millman's theorem): -
Image from here.