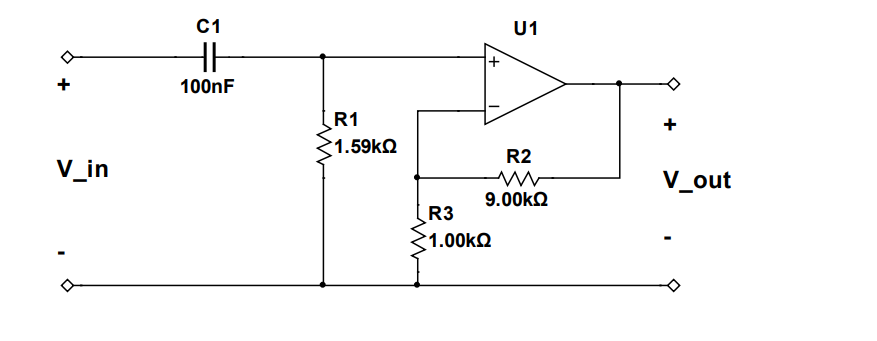
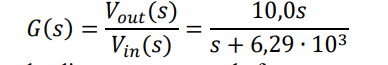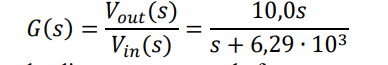I have this circuit:
I am asked to show that the transfer functions of the circuit is:
I know that for non-inverting omop-amps:
\$ Z_{in}=\infty \$\$ Z_{in}=\infty \$
\$ Z_{out}=0 \$\$ Z_{out}=0 \$
\$ H(s)=\frac{V_{out}(s)}{V_{in}(s)} = \frac{Z_{1}(s)+Z_{2}(s)}{Z_{1}(s)} = \frac{Z_{2}(s)}{Z_{1}(s)} +1 \$\$ H(s)=\frac{V_{out}(s)}{V_{in}(s)} = \frac{Z_{1}(s)+Z_{2}(s)}{Z_{1}(s)} = \frac{Z_{2}(s)}{Z_{1}(s)} +1 \$
But if I set:
\$ Z_1 = 1.00k\Omega \$\$ Z_1 = 1.00\mathrm{~k\Omega} \$
\$ Z_2 = 9.00k\Omega \$\$ Z_2 = 9.00\mathrm{~k\Omega} \$
I just get:
\$ H(s)=\frac{V_{out}(s)}{V_{in}(s)} = \frac{Z_{1}(s)+Z_{2}(s)}{Z_{1}(s)} = \frac{9.00k\Omega + 1.00k\Omega}{1.00k\Omega} = 10.00k\Omega \$\$ H(s)=\frac{V_{out}(s)}{V_{in}(s)} = \frac{Z_{1}(s)+Z_{2}(s)}{Z_{1}(s)} = \frac{9.00\mathrm{~k\Omega} + 1.00\mathrm{~k\Omega}}{1.00\mathrm{~k\Omega}} = 10.00\mathrm{~k\Omega} \$
which is not the same as:
What am I doing wrong here? thansk in advance :-)