Return to Question

Isn't the general expression for the resistance looking into the emitter
$$Re=\left(\frac{Rb}{1+β}+Rm\right)||\,(Ro+Rc)$$
So when the base is grounded with \$Rb\$ connected, we see:
$$Rm+\frac{Rb}{β+1}$$
and when it's open, we see
$$Ro+Rc$$ right?

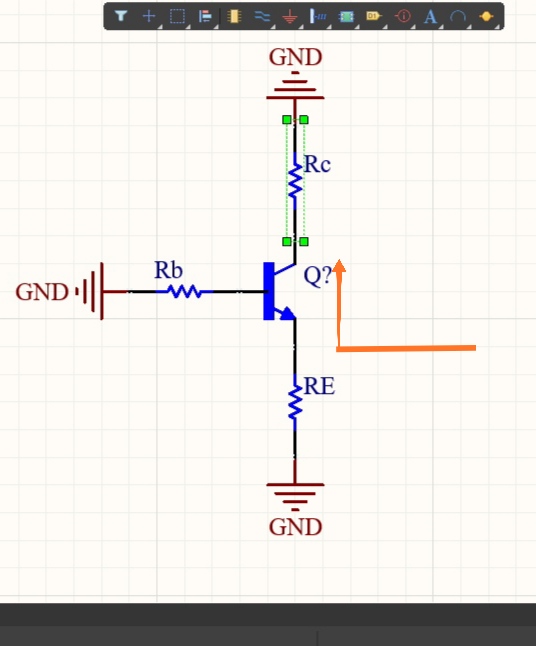
Isn't the general expression for the resistance looking into the emitter
$$Re=\left(\frac{Rb}{1+β}+Rm\right)||\,(Ro+Rc)$$
So when the base is grounded with \$Rb\$ connected, we see:
$$Rm+\frac{Rb}{β+1}$$
and when it's open, we see
$$Ro+Rc$$ right?

I Meant that when we have an emitter degeneration stage looking into the emitter of the transistor what would we see if the Rb resistor goes to infinity (which it's nearly same as saying what's the resistance we see when looking into the emitter of the common base stage i think) I just wanted the general expression for this because there's some cases that this may happen in it and we can't say it's just Rm = Rè = 1/gm (and that's all what we can find on internet)

Isn't the general expression for the resistance looking into the emitter is $$Re=\left(\frac{Rb}{1+β}+Rm\right)||\,(Ro + Rc).$$ So when the base is grounded with \$Rb\$ connected, we see:
$$Rm+\frac{Rb}{β+1}$$
and when it's open, we see \$Ro+Rc\$, right?

Isn't the general expression for the resistance looking into the emitter 
$$Re=\left(\frac{Rb}{1+β}+Rm\right)||\,(Ro+Rc)$$
So when the base is grounded with \$Rb\$ connected, we see:
$$Rm+\frac{Rb}{β+1}$$
and when it's open, we see
$$Ro+Rc$$ right?

Isn't the general expression for the resistance looking into the emitter is $$Re=\left(\frac{Rb}{1+β}+Rm\right)||\,(Ro + Rc).$$ So when the base is grounded with \$Rb\$ connected, we see:
$$Rm+\frac{Rb}{β+1}$$
and when it's open, we see \$Ro+Rc\$, right  ?

Isn't the general expression for the resistance looking into the emitter is $$Re=\left(\frac{Rb}{1+β}+Rm\right)||\,(Ro + Rc).$$ So when the base is grounded with \$Rb\$ connected, we see:
$$Rm+\frac{Rb}{β+1}$$
and when it's open, we see \$Ro+Rc\$, right?