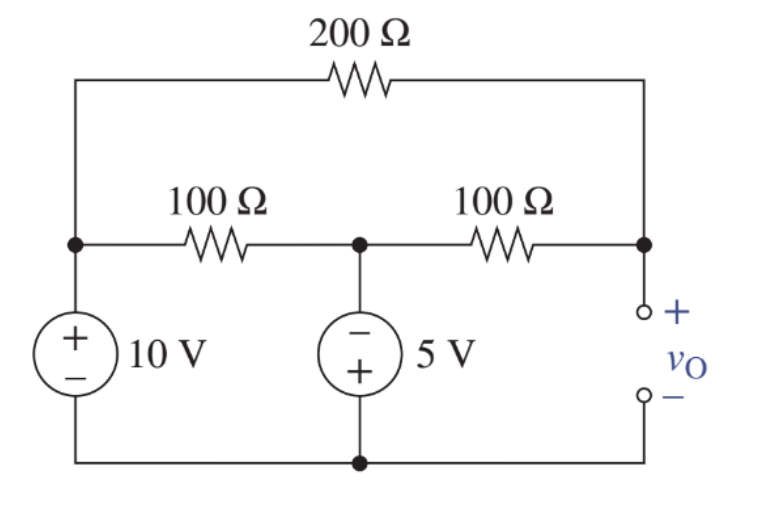
this is the circuit i'm trying to solve: please help! thanks
6
-
2\$\begingroup\$ Hint: one of the resistors can be removed without affecting the answer. \$\endgroup\$– The PhotonCommented Sep 16, 2017 at 22:20
-
\$\begingroup\$ can you remove both the 100 Ohm resistor on the right and the 200 Ohm resistor because there is no current running through them because of the open circuit? Therefore is the Vo the voltage across the 5V source? \$\endgroup\$– eestudent101Commented Sep 16, 2017 at 23:44
-
\$\begingroup\$ Sum the currents going into the Vo node. Make sure you notice that the 5V supply is kind of "upside down." A sneaky little trick. You should end up with two equations and one unknown. \$\endgroup\$– user57037Commented Sep 16, 2017 at 23:45
-
\$\begingroup\$ No, the 200 Ohm resistor may have current flowing into it. The one that does not effect the answer is the 100 Ohm closest to the 10V supply. The reason that it does not matter is because it is in between two nodes whose voltages are defined by voltage sources. There will be current flowing through it, but it does not effect Vo. Write the equations like I said. \$\endgroup\$– user57037Commented Sep 16, 2017 at 23:48
-
\$\begingroup\$ Redraw the schematic and you will see a Voltage divider from 10V to -5V \$\endgroup\$– sstobbeCommented Sep 17, 2017 at 0:49
|
Show 1 more comment
1 Answer
\$\begingroup\$
\$\endgroup\$
2
In the given circuit if we pick the lowest rail to be the ground and assign it a 0 potential then the potential values on the remaining nodes will be as shown.
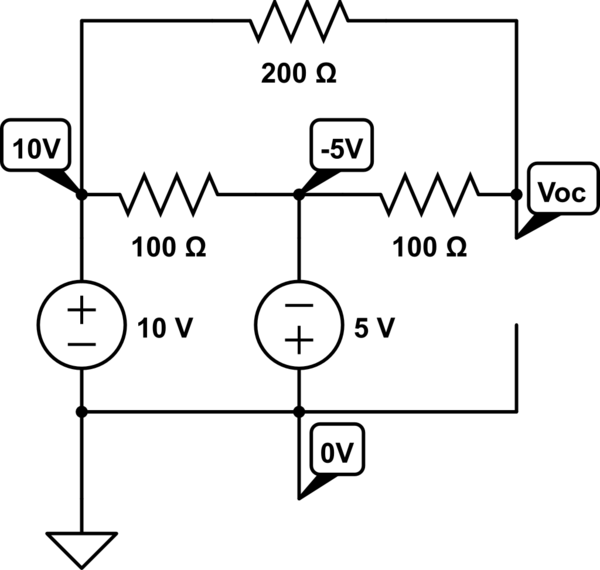
simulate this circuit – Schematic created using CircuitLab
For the 200 ohm resistor the current through it will be given by (10-Voc)/200 A and this should be equal to the current flowing out of the node that has voltage Voc ie. (Voc-(-5))/100. Equating the two currents we get Voc = 0V.
-
\$\begingroup\$ As a general rule, when someone asks for help with schoolwork, we try to give them hints, or get them "un-stuck", but not provide a complete solution. The idea is that they are supposed to do their work themselves, and also, that by struggling, they will be more likely to really learn the material. \$\endgroup\$ Commented Sep 21, 2017 at 4:16
-
\$\begingroup\$ @mkeith Ok I'll keep this in mind in future. Thanks for letting me know. \$\endgroup\$– ijunejaCommented Sep 21, 2017 at 5:07