Find the capacitance of this rectangular slab of two films of metal virtually 0 thickness and 2 sheets of plastic. Then it is rolled into a cylinder

Question

this question may be pretty simple for some people on this forum. Here's a basic image to go with the question.

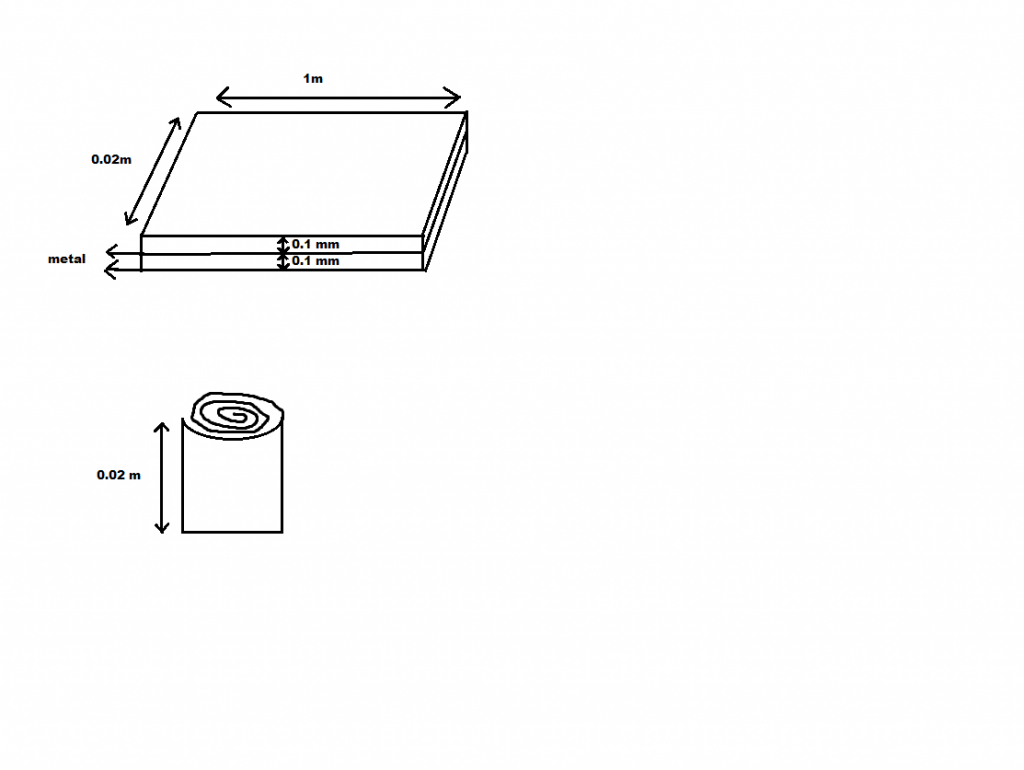(also here)

1. Two films of metal, virtually 0 thickness and 2 sheets of plastic. Find the capacitance.
2. The assembly is then rolled into a cylinder, estimate its radius. Why does the capacitance approximatly double?

Ok, first of all this is whats i've got so far. I've just used the Er of plastic as 2.

C=(A*E0*Er)/h so (1*0.02*(8.85*10^-12)*2)/10^-4 = 3.54*10^-9F

But would you multiply that by 2 since there are two plastic sheets and two metal parts? Or would you add them in capacitance of them both in series? Also if the metal sheets were thicker and had a realative permitivity how would you find the capacitance in this assembly? Could you multiply the Er of the metal with the Er of the plastic and E0. But what about the new thickness? Essentially this just two dielectrics correct?

now for 2. this where I'm even more confused. the volume of a cylinder \$ \pi r^2 d\$ we could get d. oh, i'm not sure to be honest.

Any help would be greatly appreciated.

Edit. Hang on, there is just one dielectric, the plastic and the metal is just used as a conductor between them. Sorry. Would be interesting to know how to calculate two dielectrics in the capacitor though. I'm thinking you would just need to know whether they were in series or parallel.

Answer 1

\$\epsilon_r\$ of 2 is kind of small for plastics, a lot are more like 3 or 4, but that's not too important.

As to why it (sort-of) doubles- think about the physical arrangement. The second sheet of plastic (the one that is not between the metal sheets) is doing nothing before you roll it up. When you roll it up, into (say) n turns, you will be using both sides of each sheet of metal, except for the last turn inside and the first turn outside. So, if n is big enough, it approximately doubles (if the roll starts at zero radius, the inside turn won't matter).

You should be able to estimate the radius easily from the standard mensuration formulas for solids. The rectangular prism will have volume of \$V_p = 2t\cdot L\cdot W\$ where t is the thickness of a single sheet of plastic. The cylinder will have a volume of \$V_c = \pi r^2 \cdot L_C\$ where \$L_C\$ is the length of the cylinder. So, depending on how you roll (so to speak), \$L_C\$ will be either L or W from the sheet and by equating the volumes, you can solve for r (which assumes that the inner radius is zero).