Hardware Implementation of Discrete time Systems

Question

Discrete systems work on sampled signal values. Suppose if we want to implement such a system like a discrete amplifier (i.e., \$y[n] = A\cdot x[n]\$), how do we make sure that the input to this system is available only at discrete time intervals? Even if we use sample and hold circuits, the data on the sampling capacitor may change during the sampling time interval.How do we ensure that data input to the system doesn't change between successive sampling times?

Answer 1

Many MCU implement the acquisition of the signal with a dual switch system.

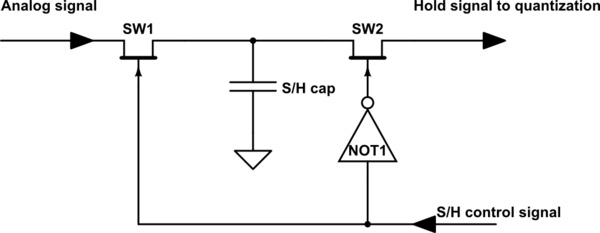
^{simulate this circuit – Schematic created using CircuitLab}

That is, the two switching elements are operated with a single signal, so as to have opposite conduction states.
While a state applies the signal to the capacitor, when the corresponding signal is activated, it stops applying the signal to the capacitor, and the voltage across the capacitor is available to be quantized.
The time during which the switch SW1 is closed, applying to capacitor signal is called the sample time or time of opening (\$t_s\$). The time during which the switch SW1 is open and SW2 is closed, is the time of quantization (\$t_q\$). Usually \$t_q\gg t_s\$