I'm using KCL to analyse the circuit that has a voltage source between two non-reference nodes connected in parallel with resistor R3.
I'm regarding the voltage source and the resistor as a supernode. To solve the circuit I need the constraint equation that can be obtained by applying KVL to the supernode.
Why isn't the resistor R3 important in this case, when connected across a supernode?
simulate this circuit – Schematic created using CircuitLab
This is so because the voltage source is ideal, and therefore, deliver a constant voltage, regardless of current requested.
The effect of adding a resistor in parallel to the voltage source, is to ask more current, which does not affect the source voltage.
For example, consider this simple circuit:
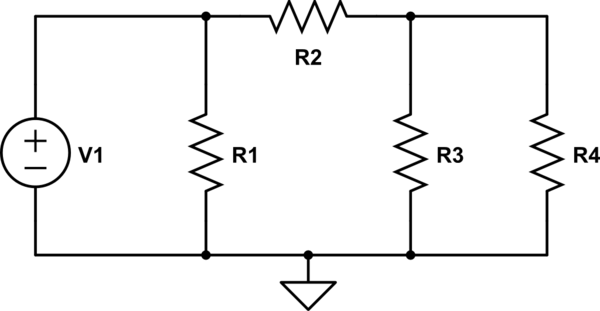
simulate this circuit – Schematic created using CircuitLab
Does it depend on the current through \$R_4\$, the value of \$R_1\$? Of course not. Moreover, one can remove the resistor \$R_1\$ and the current circuit by \$R_4\$ be the same. \$R_1\$ does affect the amount of current that requests the V1 source, but as this source is ideal, the voltage applied to the set \$R_2\$, \$R_3\$ and \$R_4\$ is unchanged.
Another way to look at it is considering that the voltage source is an element that imposes the voltage between two nodes, regardless of other elements (passive element) are connected between the two nodes. That's why, for example, you can not connect two voltage sources in parallel, since a singularity is generated.
