Need help finding the drain current of a jfet

Question

I have this simple dc jfet circuit (homework):

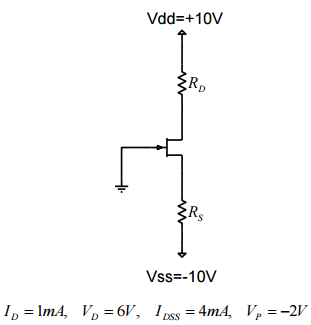
First of all I had to find the values of the drain and source resistors given the data above, this was pretty simple and I got: $$R_D=4k\Omega $$ $$R_S=11k\Omega $$

Next, \$I_{DSS}\$ get's doubled to \$8mA\$, now I'm asked to find \$I_D\$.

Using: $$I_D = I_{DSS}\left [ 1-\frac{V_{GS}}{V_P} \right ]^{2} $$

I've found out that: $$I_{D1} =1.16mA$$ $$I_{D2} =1.0258mA$$

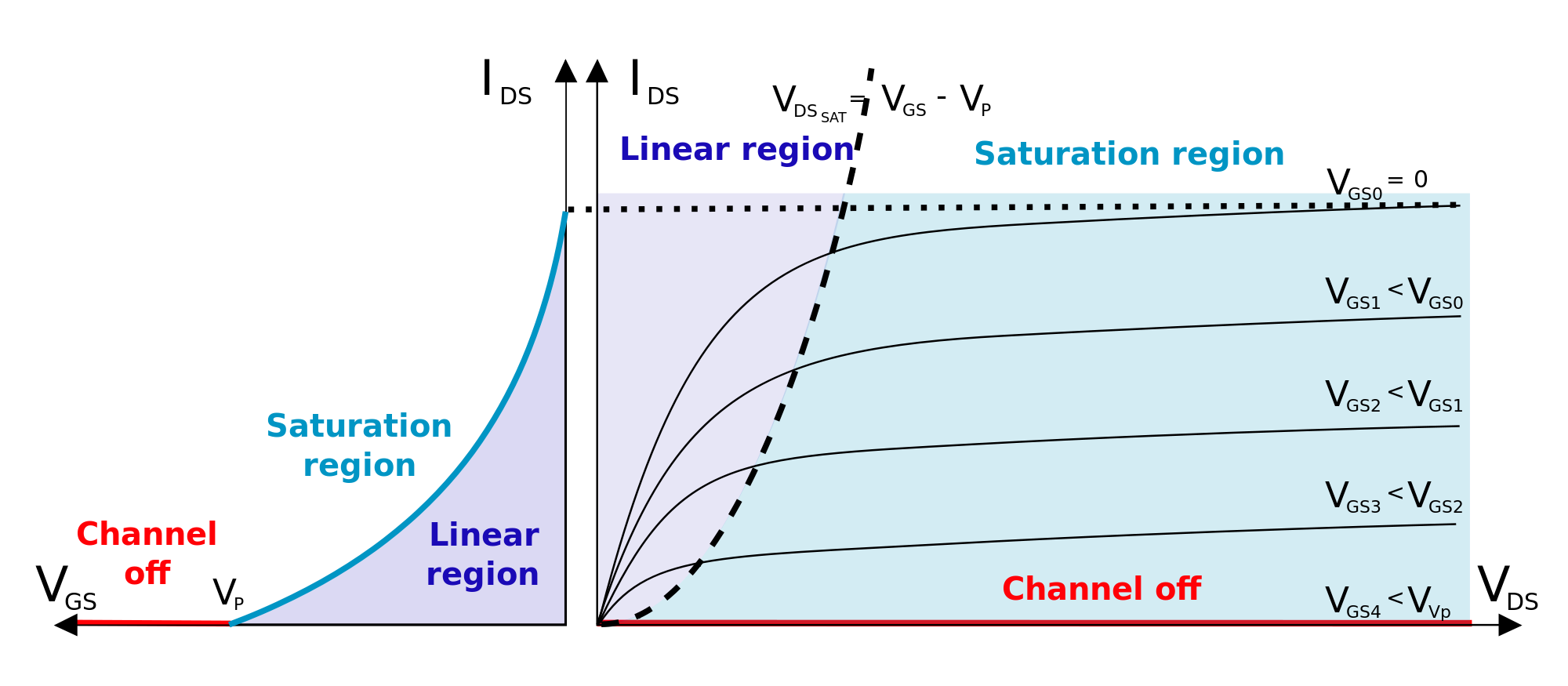
During the calculations I've assumed the transistor is saturated thus: $$V_{DG} > V_P $$ It seems like both \$I_D\$'s fulfill the above requirement.

How can I tell which one is correct ?

Answer 1

The image (taken from wikipedia) below shows the JFET chara. It can be seen that the drain current reduces to zero as \$V_{GS}\$ approaches pinch off (\$V_{P}\$). And the channel is off for \$|V_{GS}|>|V_{P}|\$ and no current flow happens.

So the transistor is in saturation and the Shockley's equation

$$I_D = I_{DSS}\left [ 1-\frac{V_{GS}}{V_P} \right ]^{2} $$

is valid only if \$|V_{GS}| < |V_P|\$ and \$V_{DG} > V_P\$.

---

Now calculating \$V_{GS}\$ in your case,

case1: \$I_{D1} =1.16mA\$ $$V_{GS} = -2.76V$$ But \$V_{P}=-2V\$ so \$|V_{GS}| > |V_P|\$ and hence transistor is in cut-off.

case2: \$I_{D2} =1.0258mA\$ $$V_{GS} = -1.2838V$$ Here, \$|V_{GS}| < |V_P|\$ and hence transistor is in saturation.

So \$I_{D} =1.0258mA\$ is the correct answer.

---

PS: You should have faced this problem while calculating the value of \$R_S\$ also.

$$1mA = 4mA\left( 1 + \dfrac{10-1mA\times R_S}{2}\right)^2$$

\$R_S = 11k\Omega\$ and \$R_S = 13k\Omega\$ will satisfy this equation. The value \$R_S = 13k\Omega\$ can not be used because of the same reason discussed above.