Consider a circuit that only has a constant current source and a capacitor. The voltage across a capacitor is proportional to the integral of the current I, times time. Since the current is constant it may be taken outside the integral. Based on this, the voltage across the capacitor will continue to increase as time goes by. Now let's say I turn the source on for X seconds and then turn it off. Would the time constant for the charging phase, and the time constant for the discharging phase be the same? I'm not sure if the input resistance of the source has any effect on the time constant. Thank you for any advice!
\$\begingroup\$
\$\endgroup\$
1
-
\$\begingroup\$ By "Turn off" do you mean shorting or disconnecting? (zero current or infinite). In either case the time will be infinite (or equal X sec) or zero. There is no meaning of time constant in these circumstances. \$\endgroup\$– Eugene Sh.Commented Jul 13, 2015 at 18:20
Add a comment
|
1 Answer
\$\begingroup\$
\$\endgroup\$
Here's the circuit I think you're describing:
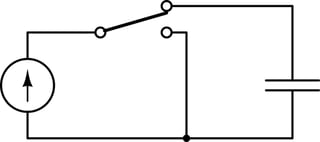
simulate this circuit – Schematic created using CircuitLab
There's no time constant in this situation. The current source makes the capacitor voltage rise linearly. Time constants only appear when there's exponential decay.
When the current source is disconnected from the capacitor, there's no way for the capacitor to discharge. You can't just connect both ends to ground since the voltage across a capacitor is not allowed to change instantaneously in circuit theory.
Sources don't have input resistance since they have no inputs. They do have output resistance. If you include an output resistance with the current source, that will get you an exponential decay with a time constant, and the resistance will affect the time constant.
In general, it is possible to have different time constants for charging and discharging if you switch other components in or out of the circuit.
answered Jul 13, 2015 at 18:29