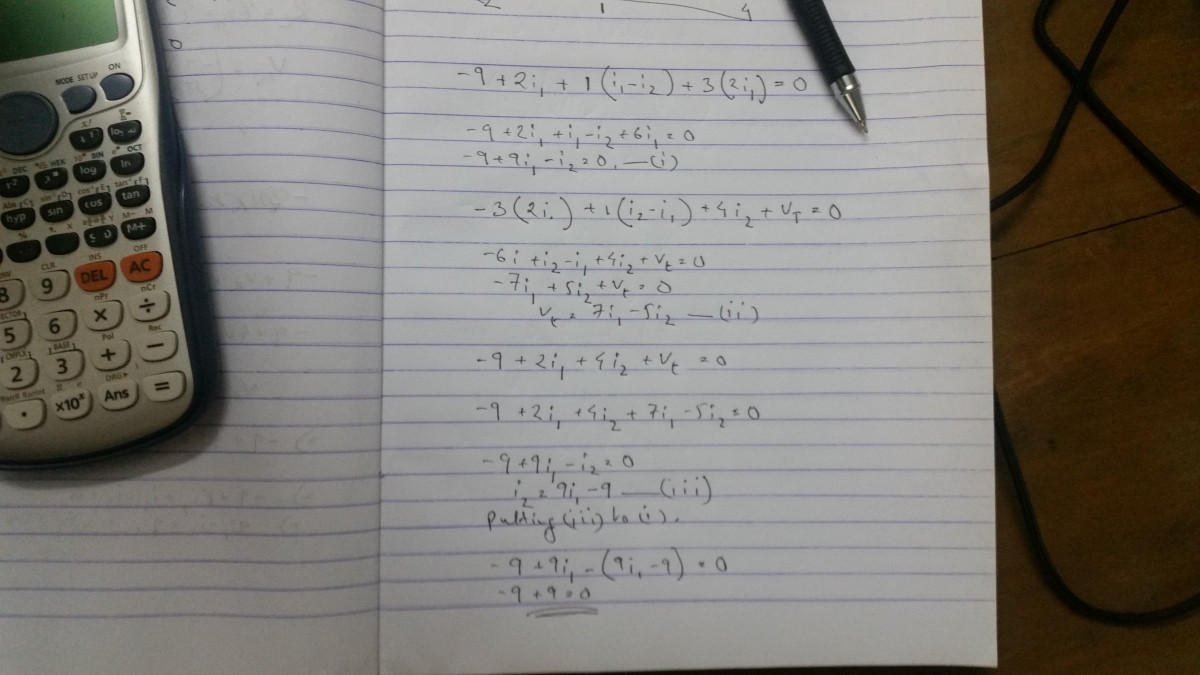To my understanding the thevenin voltage is the potential difference between a fixed circuit and the ends in which we can add a load. I am going in circles with this problem. I was able to find the thevenin resistance, but it's the thevenin voltage I am having problems with. I applied KVL to the circuit thrice (inner 2 loops and the outer loop) and I get equations that cancel each other out. Can anyone do a quick help on this problem of mine?
2 Answers
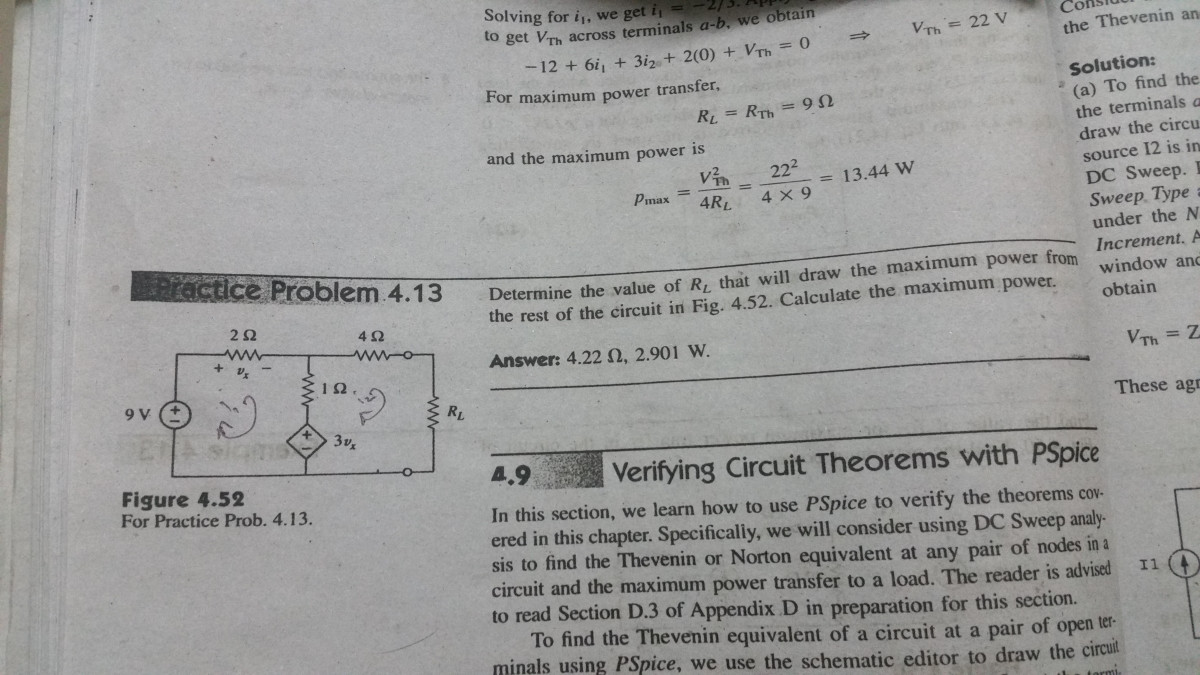
You have to find the Thevenin equivalent circuit (TEC) of the circuit without the load, i.e. the part of the circuit left of the two terminals (marked as two empty circles) the load is connected to.
In order to find the TEC of that circuit find e.g. by nodal (or mesh) analysis
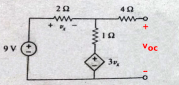
- the open circuit voltage \$v_{oc}\$ (i.e voltage between the unconnected terminals)
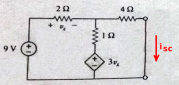
and - the short circuit current \$i_{sc}\$ (i.e. current through a short between the terminals)
of the circuit.
Then the TEC is a voltage source of \$v_{th} = v_{oc}\$ with series resistance \$R_{th} = \frac{v_{oc}}{i_{sc}}\$:
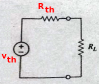
You probably know that maximum power transfer is happening if \$R_L = R_{th}\$.
Therefore the voltage across \$R_L\$ will be \$\frac{1}{2}v_{th}\$ so the power dissipated in \$R_L\$ is \$P = \frac{(\frac{1}{2}v_{th})^2}{R_L}\$.
The Thevenin equivalent voltage is the open-circuit output voltage of the circuit. So you can calculate it by finding the output voltage when \$R_L\$ goes to infinity.
This immediately gives you \$i_2=0\$.
Now you only need to write a single KVL equation to get \$i_1\$, and from there calculate the voltage at the output terminals.