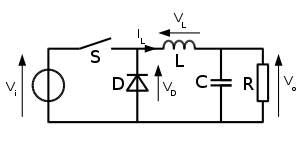
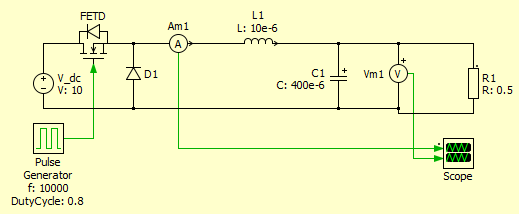
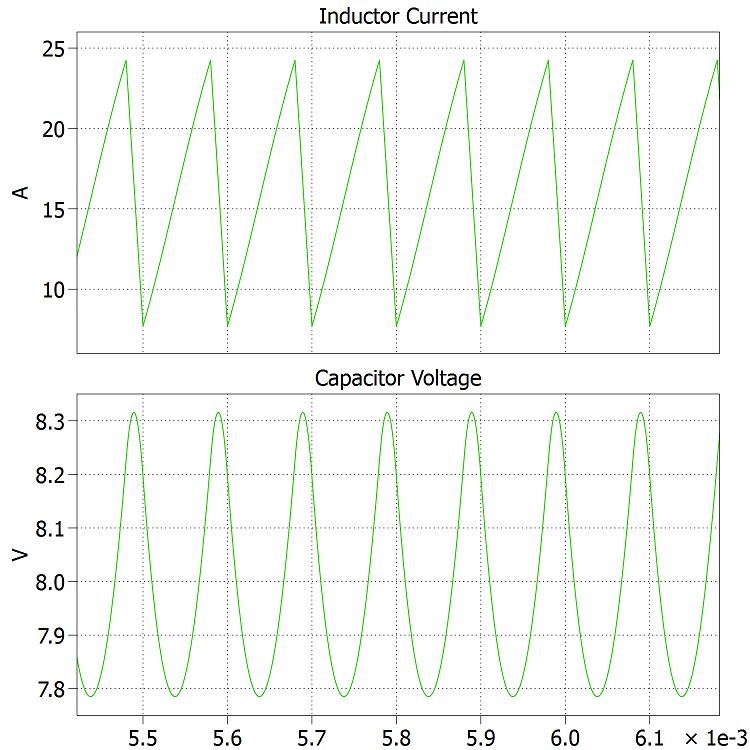
When DC voltage is applied across an inductor, would the current through it be ramp? According to voltage current relationship in an inductor, the current should be ramp if voltage across inductor is constant.
- Is this practically possible only when there is no resistance connected in series with inductor?
- Why would the current through inductor be ramp in this circuit of dc-dc buck converter?
Edit
The large value of capacitance reduces the change in output voltage and so the voltage across inductor remains constant. This would mean that load current and hence output voltage is not really constant. Why is this acceptable?
What would be the nature of voltage across the capacitor(output voltage?