1
\$\begingroup\$

This is a hybrid-pi model of a BJT, and I want to know its output impedance:

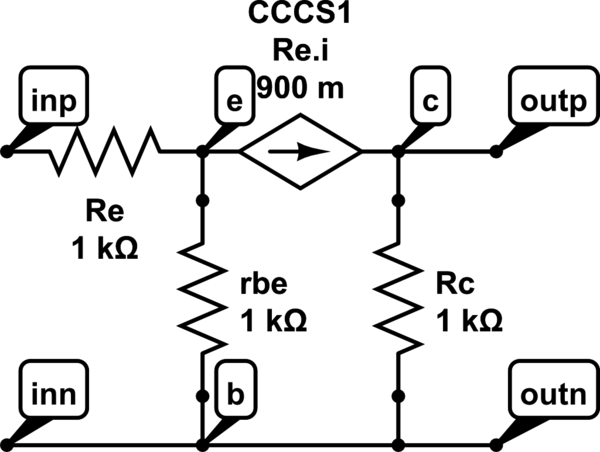
schematic

simulate this circuit – Schematic created using CircuitLab

I know that I should short the input ports, place a voltage on the output ports, calculate the consequent current and finally apply Ohm's law, but I have no idea how to deal with that CCCS.

According to the key, the input impedance is Rc. Does this implies that the CCCS can be seen as a ideal current source, and thus considered as open circuit?

\$\endgroup\$
14
  • \$\begingroup\$ You mention "input impedance" yet I see no input. Be clear about the nets between which you want to determine the impedance. For example mark those nets inp and inn in the schematic and say that you want to determine the impedance between those points. \$\endgroup\$
    – Bimpelrekkie
    Commented May 10, 2016 at 12:12
  • \$\begingroup\$ @FakeMoustache Edited now \$\endgroup\$
    – nalzok
    Commented May 10, 2016 at 12:14
  • \$\begingroup\$ Impedance is the ratio of dV/dI where dV is a small voltage change and dI is the resulting current change. Consider the change in the current coming out of a constant current source when the voltage is changed. But cccs1 is not a constant current source. Or is it ? What determines it's current ? The current through... which is the same current as .... hint: assume that current has a certain value, will it change if the voltage between inp and inn changes ? \$\endgroup\$
    – Bimpelrekkie
    Commented May 10, 2016 at 12:15
  • \$\begingroup\$ @FakeMoustache But shouldn't constant current sources be seen as open circuit when calculating input impedance? \$\endgroup\$
    – nalzok
    Commented May 10, 2016 at 12:17
  • \$\begingroup\$ Yes but cccs1 is a controlled current source. So only if it's current is actually constant and not depending on the voltage between inp and inn can you assume it to be an open when determining the impedance between inp and inn. \$\endgroup\$
    – Bimpelrekkie
    Commented May 10, 2016 at 12:20

1 Answer 1

Reset to default
2
\$\begingroup\$

This circuit model is a logical impossibility.

The control current of the CCCS is the same as its output current. If the gain of the CCCS were anything other than 1, it would be obvious why this is a problem: a current can't be \$Z\$ times itself for any value of \$Z\$ other than 1. (except of course if the current happens to be 0).

Setting the gain to 1 makes this logical contradiction seem to disappear, but the circuit model is still a poor one --- in any real circuit there'd be uncertainty about what that gain is exactly.

So, there's no more sense to trying to reason about this circuit than there is to contemplating the equation

$$3 = 2\times{}5$$

Edit

The question has been clarified to indicate this is meant to be a simplification of the hybrid pi model of a BJT. Here's the usual hybrid pi model: enter image description here (source: Wikimedia)

The issue with your model is resolved by including the \$r_o\$ element.

\$\endgroup\$
4
  • \$\begingroup\$ In fact, this is a "simplified simplified" hybrid-pi model of a BJT and I was trying to calculate Ro. As I've said, this post is an XY problem: meta.stackexchange.com/a/66378. Sorry for the confusion caused. \$\endgroup\$
    – nalzok
    Commented May 10, 2016 at 16:24
  • \$\begingroup\$ @sunqingyao, then it's over-simplified. For one thing, a BJT has three terminals, not two. \$\endgroup\$
    – The Photon
    Commented May 10, 2016 at 16:26
  • \$\begingroup\$ Exactly. Question has been edited to address my original intention, if this matter. Anyway, I've understood the reason now. \$\endgroup\$
    – nalzok
    Commented May 10, 2016 at 16:37
  • \$\begingroup\$ @sunqingyao, To make the model make sense you need to include the \$r_o\$ resistance between collector and emitter. This will resolve the logical impossibility of your model. \$\endgroup\$
    – The Photon
    Commented May 10, 2016 at 17:00

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.