1
\$\begingroup\$

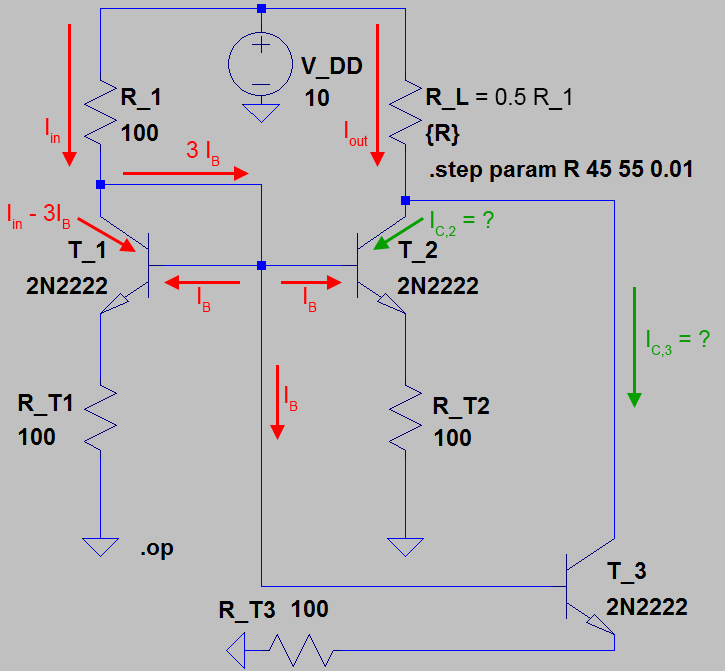
Identification: Is there a name for the following NPN BJT Current Source with three identical BJTs, which doubles the reference input current?

NPN BJT Current Source Current Doubler

Analysis: If \$R_L = \frac{1}{2} R_1\$ as shown in the schematic, then the following is valid: $$I_{out} = 2 ( I_{in} - 3 I_B ) = 2 \frac{\beta}{\beta + 3} I_{in} \approx 2 I_{in} \ \text{for} \ \beta \gg 1$$

  • Can one easily see that \$I_{out} = 2 ( I_{in} - 3 I_B )\$ is true?
  • What are the values for \$I_{C,2}\$ and \$I_{C,3}\$ based on the above assumptions?

Are the following answers correct?

  • Due to the identical BJTs and identical base currents, \$I_{C,2}\$ = \$I_{C,3}\$
  • Hence, \$I_{out} = I_{C,2} + I_{C,3} = 2 I_{C,2} = 2 I_{C,3}\$
  • Because of the mirroring characteristics, \$I_{C,1} = I_{C,2} = I_{C,3} = I_{in} - 3 I_B\$
  • Result: \$I_{out} = 2 ( I_{in} - 3 I_B ) = 2 I_C\$
  • Further transformations: $$ I_B = \frac{1}{\beta} I_C \rightarrow 2 I_C = 2 ( I_{in} - 3 I_B ) = 2 ( I_{in} - \frac{3}{\beta} I_C )$$ $$ I_C = I_{in} - \frac{3}{\beta} I_C \rightarrow I_C (1 + \frac{3}{\beta} ) = I_{in} \rightarrow I_C ( \frac{3 + \beta}{\beta} ) = I_{in} $$ $$ \hookrightarrow I_{out} = 2 I_C = \frac{2 \beta}{\beta + 3} I_{in} $$
\$\endgroup\$
4
  • \$\begingroup\$ Are you sure you don't want a resistor connected to the base of T3? \$\endgroup\$
    – The Photon
    Commented Jul 17, 2016 at 2:36
  • \$\begingroup\$ Also, why not just reduce RT2 to ~50 ohms instead of introducing the extra transistor? \$\endgroup\$
    – The Photon
    Commented Jul 17, 2016 at 2:37
  • \$\begingroup\$ @ThePhoton Yes, there was the resistor R_T3 missing, thank you. The idea of adding an extra resistor in parallel to multiply the current is introduced in a book and I try to understand the idea behind it. \$\endgroup\$
    – Discbrake
    Commented Jul 17, 2016 at 2:52
  • 1
    \$\begingroup\$ I didn't read through your text. This circuit is just a current mirror. A triple current mirror, if you like. In CMOS IC design, the current ratio between the reference current and the output current can be controlled in other ways, rather than just adding additional transistors. If you look at schematics for op-amps (in datasheets) they often have multiple output current mirrors for different stages of the amplifier. \$\endgroup\$
    – user57037
    Commented Jul 17, 2016 at 16:02

1 Answer 1

Reset to default
1
\$\begingroup\$

What you have is a multiple output current mirror with the outputs connected in parallel. If you want a short name, you could call it a current doubler or a current multiplier.

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.