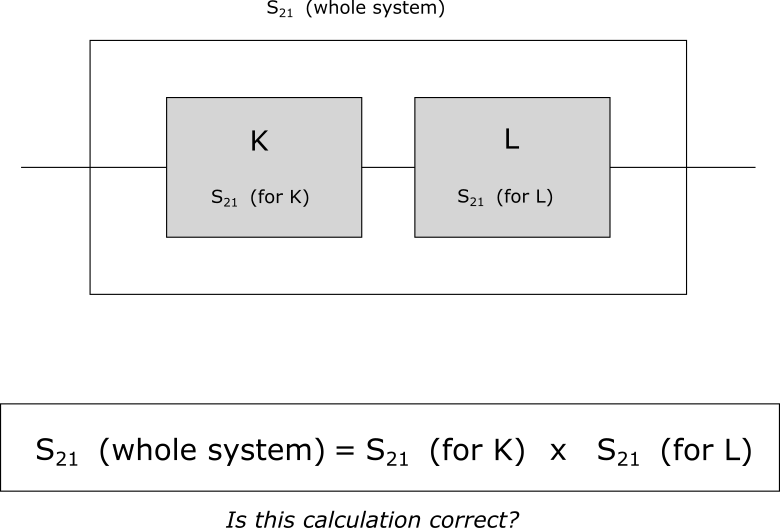
I have a two port system which consists of two subsystems. Is the multiplication of S21 parameters of the subsystems equal to that of the whole system?
3
-
\$\begingroup\$ Only if \$S_{11}(L)\$ and/or \$S_{22}(K)\$ are 0. \$\endgroup\$– The PhotonCommented Aug 4, 2016 at 23:20
-
\$\begingroup\$ Does it mean there must be no input reflection for L and no output reflection for K? \$\endgroup\$– adbaCommented Aug 4, 2016 at 23:32
-
\$\begingroup\$ If one or the other is 0, I think it's enough. \$\endgroup\$– The PhotonCommented Aug 5, 2016 at 0:33
Add a comment
|
1 Answer
\$\begingroup\$
\$\endgroup\$
1
It isn't that simple. There can also be an effect due to reflections back and forth between the input of L and the output of K.
If L has no input reflections (\$S_{11}=0\$) or K has no reverse reflections (\$S_{22}=0\$), then your formula should work. (Edit: As I think about it some more, you'd also need to have a perfectly matched load on the output of L)
But if that's not the case, you have to jump through more hoops. The usual approach is to transform the model to a different representation called "T parameters":
$$T_{11}=\frac{1}{S_{21}}$$ $$T_{12}=-\frac{S_{22}}{S_{21}}$$ $$T_{21}=\frac{S_{11}}{S_{21}}$$ $$T_{22}=\frac{-(S_{11}S_{22}-S_{12}S_{21})}{S_{21}}$$
The T parameters can then be cascaded:
$$T^{(KL)}=T^{(L)} T^{(K)}$$
And then the T parameters can be transformed back to S parameters:
$$S_{11}=\frac{T_{21}}{T_{11}}$$ $$S_{12}=\frac{(T_{11}T_{22}-T_{12}T_{21})}{T_{11}}$$ $$S_{21}=\frac{1}{T_{11}}$$ $$S_{22}=-\frac{T_{12}}{T_{11}}$$
answered Aug 5, 2016 at 0:48
-
\$\begingroup\$ ABCD parameters also (same as T, but scaled by Zo) ;-) \$\endgroup\$ Commented Aug 5, 2016 at 13:01