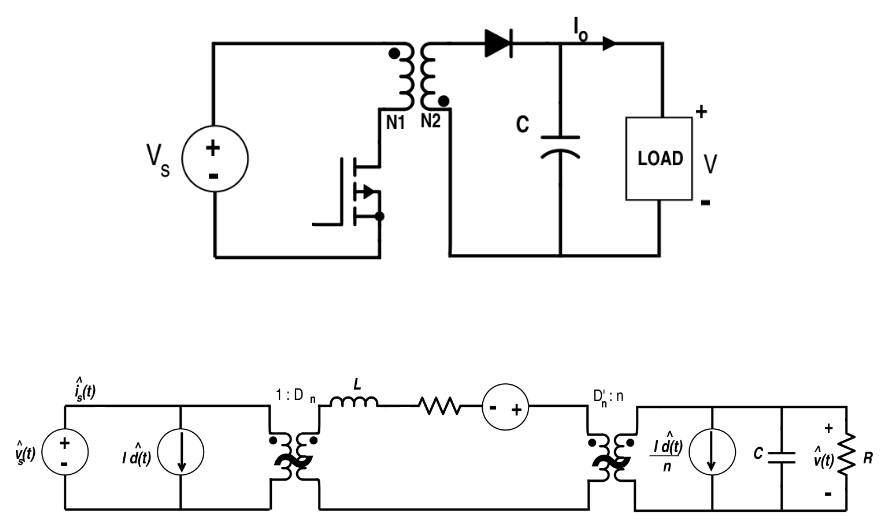
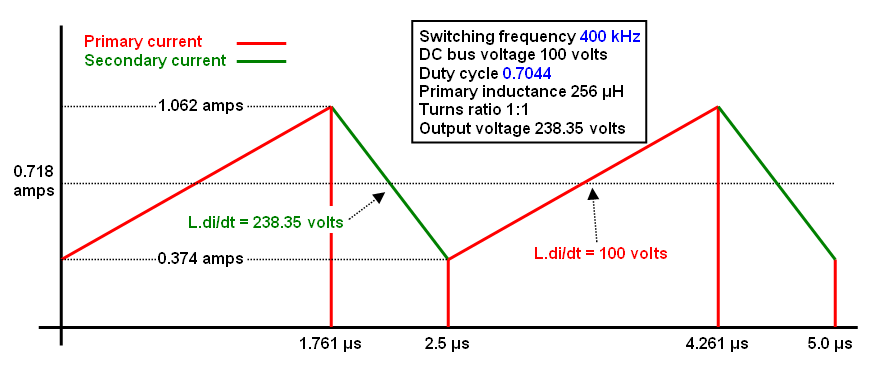
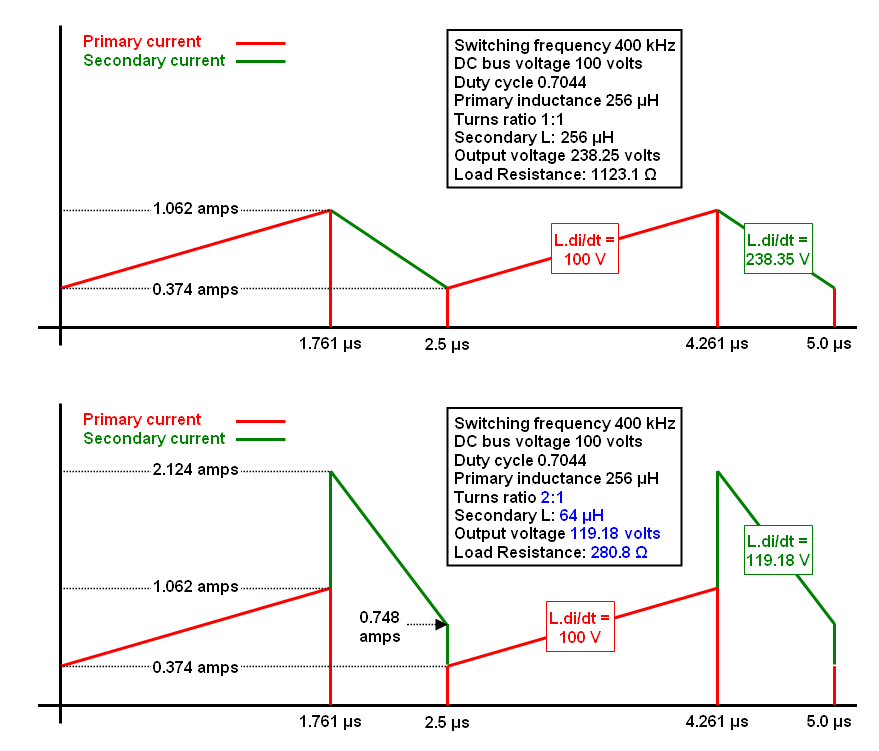
- you are analyzing a coupled choke , not a transformer nor a 2 port circuit with linear transfer function.
The primary turns, \$Np\$ are selected to satisfy the AC voltage stress (volt-seconds) and the core AC saturation properties:
\$\frac{V\:T\:}{B\:Ae}=Np\$
- Np is minimum primary turns
- V is the maximum primary DC voltage (volts)
- T is the maximum “on” period for Q1 (microseconds) B is the AC p-p flux swing (tesla) typically 200 mT for ferrite
- Ae is the effective center pole area of the core (mm2)
Energy in the primary coil is transferred to the secondary coil during the "off" state of the flyback operation.
\$E = \frac{1}2LI^{2}\$ (joules)
\$V_{L} = (V_{i}-V_{o})D-V_{o}\$
then modulating duty cycle, \$D\$ with signal \$\delta\$ to get AC voltage \$v_L\$ on \$L\$
\$v_L = (V_i+V_o)\delta - V_o(1-D)\; ≈ (V_i+V_o)\delta \;\$
\$i_L = \frac {v_L}{j\omega L} = -j \frac{V_i+V_o}{\omega L}\delta \$
during the "off" state
\$I_o=I_L (1-D)\$ then differentiating ac current with resulting \$i_L\$ having in-phase and 180' out of phase with \$\delta\$
\$i_o=-j\frac{(V_i+V_o)(1-D)}{\omega L}\delta -I_L\delta =-j \frac{V_i}{\omega L}\delta -I_Ld\$
The RHP Zero becomes \$ \omega_z=\frac{V_i}{LI_L}=\frac{R_oV_i^2}{LV_o(V_i+V_o)}\$
By using primary current sensing and current control loop, stability improves greatly.
I would like to give credit to above from one of the best SMPS designers I have briefly worked with in my past life as Test Eng Mgr at Burroughs.(mid'80's)
I hope this encourages you to buy one of his many books on the subject of SMPS Design... Tony
"in current, the immediate effect of trying to increase current is to cause a short-term decrease in output current. (This is a transitory 180° phase shift between cause and effect). This short transitory phase shift is the cause of the right-half-plane-zero in the transfer function. It is a non-compensatable dynamic effect and forces the designer to provide a very low-frequency roll off in the control loop to maintain stability. Hence transient performance will not be good. The flyback converter in the continuous mode has a boost-like converter characteristic and any converter or combination of converters that have a boost-type characteristic will have the right-half-plane-zero problem"
.
"The feature which makes it valuable for high output voltages is that it requires no output inductor. In forward converters, discussed above, output inductors become a troublesome problem at high output voltages because of the large voltages they have to sustain. Not requiring a high voltage free-wheeling diode is also a plus for the flyback in high voltage supplies."
.
"A further advantage for high voltage applications is that relatively large voltages can be obtained with relatively fewer transformer turns. "
.
"the discontinuous mode, with an inherently smaller transformer magnetizing inductance, responds more quickly and with a lower transient output voltage spike to rapid changes in output load current or input voltage. Second, because of a unique characteristic of the continuous mode (its transfer function has a right-half-plane-zero, which affects feedback loop stabilization), the error amplifier bandwidth must be drastically reduced to stabilize the feedback loop."
"Often, the effects of the layout, leakage inductance, output capacitor ESR, and circuit losses are unknown. As a result, it may be more expedient to measure the ripple current in the prototype unit and establish, or (if it has previously been calculated) confirm the final RMS values with CT's and true RMS meters."
Keith Billings, President of DKB Power Inc. and engineering design consultant, has over 46 years of experience in switch-mode power supply design. He is a Chartered Electronics Engineer and a full member of the former Great Britain’s Institution of Electrical Engineers (now the Institution of Engineering and Technology).
- i.e. It will oscillate unless loop bandwidth is drastically reduced.
- avoid saturation and flux walk or drift inside the BH loop for CCM.