Theoretical Derivation
The antenna electric field pattern of array antenna consists of isotropic radiator can be given by
where N is the number of antenna elements
d is the spacing between antenna elements
In order to find the beamwidth (3 dB), the above equation should be equated to
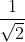and solve for
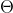
The solution will come to be as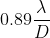 
where D is the total aperture distance and can be approximated as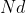 
For spacing of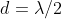 
the equation simplifies and can be approximated as 
This assumption holds accurate for larger antenna elements.
Thus beam width of planar antenna can be represented as
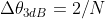
Angular Resolution Using FFT Over Antenna Elements
In order the prove the lemma that the angular resolution obtained by performing the FFT across antenna dimension equals to the beamwidth of the antenna array, we have to obtain the angular resolution using FFT.
The below diagram shows the frequency obtained due to path difference between the antenna elements which occurred due to the angle of arrival other than the broadside angle
The frequency resolution using FFT can be represented as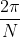 
where N is the number of samples but in our case it is equal to number of antenna elements.
The angular resolution 
can be found by equating the difference in frequency of different angles
and
&space;%7D%7B%5Clambda%7D "w_2 = \frac{2 \pi d sin\left(\Theta + \Delta \Theta \right ) }{\lambda}")
The frequency resolution becomes:
&space;%7D%7B%5Clambda%7D&space;-&space;%5Cfrac%7B2&space;%5Cpi&space;d&space;sin%5CTheta&space;%7D%7B%5Clambda%7D "w_2 - w_1 = \frac{2\pi}{N} = \frac{2 \pi d sin\left(\Theta + \Delta \Theta \right ) }{\lambda} - \frac{2 \pi d sin\Theta }{\lambda}")
Solving we get
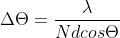and if we put 
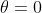and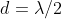
we get the same resolution as the beamwidth i.e
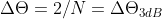
Summary
Thus for summarizing the above discussion using FFT we can only achieve the max angular resolution equals to the beamwidth of the antenna array which is equal to angular resolution = antenna array beamwidth = 2/N
Note
The answer will be in radians
Edit: *The derivation holds only for isotropic radiating element, in case of non isotropic element "array factor" should compensate the specific antenna's field pattern.
*Also the beam width is approximated to 2/N i.e. N should be large enough to get the equality
Reference: Fundamentals of Radar Signal Processing by Mark A. Richards, 2005
