So, I am familiar with what a Gilbert cell is and what it does, but I have looked through all the resources I can find trying to understand it and I just can't wrap my head around it.
Just from looking at it, I can tell it has something to do with differential amplifiers; it looks very much like a long-tailed pair of long-tailed pairs.
It could just be that my brain is fried from working on final projects and final exams, but I can't seem to understand it.
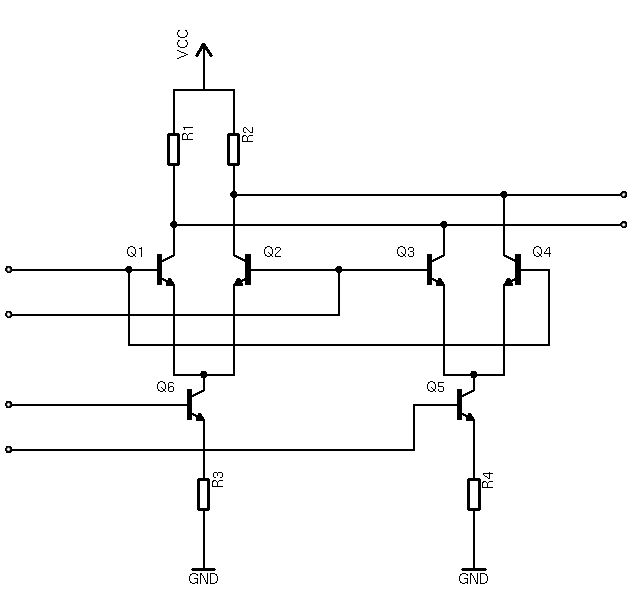
it looks very much like a long-tailed pair of long-tailed pairs.
That's exactly what it is.
Q6 and R3 form a voltage controlled current sink, which allows a total current proportional to the voltage at the base of Q2 to flow through the long tailed pair Q1, Q2. Q5 and R4 do the same thing, with the voltage at the base of Q5 determining the total current through the second long-tailed pair Q3, Q4.
A balanced AC input to Q6base and Q5base will thus control the ratio of currents flowing trough the two upstream diff pairs: if the voltage difference between Q6base and Q5base is zero, the currents are equal. If Q6base is higher than Q5base, Q6 will sink more current than Q5, and vice versa. Keep in mind that the sum of the two currents is always the same, unless the input is overdriven.
Assume for now that the lower input is zero, and thus the total current is shared equally by the two long tailed pairs (Q1, Q2 and Q3, Q4). Note how the outputs of the two long tailed pairs are cross connected. Q1, Q2 will have an opposite effect on the output relative to Q3, Q4 for any nonzero signal to the bases of Q1/Q4 and Q2/Q3. As they are constantly "fighting" for control, they cancel each other out, leaving the output of the circuit at zero (differential) voltage.
The gain of a diff amp/long tailed pair is proportional to the common mode current flowing through it. Thus the lower input controls how much weight one diff amp has over the other: if the non-inverting pair has more current flowing through it than the inverting one, the gain of the Gilbert cell is positive, and vice versa.
The six-transistor 'multiplier' (or modulator, or demodulator) circuit has continuous output dependent on two differential-mode input signals. Call the two inputs A (the Q5 and Q6 base voltages) and B (Q1 and Q2 bases). Then the differential output (Q1 and Q2 collectors) can be expressed (because it is a continuous, smooth function of the inputs) as $$Vout = C_{00} + C_{10} A + C_{01} B + C_{20} A^2 + C_{11} A B + C_{22} B^2 + ...$$ This is the Taylor series expansion, with higher order terms omitted. Note, by symmetry, $$C_{00}= C_{01} = C_{10} = C_{20} = C_{02} = 0$$ This means that the lowest-order nonvanishing term is the product A X B. So, for small signals (which allows us to ignore the higher terms), the circuit is an analog multiplier of the A and B signals.
A Gilbert Cell (actually Barry Gilbert patiently explains is NOT his creation; he does claim the translinear multiplier cell, very similar) is the analog version of an Exclusive Or gate.
If you drive the 2 sets of left inputs with differential square waves, you will see the (differential) EXOR on the right hand outputs. Assuming you drew correctly.
The Gilbert Cell is a doubly-balanced mixer, suppressing the energy from the bottom signals (usually the RF) and suppressing the energy from the top signals (usually the square-wave oscillator); this suppression leaves just the (weak?) SUM and DIFFERENCE; in a receiver, these will be weak if the RF input is weak; suppressing the RF and LO makes downstream filtering easier. In a Transmitter, suppressing the RF and LO leaves the SUM and DIFFERENCE as before, but your frequency plans (to filter out all but what you wish to transmit) may become easier and cheaper to implement.
