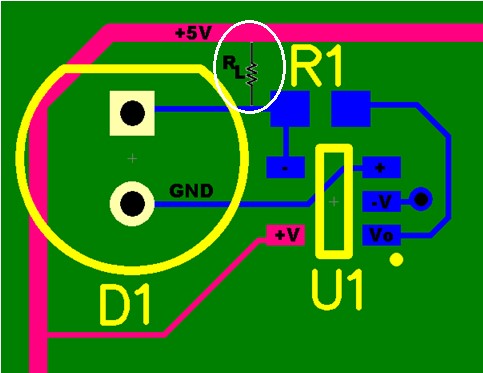
For a highly-sensitive electrometer one needs not only high resistance but also a really small capacitance to achieve certain AC behavior e.g. stability of the opamp.
The figure below shows a typical example of a electrometer circuit with an opamp and can be extracted from this Analog Devices application note.
This is equal to the following circuit.
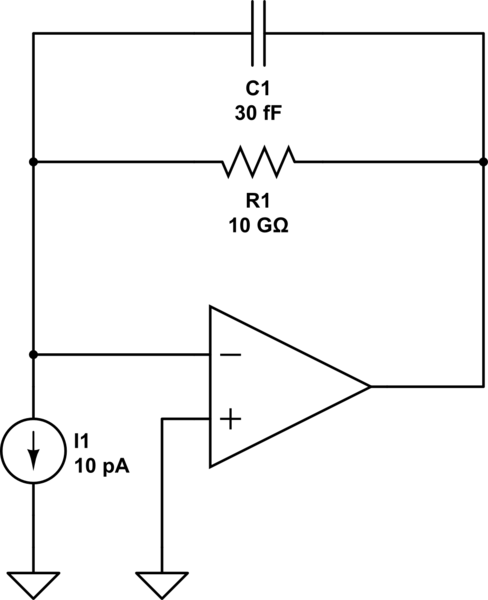
simulate this circuit – Schematic created using CircuitLab
The values given in the schematics plus the noise budget calculations I did, result in a output range (worst-case, \$T_A = 25°C\$) from \$320\, µ V\$ (noise floor) up to \$ 3.3 \, V\$ when a dual power supply (\$V_+=3.3 \, V\$ and \$V_-=-3.3 \, V\$) is used. According to simulation a \$3 dB\$ bandwidth of \$ 530.52 \, Hz\$ works well for a pulsing of the input signal with \$ 200 \, Hz\$. The idea is suppressing noise below this frequency as you mix your signal up from \$0 \, Hz\$ to a higher \$f_{IF}\$. The bandwidth of the system is mainly determined (or from a ideal POV only determined) by the feedback capacitor and can be calculated with
$$f_{3dB} = \frac{1}{R_f C_f 2 \pi}$$
So the capacitance needed is in the femtofarad range (\$30 \,fF\$). Now my question is if you have a feedback path (cf. picture), where you want to avoid coupling impedances (to somewhere outside of this low-current path) of any kind (you use all kind of fancy guarding techniques e.g. via fencing) would you say it is legitimate to use an embedded, self-made pcb cap? You know like basically two plates on TOP and BOTTOM.
I mean the calculations say, that for \$30 \, fF\$ you need two plates with an area of approx \$1.153 \, mm^2\$ when you use FR4 (\$\epsilon_r = 4.7\$) and a PCB with \$1.6 \, mm\$ thickness.
So you have
$$ C = \frac{\epsilon A}{d} $$
which can be rewritten as
$$ A = \frac{C d}{\epsilon} $$
If I got it correctly you can just draw two squares on the PCB on both sides, each of them has the following dimensions $$\sqrt{A} \cdot \sqrt{A}.$$
Conclusion: Can I use an embedded capacitance when working in the femtoampere (YES \$10^{-15}\$ A) range or am I missing out on something here? It is quite hard to get such small capacitances, which is the reason why a embedded capacitor shall be used.