21
\$\begingroup\$

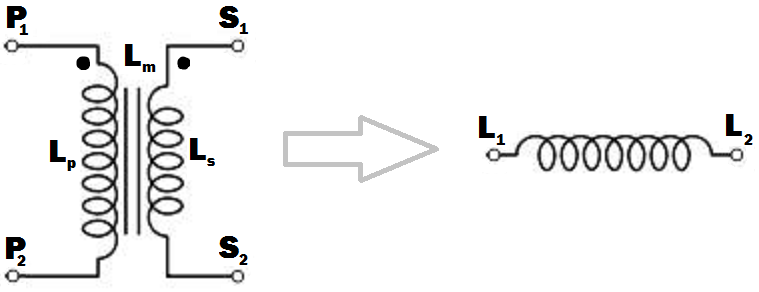
enter image description here

Lp: Self inductance of the primary winding.
Ls: Self inductance of the secondary winding.
Lm: Mutual inductance between the primary and secondary windings.

Assume that I need an iron core inductor with large inductance to use under 50Hz or 60Hz.

How do I obtain an inductor from the given transformer in the image? I don't want to use any other circuit elements unless it is absolutely required. The dot convention of the transformer is given in the image; terminal connections must be done so that the inductance of the resulting inductor must be maximum (I think that happens when the fluxes generated by the primary and secondary windings happen to be in the same direction inside the transformer core).

I'm expecting an answer like "Connect \$ P_2 \$ and \$ S_2 \$ to together, \$ P_1 \$ will be \$ L_1 \$ and \$ S_1 \$ will be \$ L_2 \$ of the resulting inductor.".
I understand that I can use the primary and secondary windings separately by making the unused winding open, but I'm looking for a smart way of connecting the windings so that the resulting inductance will maximize.

What will be the inductance of the inducter in terms of \$ L_p \$, \$ L_s \$ and \$ L_m \$ ?
What will be the frequency behavior of the resulting inductor? Will it have a good performance at frequencies other than the original transformer was rated to run in.

\$\endgroup\$
1
  • 1
    \$\begingroup\$ It's important to note that a transformer makes a lousy inductor, like trying to use a steel pole for an archery bow. The bow needs to be flexible, like an inductor core that has an air-gap in it. No air-gap, no 'flexibility', inefficient energy storage. I could put this in terms of B and H fields if you like, H field is bow deflection, B field is pull strength. A gapped core has a much higher H for the same B, so stores more energy for the same peak B, B is limited by the iron, pull strength is limited by your arm. That's why a transformer core is not gapped. \$\endgroup\$
    – Neil_UK
    Commented Jul 6, 2016 at 19:23

2 Answers 2

Reset to default
23
\$\begingroup\$

How do I obtain an inductor from the given transformer in the image? ... So that the inductance of the resulting inductor must be maximum.

  • Connect the undotted end of one winding to the dotted end of the other.
    eg P2 to S1 (or P1 to S2) and use the pair as if they were a single winding.
    (As per example in diagram below)

  • Using just one winding does NOT produce the required maximum inductance result.

  • The resulting inductance is greater than the sum of the two individual inductances.
    Call the resultant inductance Lt,

    • Lt > Lp
    • Lt > Ls
    • Lt > (Lp + Ls) !!! <- this may not be intuitive
    • \$ L_t = ( \sqrt{L_p} + \sqrt{L_s}) ^ 2 \$ <- also unlikely to be intuitive.
    • \$ \dots = L_p + L_s + 2 \times \sqrt{L_p} \times \sqrt{L_s} \$

Note that IF the windings were NOT magnetically linked (eg were on two separate cores) then the two inductances simply add and Lsepsum = Ls + Lp.

What will be the frequency behavior of the resulting inductor? Will it have a good performance at frequencies other than the original transformer was rated to run in.

"Frequency behavior" of the final inductor is not a meaningful term without further explanation of what is meant by the question and depends on how the inductor is to be used.
Note that "frequency behavior" is a good term as it can mean more than the normal term "frequency response" in this case.
For example, applying mains voltage to a primary and secondary in series, where the primary is rated for mains voltage use in normal operation will have various implications depending on how the inductor is to be used.Impedance is higher so magnetising current is lower so core is less heavily saturated. Implications then depend on application - so interesting. Will need discussing.

Connecting the two windings together so that their magnetic fields support each other will give you the maximum inductance.

When this is done

  • the field from current in winding P will now also affect winding S

  • and the field in winding S will now also affect winding P

so the resultant inductance will be greater than the linear sum of the two inductances.

The requirement to get the inductances to add where there 2 or more windings is that the current flows into (or out of) all dotted winding ends at the same time.

enter image description here

  • \$ L_{effective} = L_{eff} = (\sqrt{L_p} + \sqrt{L_s})^2 \dots (1) \$

Because:

Where windings are mutually coupled on the same magnetic core so that all turns in either winding are linked by the same magnetic flux then when the windings are connected together they act like a single winding whose number of turns = the sum of the turns in the two windings.

ie \$ N_{total} = N_t = N_p + N_s \dots (2) \$

Now: L is proportional to turns^2 = \$ N^2 \$
So for constant of proportionality k,
\$ L = k.N^2 \dots (3) \$
So \$ N = \sqrt{\frac{L}{k}} \dots (4) \$

k can be set to 1 for this purpose as we have no exact values for L.

So

From (2) above: \$ N_{total} = N_t = (N_p + N_s) \$

But : \$ N_p = \sqrt{k.L_p} = \sqrt{Lp} \dots (5) \$
And : \$ N_s = \sqrt{k.L_s} = \sqrt{L_s} \dots (6) \$

But \$ L_t = (k.N_p + k.N_s)^2 = (N_p + N_s)^2 \dots (7) \$

So

\$ \mathbf{L_t = (\sqrt{L_p} + \sqrt{L_s})^2} \dots (8) \$

Which expands to: \$ L_t = L_p + L_s + 2 \times \sqrt{L_p} \times \sqrt{L_s} \$

In words:

The inductance of the two windings in series is the square of the sum of the square roots of their individual inductances.

Lm is not relevant to this calculation as a separate value - it is part of the above workings and is the effective gain from crosslinking the two magnetic fields.

[[Unlike Ghost Busters - In this case you are allowed to cross the beams.]].

\$\endgroup\$
1
  • \$\begingroup\$ @hkBattousai - Thanks. hmm - doesn't copy to you if you are editor only. \$\endgroup\$
    – Russell McMahon ♦
    Commented Jun 14, 2012 at 18:34
7
\$\begingroup\$

Just use the primary or the secondary with the other winding open-circuit. If you use the primary, the inductance will be \$L_P\$, and if you use the secondary it will be \$L_S\$ - by definition.

But I'm not sure what you are expecting to do with this (you say you don't want to use any other circuit elements .... ?).

The frequency response will depend on what other circuit elements you use. Assuming you are trying to implement an L/R or L/C low-pass filter, a mains transformer should give rejection up to a few tens of kHz before other factors (such as winding capacitance) have an effect.

Be aware though that the primary of a mains transformer will have higher inductance and will be rated for higher voltage and lower current than the secondary. You should also ensure that if you do not use one winding is well insulated, especially if you are using the secondary. This is because very high voltages could be induced in the primary if the secondary current changes rapidly.

EDIT

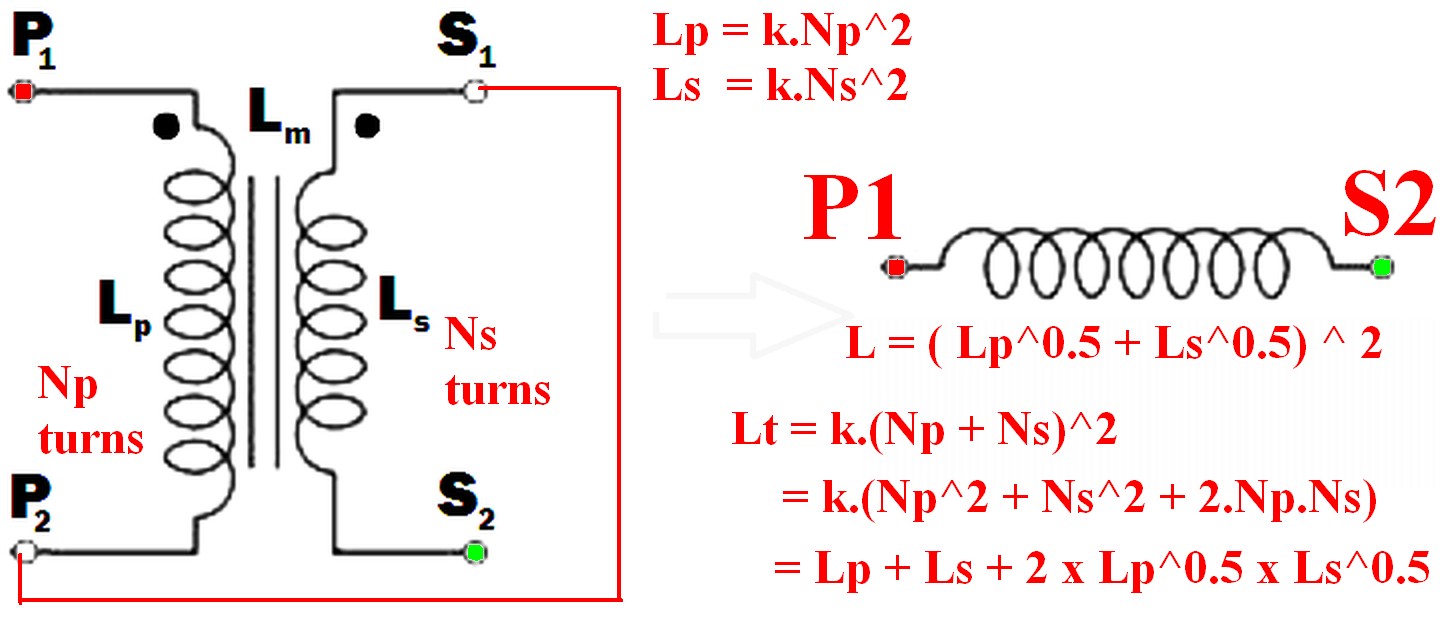
I see from your edits that you want to connect the windings together. The primary and secondary inductances can be calculated from their turns by the formulae ..

SECOND EDIT

I have rewritten this next part to make it less mathematical, more intuitive, and to distinguish it from other answers here.

The voltage induced across an inductor is proprrtional to the rate of change of current through it, and the constant of proportionality is the inductance L.

V1 = L * (rate of change of current through winding)

With coupled coils, the induced voltage has an extra factor due to the rate of change of current through the other winding, the constant being the mutual inductance Lm.

V2 = Lm * (rate of change of current through the other winding)

So in general, the voltage across the inductor is the sum of these:- (using your symbols)

Vp = Lp * (rate of change of primary current) + M * (rate of change of secondary current)

and for the secondary :-

Vs = Ls * (rate of change of secondary current) + M * (rate of change of primary current)

If we wire the primary and secondary in series, the currents are the same and the voltages will add or subtract,

depending on which way round we connect the windings together.

\$V_{total} = V_P \pm V_S = ( L_P \pm L_M + L_S \pm L_M )\$ * (rate of change of current)

SUMMARY

But this is just the same as if we had an inductor with inductance :-

\$L_t = L_p + L_s \pm 2L_m\$

If we connect the windings so that S1 is connected to P2, the current will flow the same way through both windings, the voltages will add and we maximize the inductance, so :-

\$L_t = L_p + L_s + 2L_m\$

If there is no coupling (for instance if the windings were on separate cores), the mutual inductance will be zero and the primary and secondary inductances will add as you might expect. If the coupling is less than perfect, a proportion k of the flux from one winding will couple into the other winding, with k varying from 0 to 1 as the coupling improves. The mutual inductance can then be expressed as :-

\$L_m = k\sqrt{L_pL_s}\$

and

\$L_t = L_p + L_s + 2k\sqrt{L_pL_s}\$

This is the same as Russell's answer if k=1 (perfect coupling) but I disagree that the mutual inductance is not relevant. It is.

\$\endgroup\$
6
  • \$\begingroup\$ Mike - Unfortunately this solution is just plain wrong (regardless of what votes people give it). Windings can be joined together and the inductance gained is as if there was a single winding with all the turns combined. As the two sets of magnetic fields interact the new inductance is greater than the sum of the two inductances by themselves - see my answer for details. \$\endgroup\$
    – Russell McMahon ♦
    Commented Jun 14, 2012 at 13:51
  • \$\begingroup\$ @RussellMcMahon - I didn't say that the windings couldn't be joined together. I didn't address the issue because the OP did not say in his pre-edited post that he wanted to do this. \$\endgroup\$
    – MikeJ-UK
    Commented Jun 14, 2012 at 14:05
  • \$\begingroup\$ Mike - The original question before editing was somewhat less clear but said: " ... terminal connections must be done so that the inductance of the resulting inductor must be maximum (I think that happens when the fluxes generated by the primary and secondary windings happen to be in the same direction inside the transformer core). What will be the inductance of the inducter in terms of Lp, Ls and Lm ?" \$\endgroup\$
    – Russell McMahon ♦
    Commented Jun 14, 2012 at 14:15
  • \$\begingroup\$ Ah. Deja vu. At least it's now correct :-). \$\endgroup\$
    – Russell McMahon ♦
    Commented Jun 14, 2012 at 17:08
  • \$\begingroup\$ @RussellMcMahon - Firstly a simple "The OP edited his question - you might like to amend your answer" would have done rather than reaching for the 44 Magnum. Second, I was not breaching your copyright, but just trying to show the effect of mutual inductance which your answer states is "not relevant". \$\endgroup\$
    – MikeJ-UK
    Commented Jun 15, 2012 at 8:15

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.