I am trying to calculate the small signal voltage gain of this circuit but I am having a problem comprehending the last step.
I rewrote the circuit like this
But the solution says this:
Which would equal to $$U_a/U_e = -\frac{\beta i_B R_c}{r_{BE}+R_E(1+\beta)}$$ which is nearly \$-R_c/R_E\$.
Why can I ignore the \$R_1 \parallel R_2\$ resistance but not the \$R_c\$ resistance?
A resistor that comes in parallel with a voltage source has effectively nothing to do with the rest of the circuit and can safely be ignored. See the following circuits that are equivalent:
In your circuit since you have vi in parallel with R1||R2 you can ignore R1||R2 as it doesn't matter to the circuit. However, if the signal source has some non-zero impedance, say Rs, you could no longer ignore R1||R2, as this in parallel with \$R_B=r_\pi + (\beta+1)R_E\$, makes a resistive divider at the base with the net effect that it decreases the voltage gain of the circuit as a fraction of \$\frac{R_1||R_2||R_B}{R_1||R_2||R_B+R_S}\$.
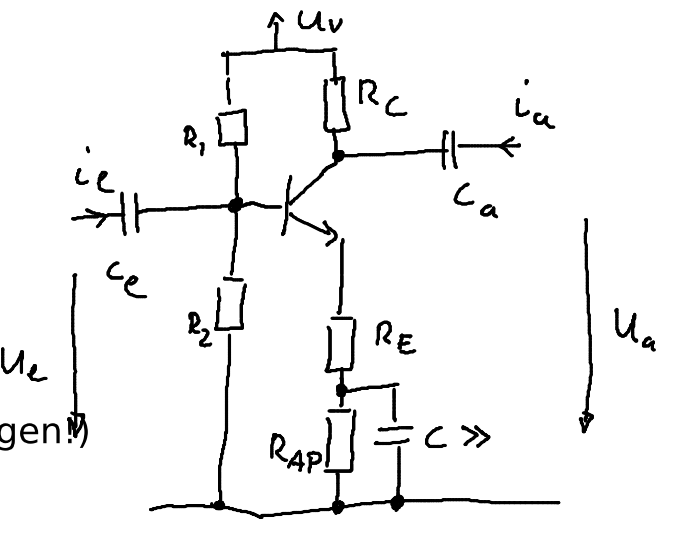
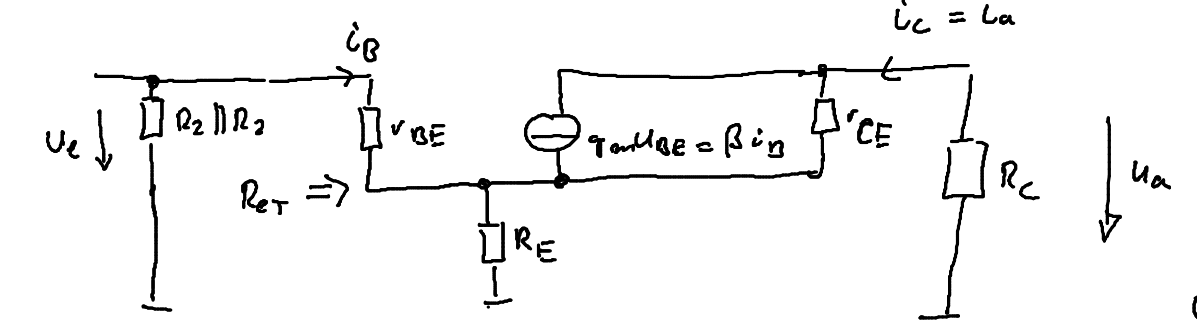
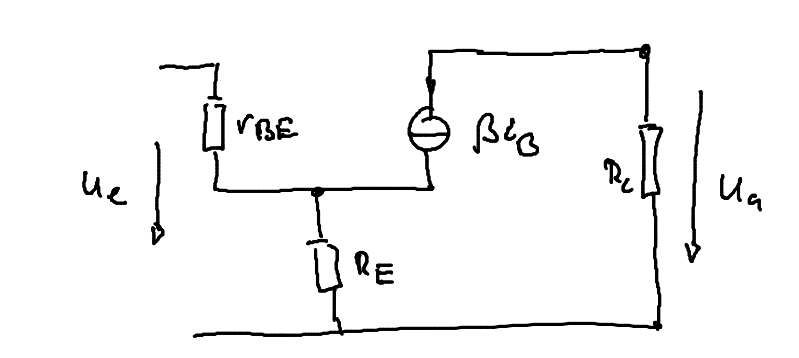
Unless the problem contains some kind of hint or special instruction, you cannot ignore R1 and R2. The input voltage, Ue, is applied at the capacitor, Ce. Ce and R1||R2 form a high-pass filter which affects the overall gain.
Sometimes, if a professor wants you to ignore a capacitor, he/she may label the capacitor as "infitnite" or may say "assume Ce is very large" or "consider gain in the passband only". Those would all be clues that you can ignore R1||R2. In the absence of any such clues, you cannot ignore it.
For example, it seems that the capacitor in parallel with RAP is intended to be so large that we can ignore RAP. (At least, that is what I think the double "greater-than" symbol means).
I would discuss the matter with the professor for sure. Just do it in a respectful way with a smile, not in a challenging way.
Examine the collector characteristics of a bipolar transistor
If the biasing network, and I*R of the collector resistor, allow several volts across emitter-collector, the transistor provides considerable and useful isolation between base and collector.
Part of the design thinking of bipolars (and FETS) is how to exploit this compliance of the collector; one way is to view the bipolar (and FET) as controlled current sources, using delta Vbase to modulate the collector current small-signal changes; the parameter "gm" or transconductance exactly describes this deltaVin -> deltaIout behavior.
"gm" for a bipolar, at room temperature, is Ie / 0.026. Thus at Ie= 26mA, bipolar GM is 1.0, that is 1 millivolt input change produces 1milliAmp change in collector current.
