How to identify which transistor is in active region and which one is in saturation?

Question

In the following amplifier circuit, voltage gain is required. Capacitors act as open circuit for DC analysis but short circuit for AC analysis (consider capacitor value to be very big). 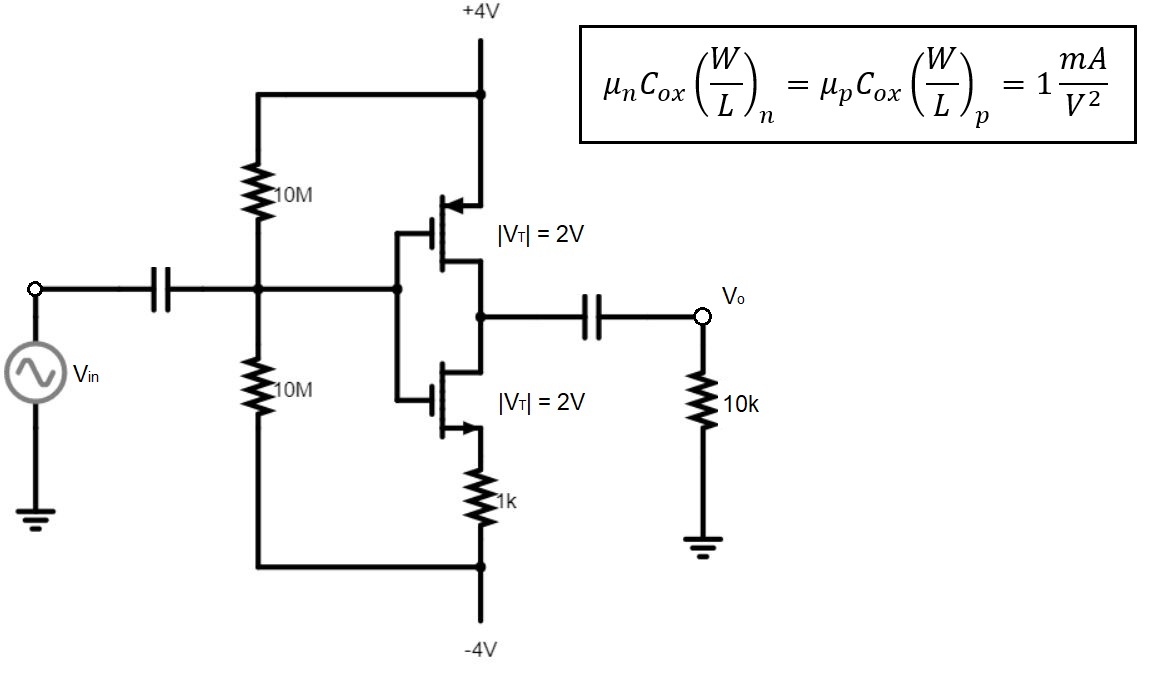Now MOSFET have 2 different equations for drain current, one for ohmic region one for saturation. How can one determine which equation to use here and for which transistor i.e. which one of the transistor is saturated and which one is not. (Early voltage Va = 50 Volts) Here is the problem I'm facing if I consider both transistors in saturation : Due to symmetry in gate circuit

\$ V_{G} = 0 V\$

Now as for pMOS

\$I_D = \frac{k_p}2 (V_{GS}-V_T )^2\$

\$ = \frac{1}2 (-4 + 2 )^2 = 2 mA\$

Therefore voltage at the source of nMOS will be

V_S = -4 + (1k-ohm x 2mA) = -2 Volts

So for nMOS V_GS = V_G - V_S = 0 - (-2) = +2 V (= V_T)

So overdrive voltage for nMOS is zero. This means no current should flow through it. But 2 mA drain current is from pMOS. That's why I'm confused weather both transistors are in saturation or one of them is in ohmic region?

Answer 1

At first, try to read this When an NMOS utilizes a PMOS current source load, which transistor is acting as the current source?

Now you need to check what current every MOS want to pass (forced) in a saturation region.

For PMOS:

$$I_{D2} = \frac{Kp}{2}(V_{GS} - V_T)^2 = 0.5m((-4V)-(-2V))^2 = 2mA $$

But the NMOS want to force

$$\frac{V_G - V_{GS}}{R_S} =\frac{Kp}{2}(V_{GS} - V_T)^2 $$

$$\frac{4V - V_{GS}}{1k\Omega} =0.5m(V_{GS} - 2)^2 $$

After you solve this you will get two solutions:

\$ Vgs =-1.236V\$ or \$ Vgs = 3.23607V\$

The first solution makes no sense, so \$ Vgs = 3.23607V\$ and \$I_{D1} = 0.763932mA \$

Hence \$I_{D1} < I_{D2}\$ we conclude that NMOS will work in saturation and PMOS in a triode region.

All that remains is to find \$V_{DS}\$ for a PMOS.

We can do this by solving this equation

$$0.763932mA = Kp(4V - 2V)V_{DS} - \frac{V_{DS}^2}{2}$$

And this time this solution is true \$V_{DS} = 0.427697V\$

Therefore the voltage at MOS's drain's is \$4V - 0.427697V = 3.57V\$

Answer 2

2 different equations for drain current, one for active region one for saturation.

You're mixing FET and Bipolar vocabulary, which is confusing. Bipolars have Saturation and Active region (and quasi-saturation in-between). Saturation occurs at low Vce, when the B-E diode passes high Ib.

For FETs the terms are the opposite:

• "Saturation region" is the mode you use to build an amplifier, as is the case here. The term "active region" is much clearer, so that's what I'd use. The FET behaves as a voltage-controlled current source which is characterized by Id as a function of Vgs (and Vds), and transconductance is d(Id)/d(Vgs).

• "Triode mode or linear region (also known as the ohmic mode)" occurs when the FET behaves like a resistor (hence "ohmic mode" is the most explicit).

If you want to build a common source amplifier (which is the case here) you need transconductance, which means using the active mode. Thus, do the calculatoins assuming active mode, then check that the FETs are indeed in active mode (saturation).