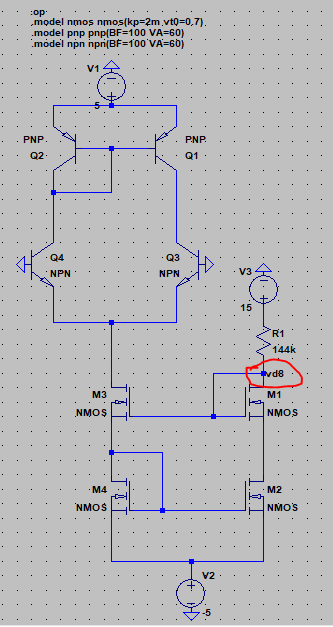
Below I have a current mirror loaded differential amplifier. Can somebody please Just give me an idea of how to find the drain voltage, vd8. I am stuck because I don't see the relationships that can lead to finding vd8 based on this configuration. Thank you.
This is a very easy task. You already should now that in the current mirror
\$I_{D1} = I_{D2} = I_{D3} = I_{D4}\$
And this can only be true if all transistor have the same \$V_{GS}\$
Therefore the current through the \$R_1\$ resistor is
\$I_{R1} = I_{D1} = \frac{20V - 2V_{GS}}{R_1}\$
Hence the equation needed to solve for \$V_{GS}\$ is:
$$\frac{20V - 2V_{GS}}{R_1} = \frac{K_p}{2}(V_{GS} - V_T)^2$$
And the solution is \$V_{GS} \approx 1.05\textrm{V}\$ and the current is \$I_{R1} \approx 124.3\mu \textrm{A}\$
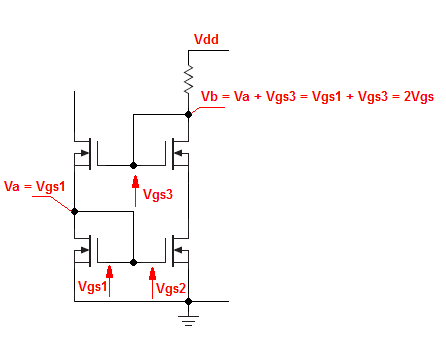
This picture tries to explain why I use \$2V_{GS}\$
Also, notice that from KVL we can wite
\$I = \frac{V_{DD} - V_B}{R}\$
And in your circuit \$ V_{DD} = 15V + 5V = 20V\$
Assuming that all transistors have the same size such that the currents in both branches are equal, you can also assume equal biasing conditions for all transistors. They all have the same \$V_{GS}\$, \$I_{DS}\$ and \$V_{DS}\$.
Combined with the current mirror references that have \$V_{GS} = V_{DS}\$, you can easily find that
$$V_{D8} = -5V + V_{DS2} + V_{DS1} = -5V + 2\cdot V_{GS4}$$
You can potentially use the equation
$$I_{DS} = K_n\frac{W}{L} (V_{GS} - V_{TH})^2$$
To find the \$V_{GS}\$ you're interested in. But you'll need the bias current.
