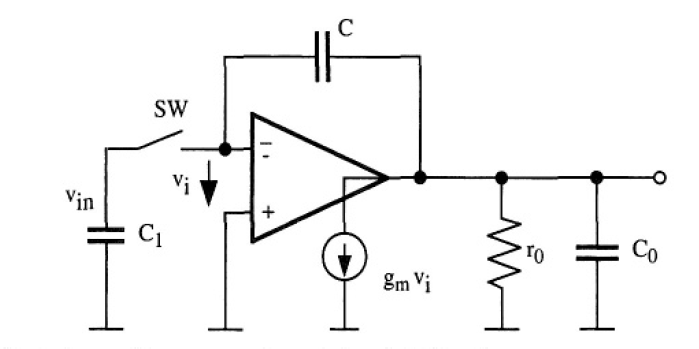
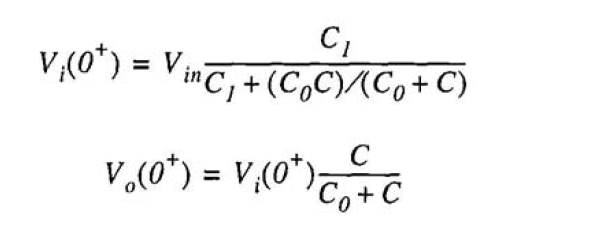Let's consider this circuit:
and suppose that the capacitor C1, charged at the input voltage Vin, at time t=0 is connected by the switch to the virtual ground. Assume also that C and C0 are initially discharged.
First question: How does the book I'm reading show that:
Second question: If C1 has an initial voltage vin, how can the switch modify the voltage of C1 to the virtual ground? I know that the voltage across a capacitor cannot change instantly.
Thanks
Consider the charge on C1 (charege Q = CV) then consider that at the instant the perfect switch closes, that charge is then shared amongst three capacitors and the net value of those three capacitors is the denominator in the formula i.e. C1 in parallel with the series combination of C and Co.
Charge therefore rapidly redistributes and the result is that the voltage falls because charge is not lost. Nothing to do with the opamp (yet) because it all happens far too quickly with ideal components.
The 2nd formula in your first question is of a capacitive potential divider using C and Co. This bit should make sense now (hopefully) and agin it happens in virtually no time at all.
For your 2nd question, forget about a virtual ground; this won’t come into play until the opamp has got wise to its input voltage disparity and slowly (a few microseconds or milliseconds) restore the virtual earth situation.
