Why do R and C have to be small for differentiator circuit

Question

I'm a beginner studying The Art of Electronics, and on page 25 they introduce the differentiator. Basic circuit like so:

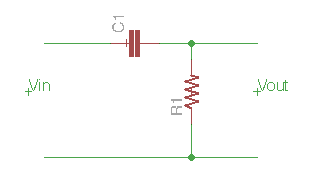
They give the complete equation:

$$ I = C \frac{d}{dt}(V_{in} - V) = {V\over R} $$

I understand this so far. But then they say: if we choose R and C small enough so that \$\frac{dV}{dt} \ll \frac{dV_{in}}{dt}\$, then...

$$ C\frac{dV_{in}}{dt} \approx \frac{V}{R} $$

This I don't follow. Can someone elaborate or explain a bit more? I see why the above equation makes it a differentiator -- V is proportional to \$\frac{dV_{in}}{dt}\$. But why does a small R and C cause the one derivative to me much less than the other?

Answer 1

If you rearrange the first equation, you get

\$C\dfrac{dV_{in}}{dt} = \dfrac{V}{R} + C\dfrac{dV}{dt}\$

So if you reduce C enough, you'll make the derivative term on the right insignificant compared to V/R, and you'll get your second equation.

Alternately, if you reduce R, you'll make the V/R term larger, and again the right-hand-side derivative term will become insignificant, and you'll get the desired result.

So I'd say it's not that you must decrease R and C together, but you do have do some combination of reducing R and reducing C to make the circuit work like a differentiator.

Answer 2

Another way to look at this is in the frequency domain rather than the time domain.

What you have is a passive 1st order RC high pass filter. The transfer function is:

\$\dfrac{V_{out}}{V_{in}} = \dfrac{j\omega RC}{1 + j \omega RC}\$

When \$ \omega << \frac{1}{RC}\$

\$\dfrac{V_{out}}{V_{in}} \approx j \omega RC\$

But this is just the transfer function of an ideal differentiator.

The requirement given in the text is essentially the same requirement as above. The basic idea is that this high-pass filter "looks like" a differentiator well below the corner frequency.

Answer 3

Assuming RC is small, we want to solve:

$$RC\frac{dV_{in}}{dt}=V+RC\frac{dV}{dt}.$$

I was a bit unsatisfied as to why we would neglect the term RCV'(t) on the right, but not RCVin'(t) on the left. So instead let's find a solution using power series.

We will abbreviate the derivative as D, so:

$$DV_{in}=\frac{dV_{in}}{dt};\;DV=\frac{dV}{dt}.$$

Let's also abbreviate

$$\epsilon=RC.$$

The equation is then

$$\epsilon DV_{in}=V+\epsilon DV,$$ $$(1+\epsilon D)V=\epsilon DV_{in}.$$

Now, we 'divide both sides by (1+ϵD)', which can be made rigorous using power series. This gives the solution

$$V=\frac{1}{1+\epsilon D}\epsilon DV_{in}.$$

Assuming ϵ=RC is small, we can use the power series expansion

$$\frac{1}{1+x}\approx 1-x,$$

which is valid whenever x is small. This gives

$$V\approx \epsilon DV_{in}-\epsilon^2D^2V_{in}=RC\frac{dV_{in}}{dt}-(RC)^2\frac{d^2V_{in}}{dt^2}.$$

For small RC we can neglect the second term.

To be precise, we need (RC)D to be small. Note that this is unitless, since RC has units of time, and the derivative D has units inverse time. In other words, the time constant RC has to be small compared to the rate at which our signal Vin changes.