Equivalent DC circuit of 3 phase induction motor

Question

I'm currently trying to model a simplified circuit of an inverter + 3 phase induction motor. For now, I want to represent the DC link as an ideal independent voltage source. To keep this example simple, I also want to keep frequency and input voltage the same, though I expect to be changing these to simulate a VFD

I'm using https://myelectrical.com/notes/entryid/251/induction-motor-equivalent-circuit as reference

The output I want is motor torque and resulting current on the DC link.

So, given \$V_{DC}\$, what would \$I_{DC}\$ be in terms of inverter equations and induction motor equations?

I think I'm most of the way there, but I can't find a full set of equations for this, only separate equations for motors and inverters.

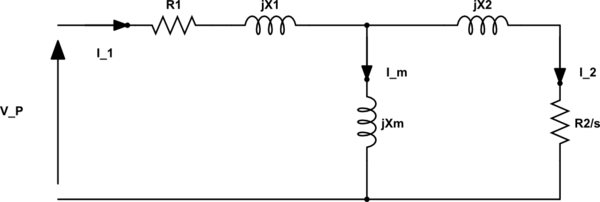
^{simulate this circuit – Schematic created using CircuitLab}

For input voltage to the motor, I think this is correct $$V_{L} = \frac{3}{\sqrt{2}\pi}V_{DC}\\ V_{P} = \frac{V_{L}}{\sqrt{3}}$$ It's my understanding that torque is per phase $$T = \frac{1}{2\pi n_s}\frac{R_{2}}{s}\frac{E_{2}^2}{\frac{R_{2}}{s}^2+X_{2}^2}$$ So total motor torque $$T_{3\phi} = 3T = \frac{3}{2\pi n_s}\frac{R_{2}}{s}\frac{E_{2}^2}{\frac{R_{2}}{s}^2+X_{2}^2}$$ To calculate back emf \$E_2\$: $$E_{2} = V_{P}-\frac{I_{1}}{Z_{1}}$$ Impedance per phase: $$Z_1=R_{1} + jX_{1}\\ Z_m=jX_{m}\\ Z_2=\frac{R_{2}}{s} + jX_{2}\\ Z=Z_{1}+\frac{Z_{m}Z_{2}}{Z_{m}+Z_{2}}$$ Would the calculation back to \$I_{DC}\$ be as follows? $$I_{1} = \frac{V_{P}}{Z}$$ $$I_{L} = \frac{I_{1}}{\sqrt{3}}$$ $$I_{DC} = \frac{3}{\sqrt{2}\pi}I_{L}$$

EDIT: Specifically, I want to know if the equations I have above are correct for determining the average current on the DC link (neglecting most losses for now)

Answer 1

The average DC current will be determined by the total power delivered to the motor plus the losses in the inverter. The reactive component of the motor current will be seen as bus capacitor ripple current. I don't believe you have taken that into consideration.

If the losses are ignored, the power delivered by the DC link is equal to the mechanical power delivered by the motor. The average DC current is the mechanical power in watts delivered by the motor divided by the DC bus voltage.