Regards Band Pass Filter with AmpOp

Question

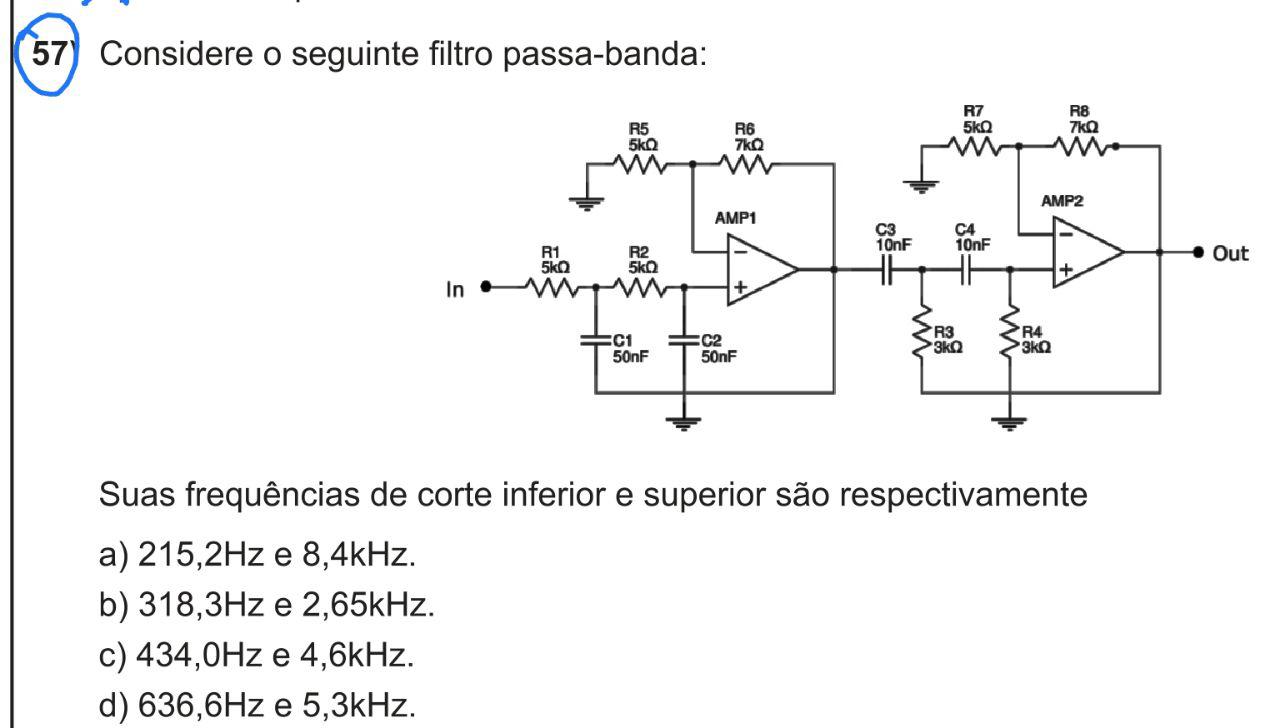
I've tried to solve this contest question regards the band pass filter (find the frequency at low-pass and high-pass filters) the picture below:

My attempt:

I consider that the first stage is 2 low-pass filter identically (R1 C1) and (R2 C_2) - circuit RC - \$R = 5\,k\Omega\$ and \$C = 50\,nF\$

So compute the cut-off frequency: \$ f_c = \frac{1}{2\pi R_1C_1} = \frac{1}{2\pi R_2C_2} = \frac{1}{2\pi \cdot 5\cdot 10^3\cdot 25\cdot 10^{-9}} \approx 636,6 Hz \$

Seems "ok".

Continue: when I look to the second stage I can see 2 high-pass filter (C3 and R3) and (C4 and R4)

cut-off frequency: \$ f_c = \frac{1}{2\pi R_3C_3} = \frac{1}{2\pi R_4C_4}=\frac{1}{2\pi \cdot 3\cdot 10^3\cdot 10\cdot 10^{-9}} \approx 5,3 kHz \$

The answer is letter d)

But whats about the others resistances the circuit? and when you use the same filter in series the simple question is: the frequency response has the same value when you use only 1 low-pass filter?

I tried to simulate this circuit and figure out that the frequency cut-off ( i guess) is \$0.79kHz\$ e \$4.18kHz\$

SIMULATED FREQUENCY RESPONSE

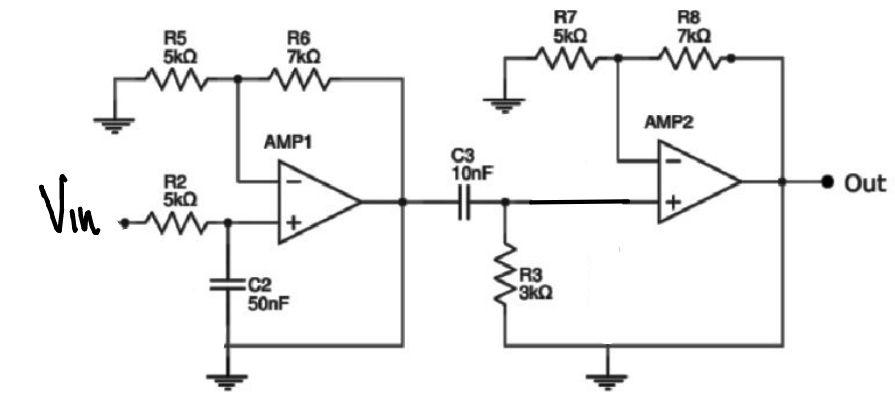
I presumed that the correct schematic this circuit to match the same \$ f_c\$ as the question required must be such as the imagem below:

So, anyone has any advice to solve the entire circuit and provided a decent analysis to this circuit? thanks for any hints.

Answer 1

But whats about the others resistances the circuit?

The gain setting resistors (R5, R6, R7 and R8) do two things: -

• Set the pass-band gain
• Modify the Q of the circuit enabling a peak in the frequency response around the cut-off frequency of each stage.

when you use the same filter in series the simple question is: the frequency response has the same value when you use only 1 low-pass filter?

With equal values for R1 and R2 AND C1 and C2 then the cut-off frequency is the same for a single order low pass filter. The big difference in your circuits (Sallen Key, 2nd order filters) is that any signals that are outside the pass-band are attenuated to a greater extent.

If you are asking about the change in the 3 dB point of the low-pass filter when you introduce the high-pass filter then sure, there'll be some interaction and that interaction gets worse as the two cut-off frequencies get closer. With the two cut-offs at 637 Hz and 5.3 kHz, there will be some slight interaction.

Your final circuit diagram is wrong and makes no sense. You have op-amp outputs connected to ground and that is a spoiler.