Do anyone know how to derive the microstrip stub equations for all four microstrip below ?
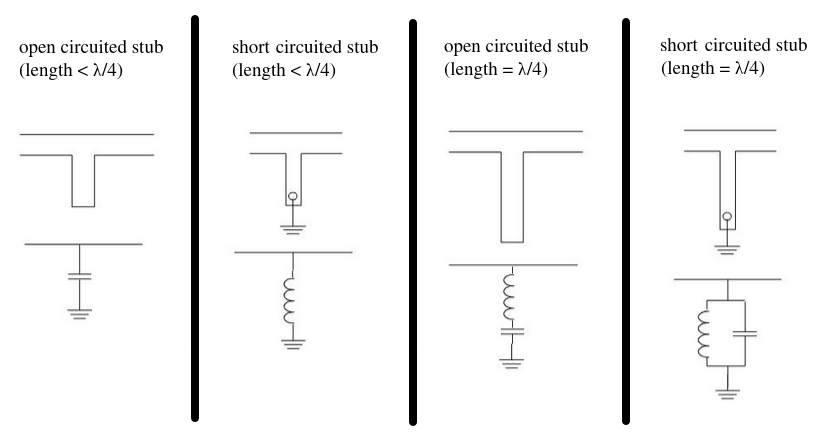
Do anyone know how to derive the microstrip stub equations for all four microstrip below ?

Stolen from: https://www.edn.com/design/test-and-measurement/4424529/So-you-think-you-understand-transmission-lines-
But anyways, that's the impedance of some transmission line that's open on the other end. You can see it decreases in impedance initially, which is the hallmark of a capacitor. Then it sharply decreases and starts going back up, which is a resonance and can be modeled by a inductor in series with the capacitor.
If you look at the impedance of a transmission line:
$$ Z_{in}(l) = Z_0 \frac {Z_L + jZ_0 \tan(\beta l)} {Z_0 + jZ_L \tan(\beta l)} $$
where \$ \beta = \frac {2 \pi} {\lambda}\$.
Try simplifying and graphing that equation when \$Z_L = 0\$ (shorted stub) and \$Z_L = \infty\$ (open stub).
You'll see the kind of behavior I described--increasing or decreasing impedance wrt frequency, and a resonance at \$l = \frac \lambda 4\$.