0
\$\begingroup\$

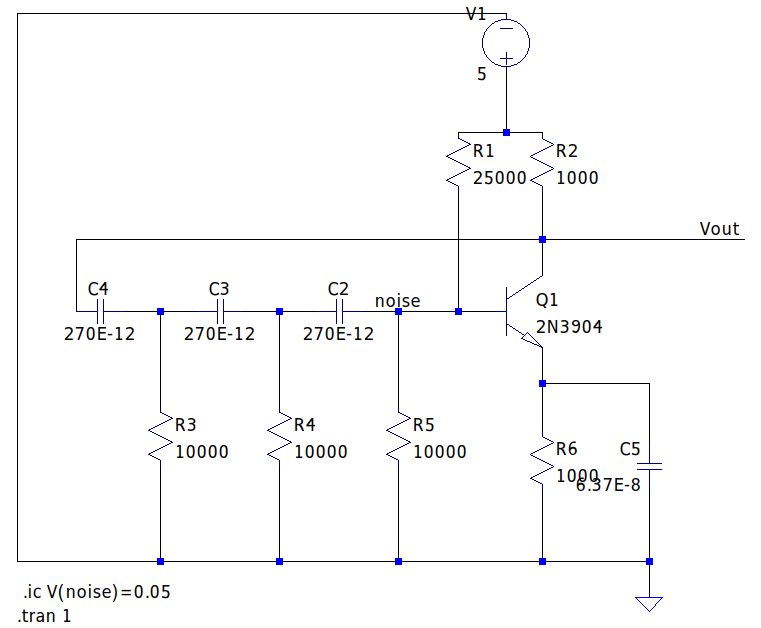
I am working with a few colleagues to build a transceiver system 100% from scratch. A necessary component here is the local oscillator system - we have opted to use a phase shift oscillator to generate our target frequencies. Below is the oscillator circuit we built, modeled through LTSpice:

enter image description here

As I understand, the frequency output should be determined according to the relation: $$f = \frac{1}{2\pi RC\sqrt{2N}}$$ Where N is the number of phase-shift networks and R,C are the values of resistance and capacitance in these networks, respectively.

Based on these assumptions, the above circuit should have N=3, R=10,000, & C=270E-12 - the sinusoidal output is expected to be very near 24KHz. However, both the simulation and actualized circuits are producing a frequency double this, in the vicinity of 47-50KHz. Have I made a poor assumption? Have I overlooked something simple? Why is the actual frequency output double my expected value?

\$\endgroup\$
5
  • \$\begingroup\$ Your oscillator may be more reliable if you use a low-pass network instead of a high-pass one. That's probably not what's causing your current difficulty, though. \$\endgroup\$
    – TimWescott
    Commented Oct 23, 2019 at 0:48
  • \$\begingroup\$ you will have high phase noise. Will that effect the usefulness of your transceiver? \$\endgroup\$
    – analogsystemsrf
    Commented Oct 23, 2019 at 4:14
  • \$\begingroup\$ @TimWescott An interesting suggestion; under what circumstances would you expect a lowpass network-based oscillator to be more stable? \$\endgroup\$
    – OPET
    Commented Oct 23, 2019 at 8:22
  • \$\begingroup\$ @analogsystemsrf Almost certainly! I expect noise figure calculations to be the main concern in the next iteration. For now, my computer engineers are eager to begin flushing out their communications protocols, so I've opted to throw together a "quick and dirty" 4QAM channel across a handful of subcarriers - an insufficient bitrate for later data transfers, but enough to work through basic handshakes and such. I (optimistically) expect our receiver to distinguish between 2 distinct phase states in the presence of this noise for now \$\endgroup\$
    – OPET
    Commented Oct 23, 2019 at 8:28
  • \$\begingroup\$ It's just that circuits are less predictable at high frequencies, with more parasitic components having an effect. A low-pass network just naturally cuts off the possibility of oscillation or noise amplification at high frequencies. \$\endgroup\$
    – TimWescott
    Commented Oct 23, 2019 at 13:57

1 Answer 1

Reset to default
0
\$\begingroup\$

You have ignored the effect of R1 and the input resistance of Q1. The parallel combination of R1, R5, and Rpi should equal R. In this case a lower value of R will help make this possible.

The formula for Fo is too simple to be believable. You don't provide a source or derivation, but I think it could only apply to N independent phase shift networks and here the three RC elements interact. The first is affected by the loading of the other two, the second is affected by the source impedance of the first and the loading of the third, etc. To create a correct formula you would need to derive the third order equation for this network and solve for F where the phase shift is 180 degrees.

\$\endgroup\$
3
  • \$\begingroup\$ Sounds like I will need to dig up my copy of Ravazi's Microelectronics! I was foolish to neglect input resistance, time to get back to the fundamentals. Although, combining the three resistors in parallel as suggested would only explain the oscillator's behavior if input resistance is unrealistically high (>1kOhm) - I imagine there is more to this mystery \$\endgroup\$
    – OPET
    Commented Oct 23, 2019 at 8:32
  • \$\begingroup\$ Your formula for Fo is not correct either. I have added the reason to the answer. \$\endgroup\$
    – EinarA
    Commented Oct 23, 2019 at 21:08
  • \$\begingroup\$ Also; I would have instinctively used the low pass version here with out thinking of the reasons, which is why l did not suggest it. \$\endgroup\$
    – EinarA
    Commented Oct 23, 2019 at 21:18

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.