Defintion of equivalent circuit

Question

I'm new in electronic and I want to know the exact definition of equivlant circuit. My trouble is that we know for example that a resistance equivalence has same current of the resistances in series for a same tension. If we substitute the resistance in series with the equivalent resistance we obtain another circuit and then we calculate the tension in to the poles of the equivlent resistance. At this point we use this voltage in original circuit, but how we know that in the orginal circuit the tension is the same? I don't see explanation maybe it follows from linearity but textbooks I had read don't say anything about this question.

Update

Suppose we have a circuit C and a subcircuit A and let be K the complement circuit of A in C so C=K+A. Now I say that A' is equivalent to A if we consider C'=K+A' calculate the currents and voltages of K in this case and they are the same of K in the original circuit C. If my definition is correct then we must proof that proposition in all cases we want to show an equivlant circuit such as equivalent resistance. Correct me if I'm wrong. I gave that definition because it is what we do in exercises.

New update

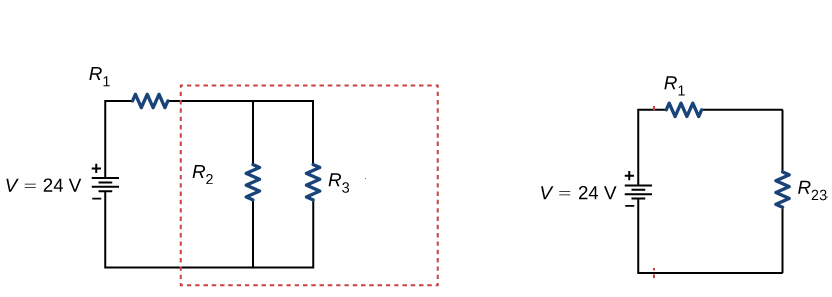
I have these two circuits:

I solve the second circuit and I find the voltage on R23, then usually in exercises I use that voltage in first circuit to compute current in R2 and R3 in first circuit. My question is: why the volatage in the second circuit can be used for the first (I want a rigorous proof)? Is there a theorem concerning equivalent circuits (in this case R23 is quivalent of R2, R3 in parallel) embedded in other circuits?

Answer 1

Equivalence of circuits means that if each was hidden in a black box (no other information from inside) with only their terminals exposed you could not discriminate between them; no matter what you connect to the terminals.

If you provide a certain voltage to the terminals the current through the terminals will be the same and and vice versa, i.e. if you let flow a certain current through the terminals the voltage across the terminals will be the same for both circuits if they are equivalent.

Note: It does not mean, however, that they would dissipate the same amount of power, i.e. dissipated heat must also be thought to be hidden inside the black box (e.g. a Norton source and a Thevenin source may be equivalent, but in open circuit configuration only the Norton source would create heat; the Thevenin source not).

If it is known that two circuits are linear circuits (with 2 terminals) there are some simple criteria to determine whether they are equivalent or not:

• E.g.: If their open circuit voltage and their short circuit current are both equal then the two linear circuits are equivalent.
• Or more general: if their V-I-characteristics (which are V-I-lines) have two points in common then they are equivalent.