Equivalent circuit and substitution

Question

Two circuits are equivalent if you provide a certain voltage to the terminals the current through the terminals will be the same and and vice versa; i.e. if you let flow a certain current through the terminals, the voltage across the terminals will be the same for both circuits.

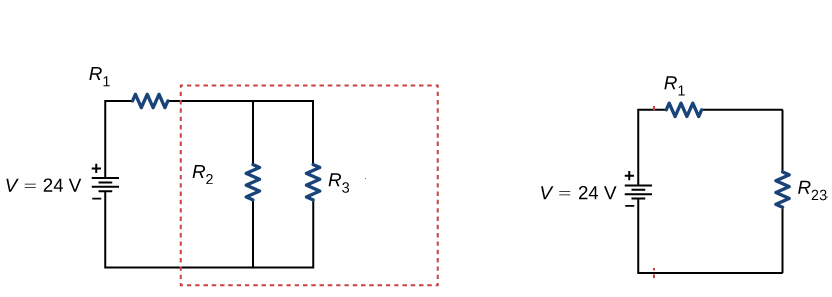
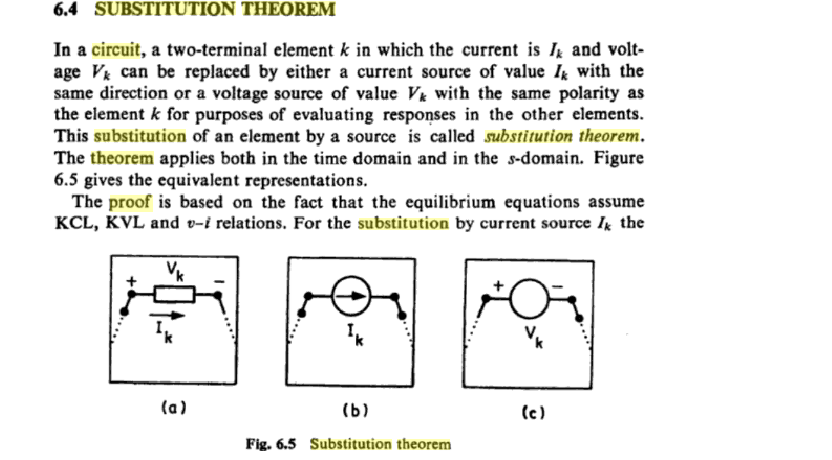
Now, I have these two circuits:

I solve the second circuit and I find the voltage on R23, then usually in exercises I use that voltage in first circuit to compute current in R2 and R3 in first circuit. My question is: why the voltage in the second circuit can be used for the first (I want a rigorous proof)? Is there a theorem concerning equivalent circuits (in this case R23 is equivalent of R2, R3 in parallel) embedded in other circuits?

Important: I gave that picture only for example, I want a proof that it is true in general. I want to prove that if I have two equivalent circuits A, B respect to a pair of terminals and I plug them to another circuit C so we have C+A and C+B, plug using the terminal in which A and B are equivalent, then the voltage on A is equals on B in both the circuits on the terminals.

In substance starting from my definition of equivalent circuit I would like prove that: Given A and B equivalent in my definition we have that the voltage across the terminals and current flowing through terminals will be the same, when placed in the same context.

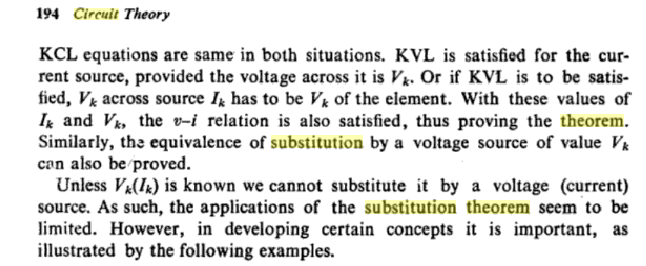
Update To give an example for proof I would like to see, we can take the substitution theorem which similar but not equal to my question (as you can see this theorem seems obvious and has a rigorous proof)

Taken from Circuit Theory T. S. K. V. Iyer

I have insert this update only to say that my question seems obvious like substitution theorem and as you can see substitution theorem has an detailed proof and I think that my question maybe should have similiar proof in which are involved considerations on KCL, KVL and v-i equations. In my question KCL, KVL and v-i equations are not exactly equals in C+A and C+B (like in substitutions theorem) but equals in C and more we have that A is equivalent to B (in sense of my definition).

Answer 1

I don't know what a "rigorous proof" would be, but consider that you combined R2 and R3 because they are parallel. By definition, elements in parallel have the same voltage across them, and as you observe the equivalent resistor must also have the same voltage across it. So, if you find the voltage across R23 then you have also found the voltage across R2 and R3. This is because of how the terms parallel and equivalent are defined in this context.

Based on OP's edits: When you combine elements in parallel the voltage across them is the same by definition, and is the same across the equivalent resistor that replaces them, but the current through them may be different. When you combine elements in series the voltage across them may be different but the current through them is the same by definition, and is the same through the equivalent resistor that replaces them.

This is inherent in the definition of parallel and series. I don't know how they would be proved more rigorously.

Answer 2

Well comments are enough to answer your query already but still I'll put it in the words you want using your network A, Network B terminology.

By your definition of equivalency you're just stating that, loosely speaking equivalent resistance of A (say Ra) and B(say Rb) is same i. e Ra=Rb(then & then only your definition would be satisfied) (technically by your definition of equivalency you're saying that input impedance of both networks are equal).

Now since Ra=Rb and resistance of your network C, Say Rc is fixed, applying voltage divider rule, of course and always,

V12= Rb/(Rb+Rc) = Ra/(Ra+Rc) = V1=V2.

I hope you understand how you're stating what's quite obvious.