Why is Frequency Conversion Additive in Acoustics Beating, but Multiplicative in Heterodyning?

Question

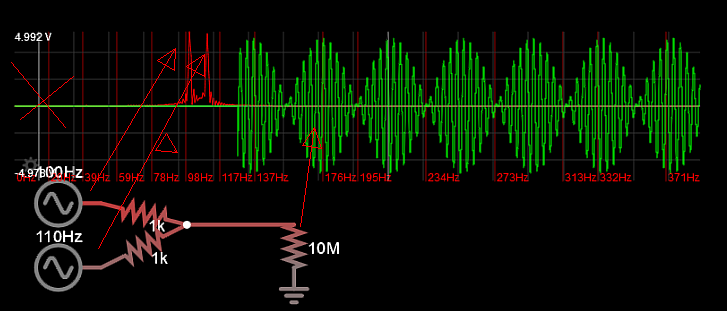
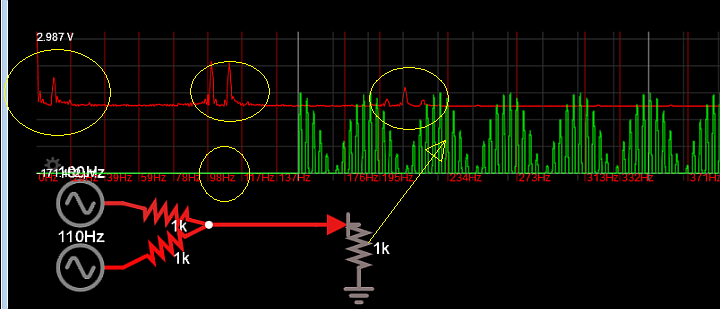
In radio electronics, one of the first concept to learn is frequency conversion by using a mixer. And it's strongly emphasized that the mixer is a multiplier, not an adder used in audio circuitry. The description is usually something like the following...

Heterodyning

Adding or summing two sine waves of different frequencies (f1 and f2) combines their amplitudes without affecting their frequencies. Viewed with an oscilloscope, adding two signals appears as a simple superimposition of one signal on the other. Viewed with a spectrum analyzer, adding two signals just sums their spectra.

Multiplying two sine waves of different frequencies produces a new output spectrum. Viewed with an oscilloscope, the result of multiplying two signals is a composite wave that seems to have little in common with its components. A spectrum-analyzer view of the same wave reveals why: The original signals disappear entirely and are replaced by two new signals — at the sum and difference of the original signals’ frequencies. - ARRL Handbook for Radio Communication

And we have,

$$ \sin (2 \pi f_1 t)\sin (2 \pi f_2 t) = \frac{1}{2}\cos [2 \pi (f_1 - f_2) t] - \frac{1}{2}\cos [2 \pi (f_1 + f_2) t] \ $$

Most books also emphasize that

Frequency conversion (or heterodyning, or amplitude modulation) is impossible in a LTI system.

---

These made perfect sense to me, until I revisit the description of acoustic beats and beating frequency in most physics textbooks... It is said that if you add two sound wave together, the result is an amplitude modulated sound wave due to constructive and destructive interference of waves.

Beating

Suppose [...] that we have two waves, [...] From one source, let us say, we would have \$ \cos{\omega_{1} t} \$, and from the other source, \$ \cos{\omega_{2} t} \$, where the two ω’s are not exactly the same. [...] Let us first take the case where the amplitudes are equal. Then the total amplitude at P is the sum of these two cosines. If we plot the amplitudes of the waves against the time, [...] we see that where the crests coincide we get a strong wave, and where a trough and crest coincide we get practically zero, and then when the crests coincide again we get a strong wave again.

On this basis one could say that the amplitude varies at the frequency \$ \frac{1}{2}(\omega_1−\omega_2) \$, but if we are talking about the intensity of the wave we must think of it as having twice this frequency. That is, the modulation of the amplitude, in the sense of the strength of its intensity, is at frequency \$ \omega_{1}-\omega_{2} \$ - The Feynman Lectures on Physics

And we have,

$$ { \cos(2\pi f_1t)+\cos(2\pi f_2t) } = { 2\cos\left(2\pi\frac{f_1+f_2}{2}t\right)\cos\left(2\pi\frac{f_1-f_2}{2}t\right) } $$

---

Why is this even possible in the case of beating?

I initially suspect this is the result of being a non-LTI system, the system somehow contains a time-varying element, but I cannot identify it.

On my second thought, it seems that \$ \frac{1}{2}(\omega_1−\omega_2) \$ (or \$ \omega_{1}-\omega_{2} \$) is only a human perception of the change in intensity of two frequencies, and it's not an actual frequency component created by a modulation process, is it the correct interpretation?

Answer 1

I initially suspect this is the result of being a non-LTI system, the system somehow contains a time-varying element, but I cannot identify it.

On my second thought, it seems that [it] is only a human perception of the change in intensity of two frequencies, and it's not an actual frequency component created by a modulation process

If you consider the human ear as a part of the system then a modulation process is involved. If it wasn't we would not be able to hear the 'beat' frequency.

In a perfectly linear medium any number of waves can coexist independently, and a time-invariant system is insensitive to instantaneous amplitude so 'beats' have no effect. But the human ear - like an rf 'mixer' or 'detector' - is nonlinear.

The generic definition of 'mixer' is 'a machine used for mixing things together'. The mixing process may combine those things to make a different product (eg. cake mixer) or simply so they can be transported together (eg. mixed sweets). In audio equipment a 'mixer' is the name for a circuit that combines signals without distorting them - ie. an adder. In rf design it is the name for a circuit which combines signals with distortion, ie. a multiplier.

Answer 2

I've searched for a while, and my own conclusion is...

The word "beat" is as ambiguous as "mixer". It can refer to audible change of amplitude (not frequency) of a signal, in this case, the change in amplitude of the wave is completely due to constructive and destructive interference in an addition process, not a result of carrying information or frequency conversion. Although the amplitude varies periodically, no new frequency component is created, which means there is no amplitude modulation or sideband. Many books refer to the phenomenon of the changing amplitude as "modulation", which made the situation more confusing, since there's no information in the signal at all and there can't be any "modulation".

The same word "beat" can also refer to an auditory illusion "binaural beats" in human perception. In this case, when two tones of different frequencies are heard by two ears, somehow the brain perceives a new frequency (possibly due to some non-linearity in the nervous system), as if a new frequency is created by a frequency mixer, but there's no such frequency.

In either case of "beating", no new frequency component is created.

Recall

$$ { \cos(2\pi f_1t)+\cos(2\pi f_2t) } = { 2\cos\left(2\pi\frac{f_1+f_2}{2}t\right)\cos\left(2\pi\frac{f_1-f_2}{2}t\right) } $$

At the right side of this equation, there is no plus or minus sign.

The term \$ 2\cos\left(2\pi\frac{f_1+f_2}{2}t\right)\cos\left(2\pi\frac{f_1-f_2}{2}t\right) \$ is just an alternative way to write down the additive combination of two frequencies.

On the other hand, the periodic variation of a real amplitude modulated or frequency converted signal is the result of a multiplicative process.

$$ \sin (2 \pi f_1 t)\sin (2 \pi f_2 t) = \frac{1}{2}\cos [2 \pi (f_1 - f_2) t] - \frac{1}{2}\cos [2 \pi (f_1 + f_2) t] \ $$

Here, \$ (f_1 - f_2) \$ and \$ (f_1 + f_2) \$ are two new frequencies we created.

Answer 3

I initially suspect this is the result of being a non-LTI system, the system somehow contains a time-varying element, but I cannot identify it.

Close, it contains a nonlinear operation:

if we are talking about the intensity of the wave we must think of it as having twice this frequency

Intensity is X^2, and X^2 is very much nonlinear. Incidentally, this is why interferometry works. Cameras detect intensity, which is the square of amplitude, so a photograph of two beams interfering records the beat frequency between them rather than the original waves.

Answer 4

In beating the resulting frequency spectrum has still the frequency components \$f_1\$ and \$f_2\$;
not \$f_1 - f_2\$ and/or \$f_1 + f_2\$.

So your statement

Here, \$(f_1−f_2)\$ and \$(f_1+f_2)\$ are two new frequencies we created.

is simply not true; at least not in the sense that those two frequency make up the spectrum of the beating sound.

So there is also nothing to wonder about.

Mixing (hetrodyning) and beating are quite different operations; the first is non-linear, the latter is linear.

They have also a very different effect. You can not use beating for frequency conversion, as you claimed.
E.g. you could not use beating to make ultrasound hearable by the human ear (as long as not any non-linear operations are involved).

Answer 5

AM

When two similar but different audio tones are added in a linear system, the result is a amplitude modulation (AM) of the two frequencies and not the actual difference frequency.

There is no difference frequency in the spectrum!

This was popular by wet-rubbing the rim of crystal wine glasses which had different resonant frequencies closely matched and thus made a beating or alias-like sound of a slow wow-wow audio modulation, as the phases shift in the resonance.

For audio we call a linear addition, a mixer unlike for non-linear systems.

Below is 100 , 110Hz of exactly the same amplitude showing a 10 Hz AM apparent beat.

But it is NOT AM. It just appears and sounds similar to it.

The spectrum is only f1, f2

---

Frequency Mixing a.k.a Intermodulation

(aka sum and difference product freq.)

For non-linear systems we use a mixer intentionally to produce sum and difference frequencies and choose the one we need.

e.g. XOR gate in a PLL is a phase detector or frequency mixer.
A double-balanced mixer is an example of a linear mixer that multiplies the signals to produce +/- f.

The spectrum is f1, f2, f1-f2 , f1+f2 ...