0
\$\begingroup\$

In the following circuit:

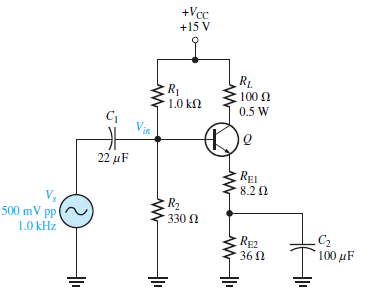
enter image description here

I calculated the base voltage by Vb = R2/(R1+R2) Vcc [stiffed voltage divider] , but then when I'm asked to calculate the total power from the power supply when we remove the load, the base voltage changes.

My question is why does the base voltage change after removing R_L and how do I calculate it? This is how the solution calculates it and this is what it says:

enter image description here

I don't understand how Thevnin's theorm is used here and why is it even used

\$\endgroup\$
10
  • \$\begingroup\$ Do you need a more thoroughly descriptive answer than what Cristobol provided? What he wrote is correct. But it may be assuming that you understand things you may not actually understand. Another way of putting this is: When you remove the load, there cannot be any collector current (obviously.) So now the emitter current equals the base current (which isn't true when the load is connected.) So the whole situation suddenly changes. \$\endgroup\$
    – jonk
    Commented Feb 26, 2020 at 23:48
  • \$\begingroup\$ I understood what he meant yes, but I want to make sure I understand it correctly, if the emitter current is now the base current, does that affect the voltage divider because the current of the voltage divider is the same as the current that flows through the emitter resistors now? \$\endgroup\$
    – khaled014z
    Commented Feb 27, 2020 at 0:05
  • \$\begingroup\$ The base current flows through those two DC resistors. Of course, you need Thevenin's for the base resistor pair to make things easier. Then you have an equivalent ideal voltage source along with an equivalent series resistance, then a diode, then the two emitter resistors to ground. So you can just sum up all that resistance, leaving just the Thevenin source voltage, one resistor, and one diode. Subtract the diode voltage from the Thevenin source voltage and that's the voltage that must be dropped across that remaining resistor. So you know the current (approximately, without Shockley eq.) \$\endgroup\$
    – jonk
    Commented Feb 27, 2020 at 0:11
  • \$\begingroup\$ What I did was, I have the thevenin voltage with the thevenin resistance that comes from R1 and R2 , in series with a diode and the two emitter resistors, so now I used voltage divider to get the voltage across the two emitter resistors, then I added 0.7V to it to get the base voltage ,is that correct? \$\endgroup\$
    – khaled014z
    Commented Feb 27, 2020 at 0:16
  • \$\begingroup\$ Also just to make sure I understand what Cristobol said, when the only current flowing through the emitter resistors is the base current, this means that the emitter resistors have to draw more voltage from the voltage divider because the current decreased, no? \$\endgroup\$
    – khaled014z
    Commented Feb 27, 2020 at 0:19

2 Answers 2

Reset to default
1
\$\begingroup\$

When \$R_L\$ is connected, \$R_{E1}\$ and \$R_{E2}\$ are fed predominantly by the collector current. The base provides only a small fraction \$1/(\beta+1)\$ of that current. In this case, the base voltage is primarily determined by \$R_1\$ and \$R_2\$.

When you disconnect \$R_L\$, there is no source for current to \$R_{E1}\$ and \$R_{E2}\$ except from the base. This draws significant current from the voltage divider on 15V comprised of \$R_1\$ and \$R_2\$. This is represented by its Thevenin equivalent so that the network can be analyzed as a series circuit. The transistor, with no collector connection, is essentially a diode.

\$\endgroup\$
0
0
\$\begingroup\$

Well, we have the following circuit:

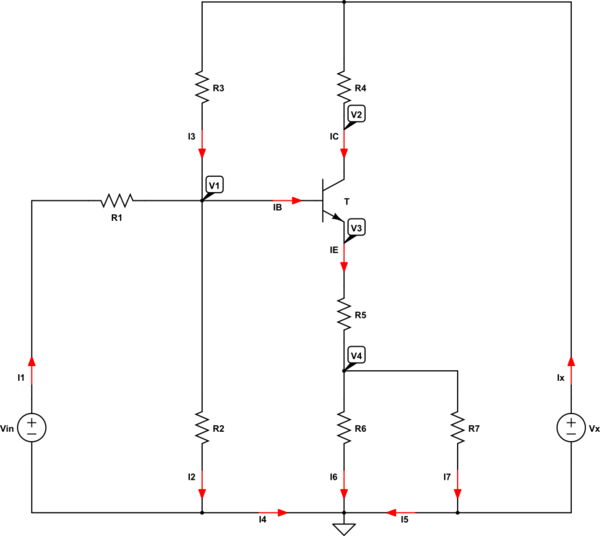
schematic

simulate this circuit – Schematic created using CircuitLab

When analyzing a transistor we need to use the following relations:

  • $$\text{I}_\text{E}=\text{I}_\text{B}+\text{I}_\text{C}\tag1$$
  • Transistor gain \$\beta\$: $$\beta=\frac{\text{I}_\text{C}}{\text{I}_\text{B}}\tag2$$
  • Emitter voltage: $$\text{V}_\text{E}=\text{V}_1-\text{V}_3\tag3$$

When we use and apply KCL, we can write the following set of equations:

$$ \begin{cases} \text{I}_1+\text{I}_3=\text{I}_\text{B}+\text{I}_2\\ \\ \text{I}_2=\text{I}_1+\text{I}_4\\ \\ \text{I}_\text{x}=\text{I}_\text{C}+\text{I}_3\\ \\ \text{I}_\text{E}=\text{I}_6+\text{I}_7\\ \\ 0=\text{I}_4+\text{I}_5+\text{I}_6\\ \\ \text{I}_7=\text{I}_\text{x}+\text{I}_5 \end{cases}\tag4 $$

When we use and apply KVL, we can write the following set of equations:

$$ \begin{cases} \text{I}_1=\frac{\text{V}_\text{in}-\text{V}_1}{\text{R}_1}\\ \\ \text{I}_2=\frac{\text{V}_1}{\text{R}_2}\\ \\ \text{I}_3=\frac{\text{V}_\text{x}-\text{V}_1}{\text{R}_3}\\ \\ \text{I}_\text{C}=\frac{\text{V}_\text{x}-\text{V}_2}{\text{R}_4}\\ \\ \text{I}_\text{E}=\frac{\text{V}_3-\text{V}_4}{\text{R}_5}\\ \\ \text{I}_6=\frac{\text{V}_4}{\text{R}_6}\\ \\ \text{I}_7=\frac{\text{V}_4}{\text{R}_7} \end{cases}\tag5 $$

When applying the set of equations to your circuit, I used Mathematica-code to solve for the unknowns:

FullSimplify[
 Solve[{VE == V1 - V3, IE == IB + IC, β == IC/IB, 
   I1 + I3 == IB + I2, I2 == I1 + I4, Ix == IC + I3, IE == I6 + I7, 
   I4 + I5 + I6 == 0, I7 == Ix + I5, I1 == (Vin - V1)/R1, 
   I2 == (V1)/R2, I3 == (Vx - V1)/R3, IC == (Vx - V2)/R4, 
   IC == (V3 - V4)/R5, I6 == V4/R6, I7 == V4/R7}, {I1, I2, I3, I4, I5,
    I6, I7, IB, IC, IE, Ix, V1, V2, V3, V4}]]

Using that, I got the following result:

{{I1 -> (-R2 R3 (R6 + R7) (VE - Vin) + R3 R5 R7 Vin β + 
      R3 R6 Vin (R7 + (R5 + R7) β) + 
      R2 (Vin - Vx) (R5 R7 β + 
         R6 (R7 + (R5 + R7) β)))/(R1 R2 R3 R6 + R1 R2 R3 R7 + 
      R1 R2 R6 R7 + R1 R3 R6 R7 + 
      R2 R3 R6 R7 + (R2 R3 + R1 (R2 + R3)) (R6 R7 + 
         R5 (R6 + R7)) β), 
  I2 -> (R1 R3 R6 VE + R1 R3 R7 VE + R3 R6 R7 Vin + 
      R1 R6 R7 Vx + (R6 R7 + R5 (R6 + R7)) (R3 Vin + 
         R1 Vx) β)/(R1 R2 R3 R6 + R1 R2 R3 R7 + R1 R2 R6 R7 + 
      R1 R3 R6 R7 + 
      R2 R3 R6 R7 + (R2 R3 + R1 (R2 + R3)) (R6 R7 + 
         R5 (R6 + R7)) β), 
  I3 -> (-R2 (Vin - Vx) (R5 R7 β + 
         R6 (R7 + (R5 + R7) β)) + 
      R1 (-R2 (R6 + R7) (VE - Vx) + R5 R7 Vx β + 
         R6 Vx (R7 + (R5 + R7) β)))/(R1 R2 R3 R6 + R1 R2 R3 R7 +
       R1 R2 R6 R7 + R1 R3 R6 R7 + 
      R2 R3 R6 R7 + (R2 R3 + R1 (R2 + R3)) (R6 R7 + 
         R5 (R6 + R7)) β), 
  I4 -> (R1 R3 (R6 + R7) VE + R2 R3 (R6 + R7) (VE - Vin) + 
      R1 R5 R7 Vx β + R1 R6 Vx (R7 + (R5 + R7) β) - 
      R2 (Vin - Vx) (R5 R7 β + 
         R6 (R7 + (R5 + R7) β)))/(R1 R2 R3 R6 + R1 R2 R3 R7 + 
      R1 R2 R6 R7 + R1 R3 R6 R7 + 
      R2 R3 R6 R7 + (R2 R3 + R1 (R2 + R3)) (R6 R7 + 
         R5 (R6 + R7)) β), 
  I5 -> (-R1 (R5 R7 Vx β - R2 R7 (VE - Vx) (1 + β) + 
         R3 VE (R6 - R7 β) + R6 Vx (R7 + (R5 + R7) β)) + 
      R2 (-R3 (VE - Vin) (R6 - R7 β) + (Vin - 
            Vx) (R5 R7 β + 
            R6 (R7 + (R5 + R7) β))))/(R1 R2 R3 R6 + 
      R1 R2 R3 R7 + R1 R2 R6 R7 + R1 R3 R6 R7 + 
      R2 R3 R6 R7 + (R2 R3 + R1 (R2 + R3)) (R6 R7 + 
         R5 (R6 + R7)) β), 
  I6 -> -((R7 (R1 (R2 + R3) VE + R2 R3 (VE - Vin) - 
          R1 R2 Vx) (1 + β))/(R1 R2 R3 R6 + R1 R2 R3 R7 + 
        R1 R2 R6 R7 + R1 R3 R6 R7 + 
        R2 R3 R6 R7 + (R2 R3 + R1 (R2 + R3)) (R6 R7 + 
           R5 (R6 + R7)) β)), 
  I7 -> -((R6 (R1 (R2 + R3) VE + R2 R3 (VE - Vin) - 
          R1 R2 Vx) (1 + β))/(R1 R2 R3 R6 + R1 R2 R3 R7 + 
        R1 R2 R6 R7 + R1 R3 R6 R7 + 
        R2 R3 R6 R7 + (R2 R3 + R1 (R2 + R3)) (R6 R7 + 
           R5 (R6 + R7)) β)), 
  IB -> -(((R6 + R7) (R1 (R2 + R3) VE + R2 R3 (VE - Vin) - 
          R1 R2 Vx))/(R1 R2 R3 R6 + R1 R2 R3 R7 + R1 R2 R6 R7 + 
        R1 R3 R6 R7 + 
        R2 R3 R6 R7 + (R2 R3 + R1 (R2 + R3)) (R6 R7 + 
           R5 (R6 + R7)) β)), 
  IC -> -(((R6 + R7) (R1 (R2 + R3) VE + R2 R3 (VE - Vin) - 
          R1 R2 Vx) β)/(R1 R2 R3 R6 + R1 R2 R3 R7 + 
        R1 R2 R6 R7 + R1 R3 R6 R7 + 
        R2 R3 R6 R7 + (R2 R3 + R1 (R2 + R3)) (R6 R7 + 
           R5 (R6 + R7)) β)), 
  IE -> -(((R6 + R7) (R1 (R2 + R3) VE + R2 R3 (VE - Vin) - 
          R1 R2 Vx) (1 + β))/(R1 R2 R3 R6 + R1 R2 R3 R7 + 
        R1 R2 R6 R7 + R1 R3 R6 R7 + 
        R2 R3 R6 R7 + (R2 R3 + R1 (R2 + R3)) (R6 R7 + 
           R5 (R6 + R7)) β)), 
  Ix -> (R2 R6 R7 (-Vin + Vx) + 
      R2 (-R3 (R6 + R7) (VE - Vin) - (R5 R6 + (R5 + R6) R7) (Vin - 
            Vx)) β + 
      R1 (R6 R7 Vx + (-R3 (R6 + R7) VE + 
            R5 R6 Vx + (R5 + R6) R7 Vx) β - 
         R2 (R6 + R7) (VE - Vx) (1 + β)))/(R1 R2 R3 R6 + 
      R1 R2 R3 R7 + R1 R2 R6 R7 + R1 R3 R6 R7 + 
      R2 R3 R6 R7 + (R2 R3 + R1 (R2 + R3)) (R6 R7 + 
         R5 (R6 + R7)) β), 
  V1 -> (R2 (R1 R3 R6 VE + R1 R3 R7 VE + R3 R6 R7 Vin + 
        R1 R6 R7 Vx + (R6 R7 + R5 (R6 + R7)) (R3 Vin + 
           R1 Vx) β))/(R1 R2 R3 R6 + R1 R2 R3 R7 + R1 R2 R6 R7 +
       R1 R3 R6 R7 + 
      R2 R3 R6 R7 + (R2 R3 + R1 (R2 + R3)) (R6 R7 + 
         R5 (R6 + R7)) β), 
  V2 -> (R1 (R2 R3 R6 + R3 R6 R7 + R2 (R3 + R6) R7) Vx + 
      R1 ((R2 + R3) R4 (R6 + R7) VE + (R3 R5 R6 + R3 (R5 + R6) R7 + 
            R2 (R5 R6 + (R5 + R6) R7 - R4 (R6 + R7))) Vx) β + 
      R2 R3 (R6 R7 Vx + (R4 (R6 + R7) (VE - Vin) + R6 R7 Vx + 
            R5 (R6 + R7) Vx) β))/(R1 R2 R3 R6 + R1 R2 R3 R7 + 
      R1 R2 R6 R7 + R1 R3 R6 R7 + 
      R2 R3 R6 R7 + (R2 R3 + R1 (R2 + R3)) (R6 R7 + 
         R5 (R6 + R7)) β), 
  V3 -> -(((R1 (R2 + R3) VE + R2 R3 (VE - Vin) - 
          R1 R2 Vx) (R5 R7 β + 
          R6 (R7 + (R5 + R7) β)))/(R1 R2 R3 R6 + R1 R2 R3 R7 + 
        R1 R2 R6 R7 + R1 R3 R6 R7 + 
        R2 R3 R6 R7 + (R2 R3 + R1 (R2 + R3)) (R6 R7 + 
           R5 (R6 + R7)) β)), 
  V4 -> -((R6 R7 (R1 (R2 + R3) VE + R2 R3 (VE - Vin) - 
          R1 R2 Vx) (1 + β))/(R1 R2 R3 R6 + R1 R2 R3 R7 + 
        R1 R2 R6 R7 + R1 R3 R6 R7 + 
        R2 R3 R6 R7 + (R2 R3 + R1 (R2 + R3)) (R6 R7 + 
           R5 (R6 + R7)) β))}}

Which means that the base voltage is given by:

V1 -> (R2 (R1 R3 R6 VE + R1 R3 R7 VE + R3 R6 R7 Vin + 
            R1 R6 R7 Vx + (R6 R7 + R5 (R6 + R7)) (R3 Vin + 
               R1 Vx) β))/(R1 R2 R3 R6 + R1 R2 R3 R7 + R1 R2 R6 R7 +
           R1 R3 R6 R7 + 
          R2 R3 R6 R7 + (R2 R3 + R1 (R2 + R3)) (R6 R7 + 
             R5 (R6 + R7)) β)

So, as you can see the base voltage is independent on \$\text{R}_4\$.

Using your values, with:

  1. $$\text{R}_1=\frac{1}{\text{sC}_1}\space\space\space\Longrightarrow\space\space\space\text{R}_1=\frac{1}{\text{s}\cdot22\cdot10^{-6}}=\frac{10^6}{22\text{s}}\tag6$$
  2. $$\text{R}_7=\frac{1}{\text{sC}_2}\space\space\space\Longrightarrow\space\space\space\text{R}_7=\frac{1}{\text{s}\cdot100\cdot10^{-6}}=\frac{10^4}{\text{s}}\tag7$$
  3. $$\text{V}_\text{in}=\mathcal{L}_t\left[250\cdot10^{-3}\cdot\sin\left(2000\pi t\right)\right]_{\left(\text{s}\right)}=\frac{500\pi}{4000000\pi^2+\text{s}^2}\tag8$$
  4. $$\text{V}_\text{x}=\mathcal{L}_t\left[15\right]_{\left(\text{s}\right)}=\frac{15}{\text{s}}\tag9$$

And I used the assumptions:

  1. $$\beta=100\tag{10}$$
  2. $$\text{V}_\text{E}=\text{V}_1-\text{V}_3=\mathcal{L}_t\left[\frac{7}{10}\right]_{\left(\text{s}\right)}=\frac{1}{\text{s}}\cdot\frac{7}{10}\tag{11}$$

And the base voltage is then given by:

V1 -> (16500 (200000000 \[Pi]^2 (168850 + 117 s) + 
      50 s^2 (168850 + 117 s) + 
      11 \[Pi] s^2 (557000 + 369 s)))/(s (4000000 \[Pi]^2 + 
      s^2) (39103000000 + 3 s (78051500 + 44649 s)))

And in the time domain it is equal to:

(33 E^(-((250 (156103 + 5 Sqrt[602265097]) t)/
    44649)) (1529044609 (47 (-216366939635 + 
           8620393 Sqrt[602265097]) + 
        22 (-335461659029 + 13542139 Sqrt[602265097]) \[Pi]) + 
     20338492325690 E^((250 (156103 + 5 Sqrt[602265097]) t)/
      44649) (1529044609 + 177411483153 \[Pi]^2 + 
        287068780944 \[Pi]^4) + 
     9 \[Pi]^2 (926482189799 (-216366939635 + 
           8620393 Sqrt[602265097]) + 
        16236 \[Pi] (39103 (-4277888983991 + 
              88624051 Sqrt[602265097]) + 
           92334132 (-216366939635 + 
              8620393 Sqrt[602265097]) \[Pi])) - 
     E^((2500 Sqrt[602265097] t)/
      44649) (71865096623 (216366939635 + 
           8620393 Sqrt[
            602265097]) + \[Pi] (33638981398 (335461659029 + 
              13542139 Sqrt[602265097]) + 
           9 \[Pi] (926482189799 (216366939635 + 
                 8620393 Sqrt[602265097]) + 
              16236 \[Pi] (39103 (4277888983991 + 
                    88624051 Sqrt[602265097]) + 
                 92334132 (216366939635 + 
                    8620393 Sqrt[602265097]) \[Pi])))) + 
     1036216371871604 E^((250 (156103 + 5 Sqrt[602265097]) t)/
      44649) \[Pi] ((21780371 + 47178126 \[Pi]^2) Cos[2000 \[Pi] t] + 
        33 \[Pi] (7030003 + 11982168 \[Pi]^2) Sin[
          2000 \[Pi] t])))/(188402976703928 (1529044609 + 
     177411483153 \[Pi]^2 + 287068780944 \[Pi]^4))

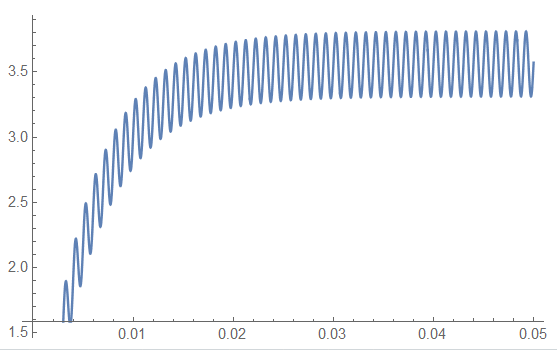
Plotting, gives:

enter image description here

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.