How to find second order transfer function from a graph?

Question

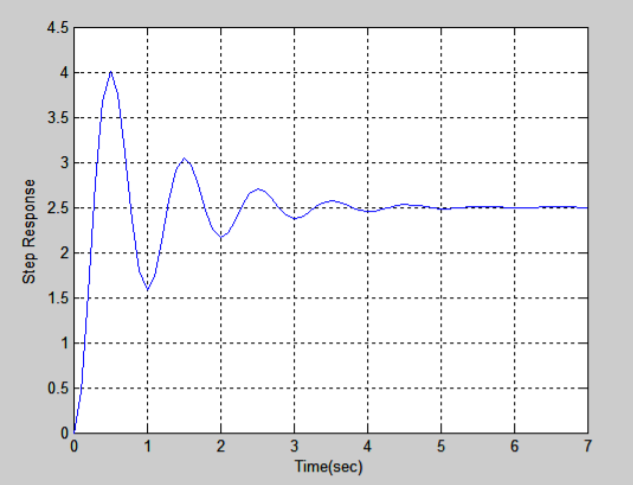
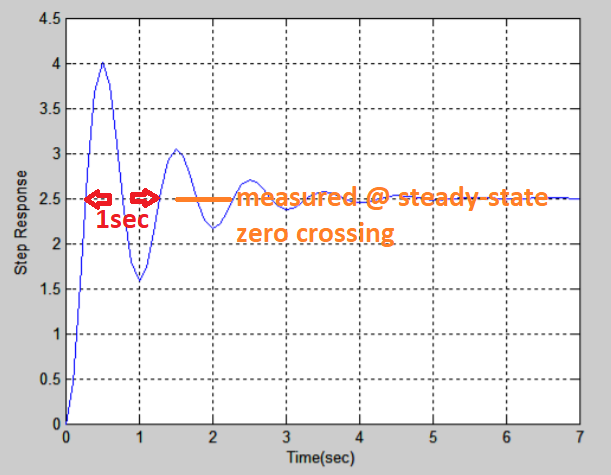
I have been given this step response graph and asked to determine the second order transfer function of the system. I completed the question, using a lot of help from this post and got the answers below:

• steady state gain = 2.5
• un-damped natural frequency = 3.18 rad/s
• damping factor = 0.16

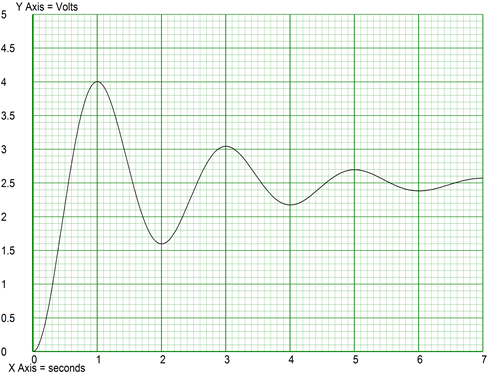
I then decided to use ServoCad to produce a graph of my transfer function, to compare against the original and check my answers. This is the graph below. The two graphs look very similar, but on my graph each oscillation appears to take double the time it should on the original graph.

I feel like this is a relatively simple mistake but I'm new to this so if anyone can point out where I've gone wrong I'd be grateful! Thanks!

Answer 1

Here is a "quickie" way of capturing \$ \omega \$ by eye. Measure the time for one cycle. Use the zero-crossing points, translated by the steady-state (+2.5 in this case) to give you a time \$t\$.
This time measurement gives you frequency \$f= 1/t\$.
Then \$ \omega = 2\pi f\$:

You can use any of the many cycles along the steady state line to measure \$t\$; they should all have the same period. The first cycle will be the largest, where you can see its steady-state crossing points easiest. Cycles following the first have less amplitude, so that estimating where they cross steady-state becomes more difficult.
:
When waveforms are quickly damped, this method is difficult to apply...it is appropriate for slowly-damped waveforms. Some folks measure time from one peak to the next. This is less accurate with a quickly-damped waveform.