First, I will present a method that uses Mathematica to solve this problem. When I was studying this stuff I used the method all the time (without using Mathematica of course).
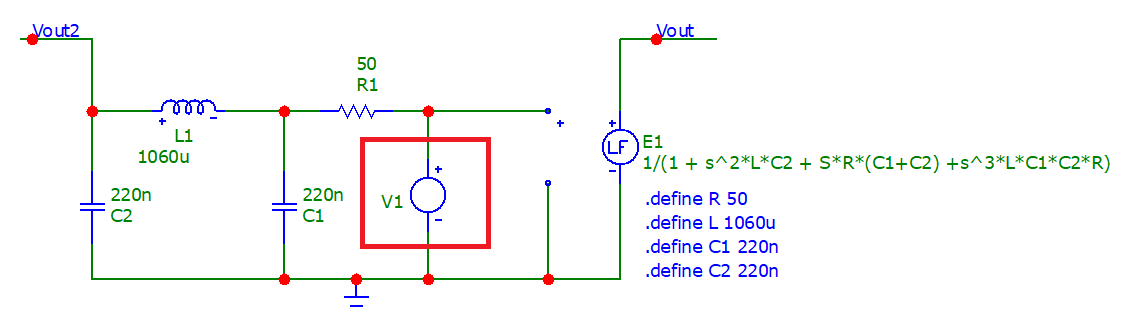
Well, we are trying to analyze the following circuit:

simulate this circuit – Schematic created using CircuitLab
When we use and apply KCL, we can write the following set of equations:
$$\text{I}_1=\text{I}_2+\text{I}_3\tag1$$
When we use and apply Ohm's law, we can write the following set of equations:
$$
\begin{cases}
\text{I}_1=\frac{\text{V}_\text{i}-\text{V}_1}{\text{R}_1}\\
\\
\text{I}_2=\frac{\text{V}_1}{\text{R}_2}\\
\\
\text{I}_3=\frac{\text{V}_1-\text{V}_2}{\text{R}_3}\\
\\
\text{I}_3=\frac{\text{V}_2}{\text{R}_4}
\end{cases}\tag2
$$
Substitute \$(2)\$ into \$(1)\$, in order to get:
$$
\begin{cases}
\frac{\text{V}_\text{i}-\text{V}_1}{\text{R}_1}=\frac{\text{V}_1}{\text{R}_2}+\frac{\text{V}_1-\text{V}_2}{\text{R}_3}\\
\\
\frac{\text{V}_\text{i}-\text{V}_1}{\text{R}_1}=\frac{\text{V}_1}{\text{R}_2}+\frac{\text{V}_2}{\text{R}_4}
\end{cases}\tag3
$$
Now, we can solve for the transfer function:
$$\mathcal{H}:=\frac{\text{V}_2}{\text{V}_\text{i}}=\frac{\text{R}_2\text{R}_4}{\text{R}_1\left(\text{R}_2+\text{R}_3+\text{R}_4\right)+\text{R}_2\left(\text{R}_3+\text{R}_4\right)}\tag4$$
Where I used the following Mathematica-code:
In[1]:=Clear["Global`*"];
FullSimplify[
Solve[{I1 == I2 + I3, I1 == (Vi - V1)/R1, I2 == V1/R2,
I3 == (V1 - V2)/R3, I3 == V2/R4}, {I1, I2, I3, V1, V2}]]
Out[1]={{I1 -> ((R2 + R3 + R4) Vi)/(R2 (R3 + R4) + R1 (R2 + R3 + R4)),
I2 -> ((R3 + R4) Vi)/(R2 (R3 + R4) + R1 (R2 + R3 + R4)),
I3 -> (R2 Vi)/(R2 (R3 + R4) + R1 (R2 + R3 + R4)),
V1 -> (R2 (R3 + R4) Vi)/(R2 (R3 + R4) + R1 (R2 + R3 + R4)),
V2 -> (R2 R4 Vi)/(R2 (R3 + R4) + R1 (R2 + R3 + R4))}}
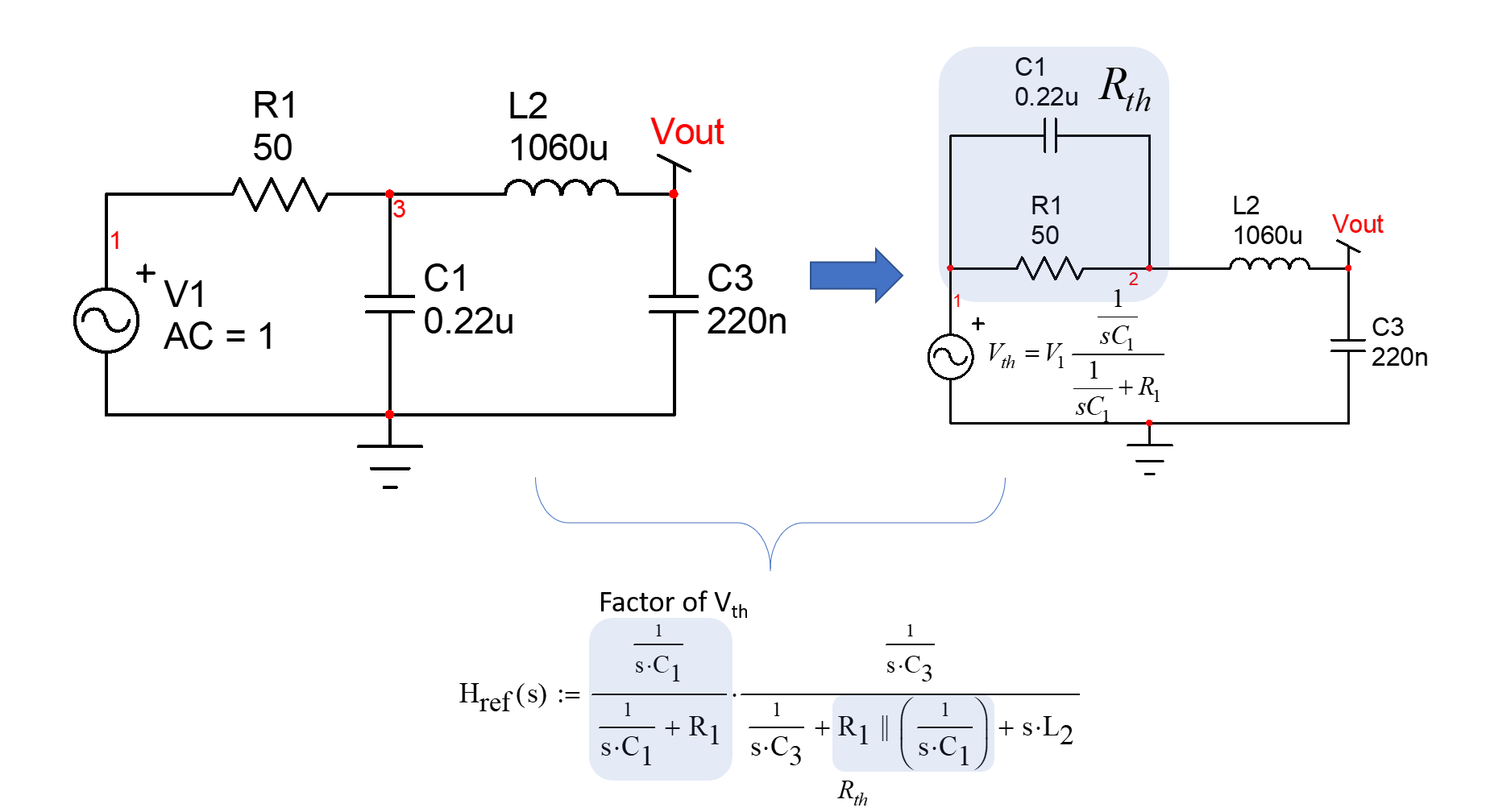
When we want to apply the derivation from above to your circuit we need to use Laplace transform (I will use lower case function names for the functions that are in the (complex) s-domain, so \$\text{y}\left(\text{s}\right)\$ is the Laplace transform of the function \$\text{Y}\left(t\right)\$):
- $$\text{R}_2=\frac{1}{\text{sC}_1}\tag5$$
- $$\text{R}_3=\text{sL}\tag6$$
- $$\text{R}_4=\frac{1}{\text{sC}_2}\tag7$$
So, we can rewrite the transfer function as:
$$\mathscr{H}\left(\text{s}\right)=\frac{\frac{1}{\text{sC}_1}\cdot\frac{1}{\text{sC}_2}}{\text{R}_1\left(\frac{1}{\text{sC}_1}+\text{sL}+\frac{1}{\text{sC}_2}\right)+\frac{1}{\text{sC}_1}\cdot\left(\text{sL}+\frac{1}{\text{sC}_2}\right)}=$$
$$\frac{1}{\text{C}_1\text{C}_2\text{L}\text{R}_1\text{s}^3+\text{C}_2\text{L}\text{s}^2+\text{R}_1\left(\text{C}_1+\text{C}_2\right)\text{s}+1}\tag8$$
Now, when working with sinusoidal signals we can use \$\text{s}:=\text{j}\omega\$ (where \$\text{j}^2=-1\$ and \$\omega=2\pi\text{f}\$ with \$\text{f}\$ is the frequency of the input signal in Hertz). So we get:
$$\underline{\mathscr{H}}\left(\text{j}\omega\right)=\frac{1}{\text{C}_1\text{C}_2\text{L}\text{R}_1\left(\text{j}\omega\right)^3+\text{C}_2\text{L}\left(\text{j}\omega\right)^2+\text{R}_1\left(\text{C}_1+\text{C}_2\right)\text{j}\omega+1}=$$
$$\frac{1}{-\text{C}_1\text{C}_2\text{L}\text{R}_1\omega^3\text{j}-\text{C}_2\text{L}\omega^2+\text{R}_1\left(\text{C}_1+\text{C}_2\right)\omega\text{j}+1}=$$
$$\frac{1}{1-\text{C}_2\text{L}\omega^2+\text{R}_1\omega\left(\text{C}_1+\text{C}_2\left(1-\text{C}_1\text{L}\omega^2\right)\right)\text{j}}\tag9$$
So, the absolute value of the transfer function is given by:
$$\left|\underline{\mathscr{H}}\left(\text{j}\omega\right)\right|=\frac{1}{\sqrt{\left(1-\text{C}_2\text{L}\omega^2\right)^2+\left(\text{R}_1\omega\left(\text{C}_1+\text{C}_2\left(1-\text{C}_1\text{L}\omega^2\right)\right)\right)^2}}\tag{10}$$
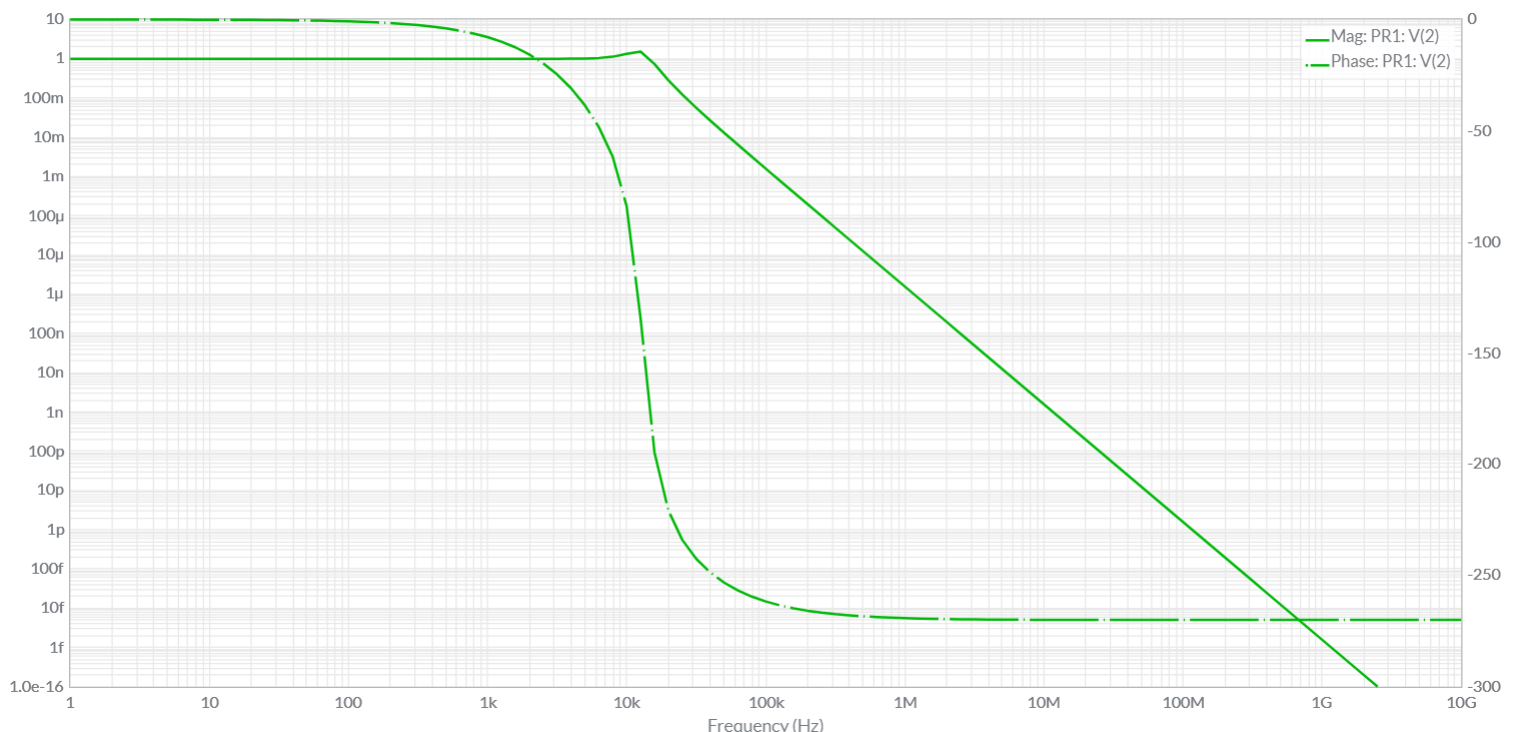 Can anyone help me derive the transfer function of the system using these Bode plots?
Can anyone help me derive the transfer function of the system using these Bode plots?

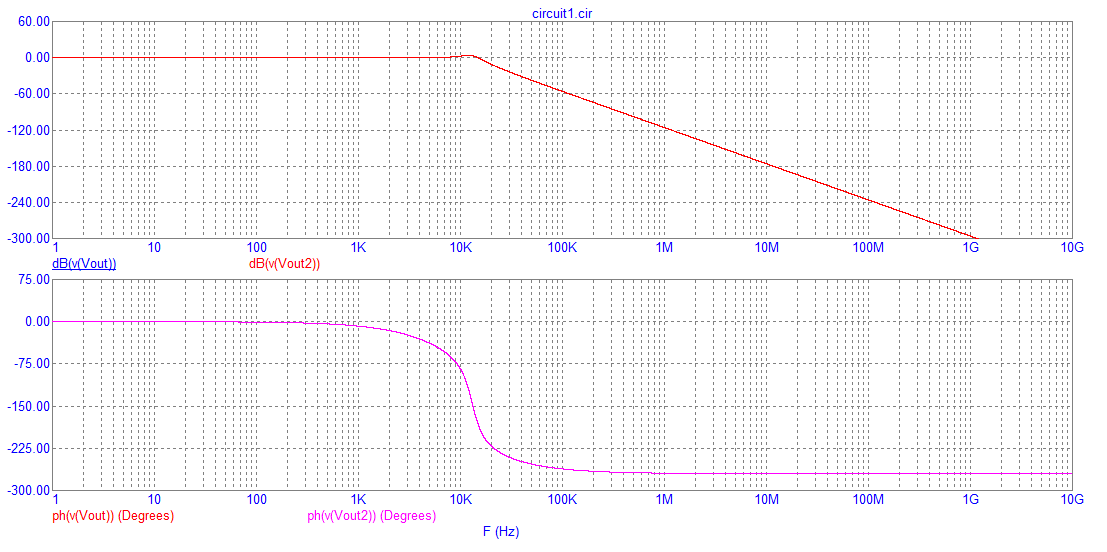
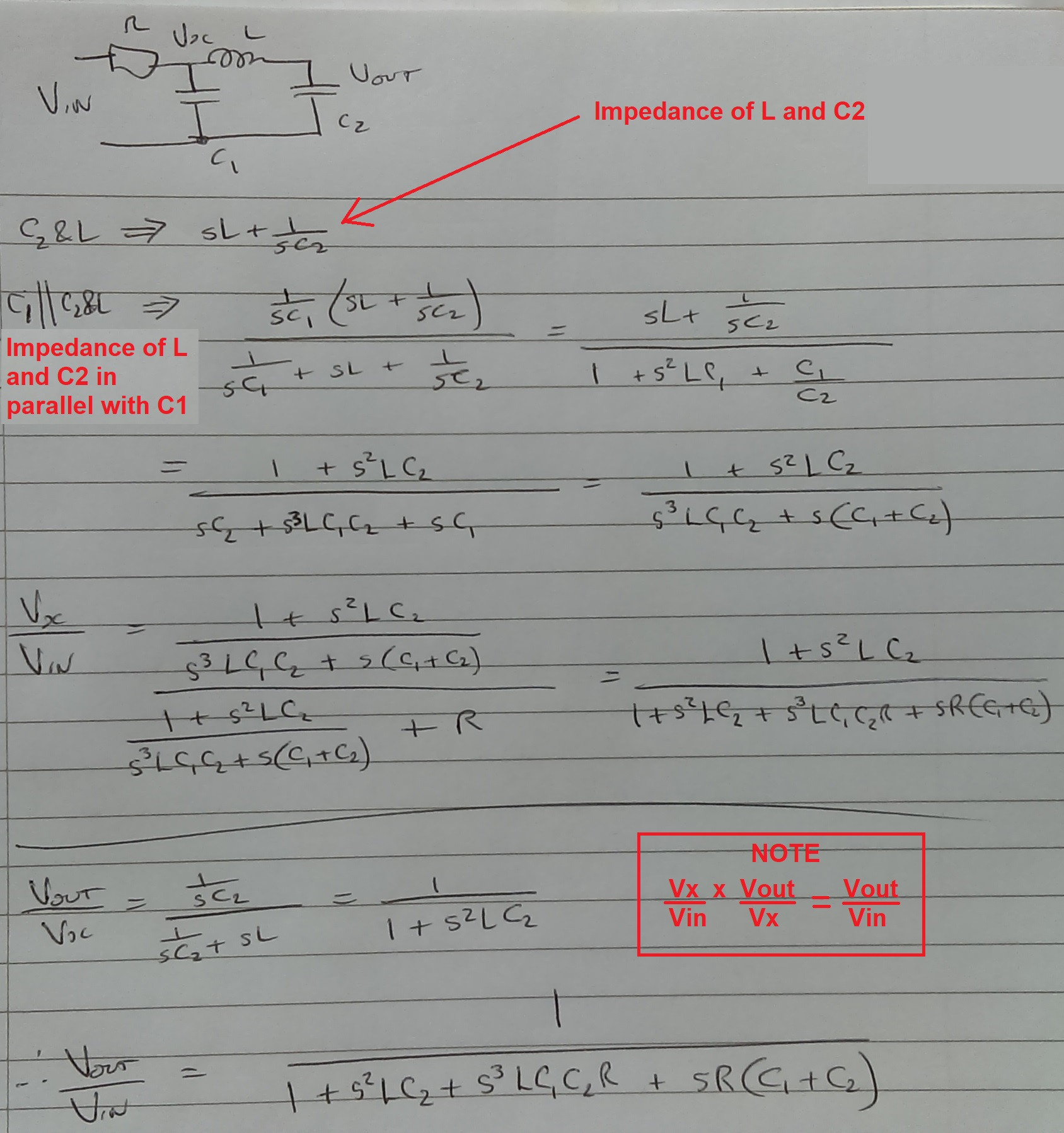
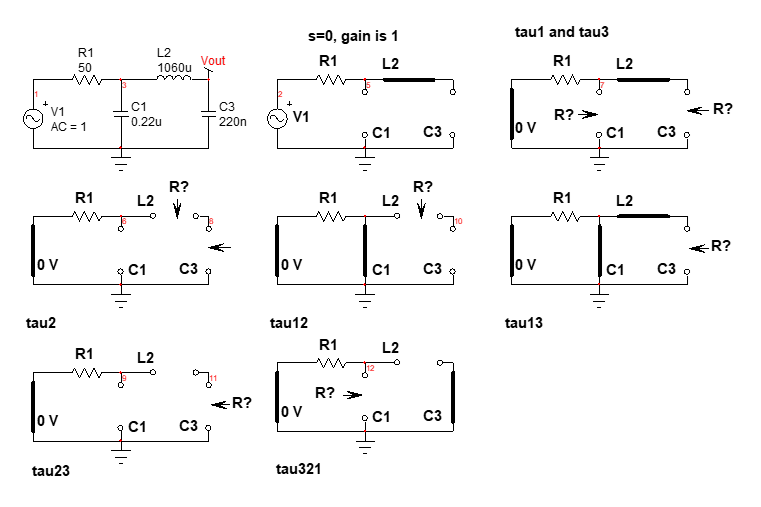



0next to the ground nodes. \$\endgroup\$