BJT average power dissipation

Question

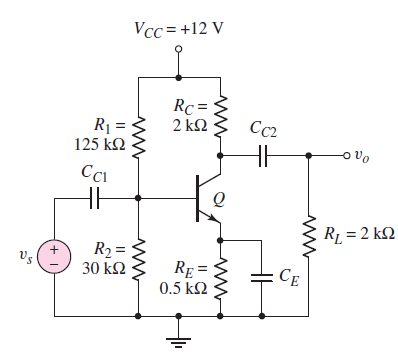
Given the following circuit with \$\beta=80\$, \$V_{BE(on)}=0.7V\$, \$V_A=\infty\$, for an input signal \$V_s=18cos(\omega t) mV\$, I would like to calculate the power dissipated in the transistor.

I began by calculating the thevenin equivalent voltage and resistance in order to solve for the base current as follows:

\$R_{thev} = \frac{R_1 R_2}{R_1+R_2} = \frac{30k\Omega \times 125k\Omega}{30k\Omega + 125k\Omega} = 24.194k\Omega\$

\$V_{thev} = V_{cc} \frac{R_2}{R_1+R_2} = 2.32V\$

The base current can be solved for by writing a KVL equation around the base-emitter loop and substituting \$I_e=(\beta+1)I_b\$:

\$-V_{thev} + R_{thev}I_b + V_{BE(on)} + R_eIe = 0\$

\$-V_{thev} + R_{thev}I_b + V_{BE(on)} + R_eIb(1+\beta) = 0\$

and solving for \$I_b\$:

\$I_b = \frac{V_{thev}-V_{BE(on)}}{R_{thev} + (1+\beta)R_e} = 25.1\mu A\$

Then \$I_c = \beta I_b = (80)(2.006 \mu A) = 2.01mA \$

The collector-emitter voltage can be solved for by writing the equation around the collector emitter loop:

\$V_{CE} = V_{cc} - R_c I_c - R_e I_e = 6.973 V\$

The power dissipated in the transistor is given by

\$PQ = I_{CQ}V_{CEQ}-\frac{1}{2}I^2_cR_c\$

And presumably, the power dissipated in the load is given by

\$PL = \frac{1}{2}I^2_cR_L\$

First I will need to solve \$I_c\$

I write te expression as:

\$I_c = \beta(\frac{R_{thev}}{R_{thev} + R_{ib}})(\frac{V_s}{R_{is}})\$

In the expression above \$R_{ib}\$ is the internal resistance of the transistor in the small signal model given by:

\$R_{ib} = r_\pi + (1+\beta)R_e\$ with \$r_\pi = \frac{V_T}{I_b}\$

\$R_{is} = Rs + R_i\$ (in this case no source resistance was indicated so it is just \$R_i\$)

And \$R_i = R_{thev}||R_{ib}\$

Therefore substituting the above expressions into \$I_c\$

\$I_c = \beta(\frac{R_{thev}}{R_{thev} + r_\pi + (1+\beta)R_e })(\frac{V_s}{R_{thev}||R_{ib}})\$

\$ = (80)(\frac{24194}{24194+40513})(\frac{V_s}{15148}) = 1.974\times 10^{-3} V_s\$

From this I calculate the power dissipated in the transistor to be

\$PQ = I_{CQ}V_{CEQ}-\frac{1}{2}I^2_cR_c = (2.01mA)(6.973V) - 0.5(1.974\times 10^{-3} \times 0.018)^2(2000) = 13.98mW\$

However the textbook answer gives \$13.0mW\$ rather. Where am I going wrong?

Answer 1

In short.

$$P_Q = I_{CQ} \times V_{CEQ} \approx 2\text{mA} \times 6.97\text{V} \approx 13.94 \text{mW}$$

And the AC component of a collector current is equal to

$$i_c = g_m v_{\pi} = 1/13\Omega \times 18 \text{mV} \approx 1.38 \text{mA}$$

Therefore power dissipated in the transistor is:

$$P_Q = I_{CQ}V_{CEQ}-\frac{i^2_c \left(R_C||R_L\right)}{2} = 13.94\text{mW} - \frac{1.38\text{mA}^2 \times 1\text{k}\Omega}{2} \approx 13\text{mW}$$