3
\$\begingroup\$

I have been reading up on network analysis techniques for an upcoming competition, and I was wondering if anyone could be so kind as to derive and explain the system of mesh equations required to solve the following problem:

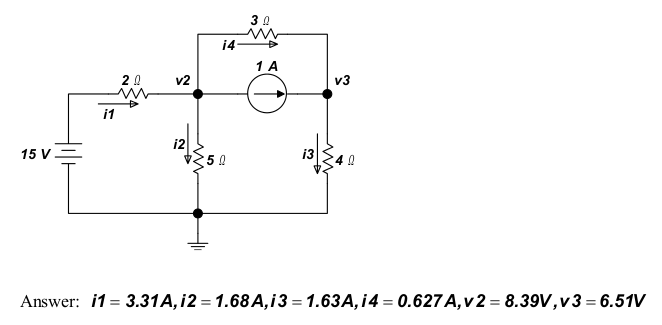
enter image description here

There is an ideal current source present. I want to solve for values that the answers are given for, but I have no idea what setup I should use for the two meshes with the ideal current source. Drawing three clockwise mesh currents i_a for the left loop, i_b for the top right loop, and i_c for the bottom right loop, I have come up (after applying KVL) with the equation

\$ 15=2I_a+5(I_a-I_c) \$

How do I formulate the other equations?

Thanks in advance!

Also, if this does not belong here, I apologize - and please tell me where I should post it!

\$\endgroup\$

3 Answers 3

Reset to default
1
\$\begingroup\$

I have no idea what setup I should use for the two meshes with the ideal current source.

Here's a hint: the current source provides the following equation:

\$ 1A = I_C - I_B \$

This is analogous to having a floating voltage source when doing node voltage analysis. There, you must form a supernode enclosing the voltage source and add the equation relating the voltage between the two nodes enclosed to complete the solution.

Here, you must form a supermesh and add the equation I've given above.

\$\endgroup\$
4
  • \$\begingroup\$ Thank you for the hint and help - it seems I have a bunch of random gaps in my knowledge (I guess it's because I'm doing reading on my own and didn't know where to start) - are there any books you would suggest to me for additional reading on such topics? Thanks! \$\endgroup\$
    – hedgepig
    Commented Dec 11, 2012 at 0:34
  • \$\begingroup\$ @user1230219, have you tried the time tested Schaum's Basic Circuit Analysis? amazon.com/Schaums-Outline-Circuit-Analysis-Second/dp/… \$\endgroup\$
    – Alfred Centauri
    Commented Dec 11, 2012 at 0:44
  • \$\begingroup\$ I will try to check that out at my local library. Thanks! Also, I have formulated the following system based off of your hint - 15=2I_a+5(I_a-I_c); 1=I_c-I_b; 3I_b+4I_c+5(I_c-I_a)=0 Is this correct? \$\endgroup\$
    – hedgepig
    Commented Dec 11, 2012 at 13:32
  • \$\begingroup\$ @user1230219, that's correct. \$\endgroup\$
    – Alfred Centauri
    Commented Dec 11, 2012 at 15:22
1
\$\begingroup\$

Another approach when dealing with multiple independent sources in a circuit is to use the principle of superposition. Consider each source one at a time, and for all the other sources, you "null" them out, replacing each voltage sources with a short circuit and each current source with an open circuit.

Solve the circuit (i.e., find the four unknown currents) for each source in turn in this manner, and then add together the results to get the final answer. It should be the same as any other method.

\$\endgroup\$
3
  • 1
    \$\begingroup\$ I agree that superposition is a wonderful circuit analysis technique that renders many circuit analysis problems trivial. I highly recommend it. Nonetheless, this question is specifically about the technique of Mesh Analysis. Doing a solution "in your head" with superposition will amaze your EE friends but, if you are asked to demonstrate you skill with mesh analysis on an exam or whatever this "competition" is, it's probably safe to assume that solving it another way won't get you points. \$\endgroup\$
    – Alfred Centauri
    Commented Dec 11, 2012 at 1:44
  • \$\begingroup\$ @AlfredCentauri: Different people read the same question differently. In this case, I took it that the OP needed to come up with the answer, and had chosen mesh analysis to do it. I'm offering an alternative. Usually in competitions, it's the answer, not the method, that matters. \$\endgroup\$
    – Dave Tweed
    Commented Dec 11, 2012 at 1:51
  • \$\begingroup\$ My bad, I should have clarified. I meant that I was trying to learn how to use mesh analysis as a technique, but for this competition (Science Olympiad) I really just need to read up as much as I can about the topic of basic DC circuits. Any method of solution is helpful to me though - there is just so much for me to learn. \$\endgroup\$
    – hedgepig
    Commented Dec 11, 2012 at 13:17
0
\$\begingroup\$

You can also convert the current source in a corresponding voltage source as a parallel resistance has been given

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.