How many amps will flow through the cables between my batteries if I have 2 batteries wired in parallel?
Let's look at a simple example:
We've got 2 batteries in parallel, and one 12v lamp that requires 3 amps. The wire from the battery to the lamp will require a wire that can handle 3amps, but how many amps will go through the cables between the batteries in this example?
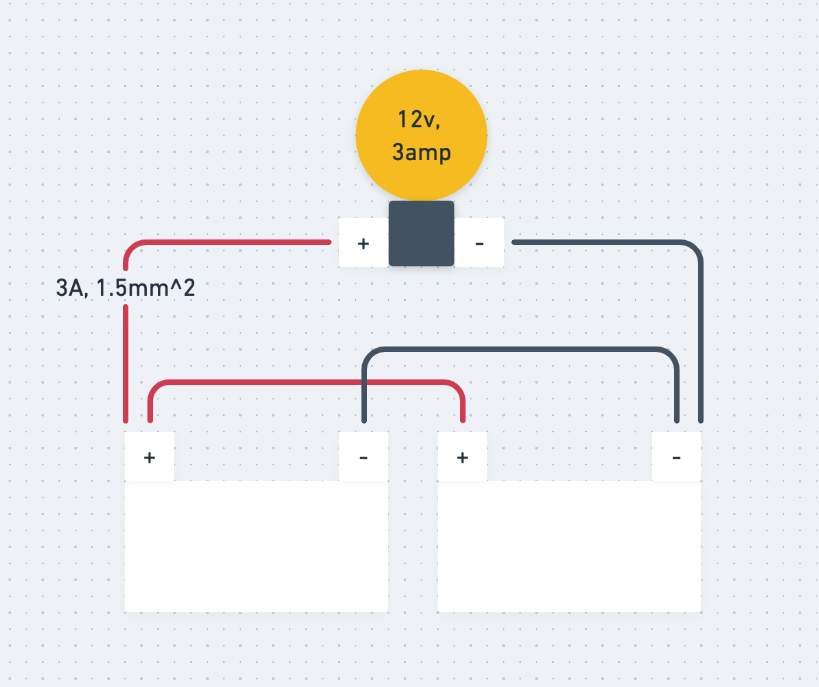
If they are identical batteries with identical charge (an ideal assumption and not the case, but its safe to assume so hypothetically) then half the current will be drawn from both each such that the required 3A comes from 1.5A of each of the batteries - they can be seen as mutually exclusive in the way that the current from the 2nd battery doesnt have to go through the 1st one - i.e. the positive conenctor of 1st battery which connects to the 2nd battery wire AND the 3A wire, is thick enough to support the 3A so the 1st battery and 2nd each give out 1.5A to a seemingly 1.5A load each. Because of this, the wire from 1st to 2nd (essentially the same wire as the 2nd to the connector to the 3A wire) should handle 1.5A minimum.
This is, again, assuming both batteries uniformly give 1.5A. Voltage imbalances (which wouldn't happen as they are in parallel, but nonetheless there are other chemical imbalances) mean that there might be slight differences in the current draw. For safety I would personally use 2A wire to account for any current imbalances.
To answer your question in at the start of your post, though; if the load requires 3A at 12 V (and assuming your power source can provide this without dropping voltage - a couple of car batteries should do it just fine) it will draw 3A. Just as much as it needs, despite the power source being able to supply more. You could connect 10 car batteries in parallel and it would still only draw 3A in total because that's all it needs (in this case, each one would supply 0.3 A if they are identical)
If the batteries are identical, one battery provides half the current. If they are not identical, e.g. one battery is dead or missing, full 3A. If you connect two batteries together that are unequally charged, e.g. one full and one empty, any arbitrarily large current would flow to equalize the charge levels. Prepare with good margin and fuses.
