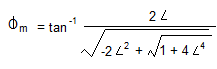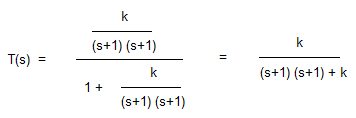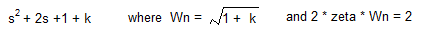Decide P controller gain K so that phase margin is 50°

Question

I need help finding the gain \$K\$ so that the phase margin for the system equals 50°.

$$F(s) = K$$

$$G(s) = \dfrac{1}{(s+1)^2}$$

\$v\$ is a process disturbance sinusoid with amplitude 2.5 and freqency 0.5 rad/s. Image below.

Now I need to find K so that the phase margin = 50°. I tried:

\$\varphi_m = 180^\circ\$ \$+ \arg G(i\omega_c) + \arg F(i\omega_c) = 50^\circ\$

and solve for \$\omega_c\$ but I can't really figure out how to. Do I have to account for the disturbance as well? If so, why? If not, why not?

Answer 1

From you question I understand that you have written one equation and have unknown variables in it; viz, \$\omega_c\$ and K. If you write one more equation, you may be able to solve for K. To that end,

Definition of gain cross over frequency is

\$ \begin{align} |GF|_{s=j\omega_c} ={}& 1\\ \frac{K}{|1+j\omega_c|^2} ={}& 1\\ K^2={}& (1+\omega_c^2)^2 \\ \end{align}\$

Solve for \$\omega_c\$ in terms of K.

In the phase equation you already have with you, substitute \$\omega_c\$ with the expression containing K. Now that equation has only one unknown, viz. K. Solve!

Answer 2

I don't know if the following method is the easiest technique to find k given phase margin or not.

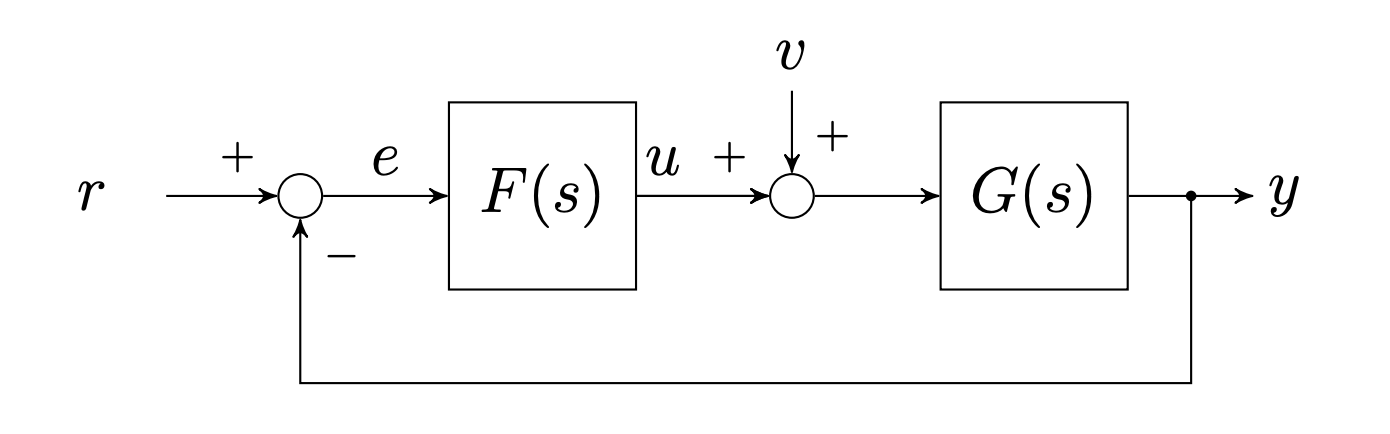
The following equation gives phase margin when damping ratio, zeta is known. We want to know zeta given phase margin but I had trouble trying to make zeta the subject, so I used the equation as shown and entered several values for zeta until I converged on a phase margin of 50 degrees.

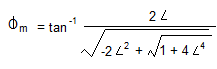
It didn't take long to find that a value for zeta of 0.478 gives a phase margin of 50 degrees.

Next we derive the closed loop transfer function which is:-

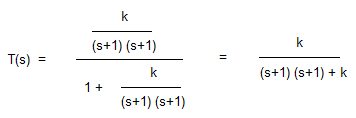
Next we look at the characteristic equation and, using the Root Locus technique, we plot the loci of the closed loop poles as k is varied from zero to infinity. The two loci start at (-1,j0) because, for k=0, we have two real and identical poles meaning that for k=0, zeta =1 and we have critical damping. As k is increased in value the poles become a pair of complex conjugate poles and move away from each other to infinity parallel to the jw axis.

The characteristic equation, which we solve for values of k from 0 to infinity, is:

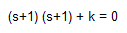
The loci of the poles on the s plane look like this:

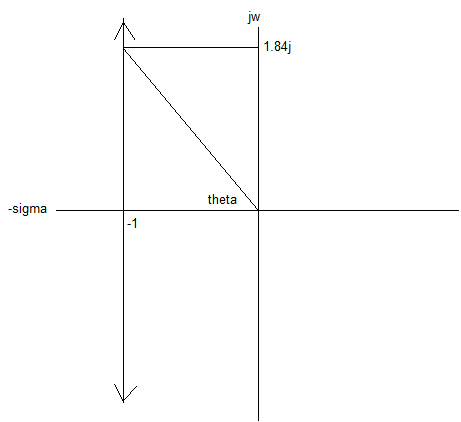
Now the damping ratio is the cosine of the angle (theta) which the vector between the origin and a particular pole position makes with the real axis. For a value for zeta of 0.478 we have theta = 61.45 degrees which, by simple trigonometry, gives a jw value of 1.8j (incidentally this is the damped natural frequency, Wd). So our pole positions, for zeta = 0.478, are (-1,+j1.8) and (-1,-j1.8).

Now, to find the value of k which gives these pole positions on the root locus we simply take the distances from the two open loop poles to the closed loop poles and multiply them together. The open loop poles are identical to the closed loop poles when k=0 that is to say the open loop pole positions are at the start of the root locus.

So, k = 1.84 * 1.84 = 3.38

Adding a simplification as an after thought.

Once you have a value for zeta and the closed loop transfer function it's possible to determine k just by examining the denominator of the closed loop transfer function.

The denominator of the closed loop transfer function is:

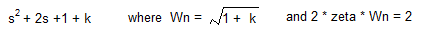
We know zeta and so we can solve for k which, again gives a value for k of 3.38

Answer 3

By far the easiest way is to investigate the open loop transfer function \$H_\text{ol}(s) = KG(s) \$. Initially, set \$K=1\$ and look at the Bode Plot: -

s = tf('s');
G = 1/(s+1)^2
K = 1
margin(G*K)

For \$50°\$ phase margin the crossover frequency has to be \$\omega_c = 2.14\: \text{rad/s}\$, as seen from the Bode Plot. This is achieved by increasing the gain to \$K=10^{\frac{14.95}{20}}\$: -