2
\$\begingroup\$

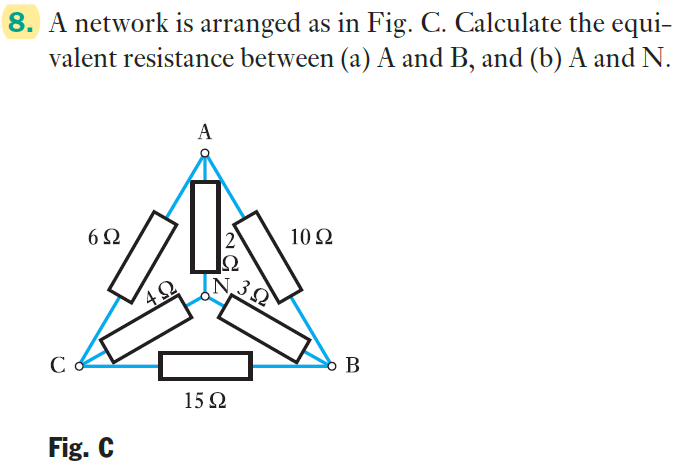
This post is regarding exersise 4-8 from the following book: Hughes Electrical And Electronics Technology 10th Ed.

Here it is:

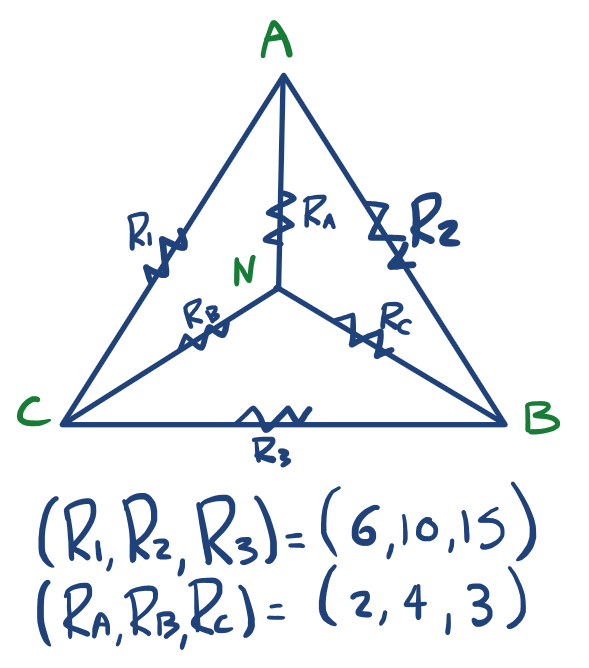
enter image description here

Let's focus on what I tried for solving part (b) :

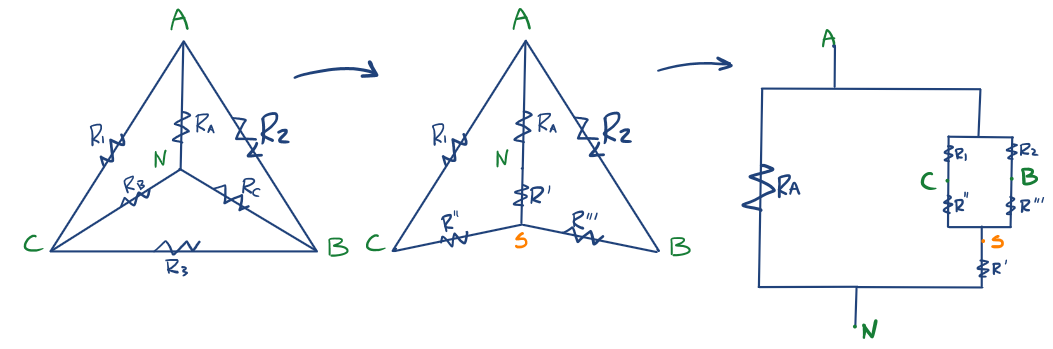
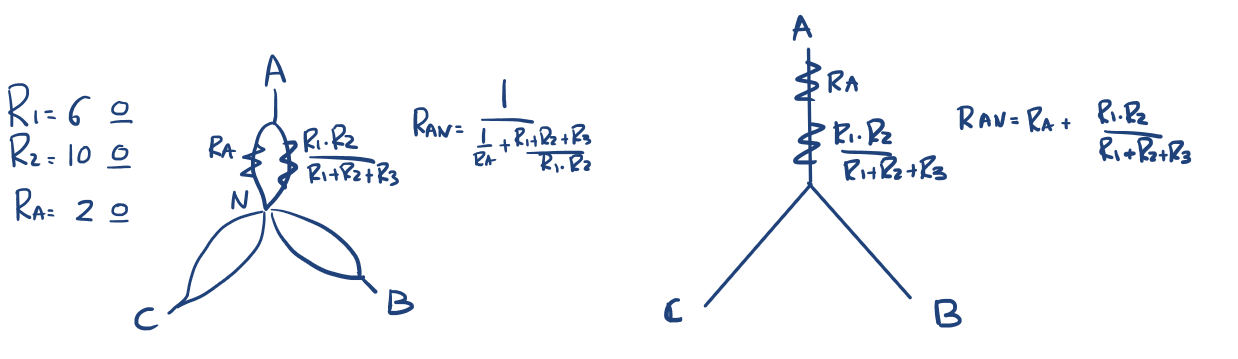
Since this is an exercise under the chapter for delta-star transformations my first thought was to try and perform some such transformation. However this was a combination of both star and delta connections so I didnt really know whether the resulting resistances would be in series / parallel or neither, with the already existing ones, so I tried both series and parallel: enter image description here

However neither worked.

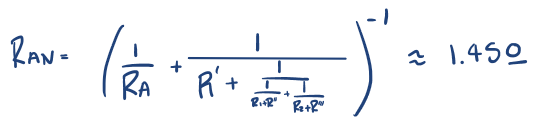
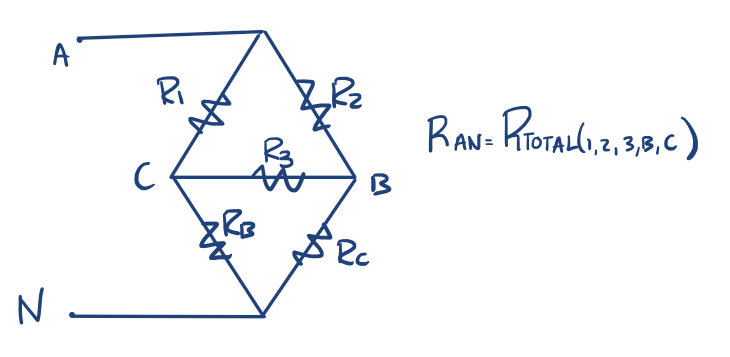
My next thought was that maybe I have understood something very badly about the resulting resistance of two points in a circuit. So after a little bit of digging I tried the following: enter image description here

However I again got a wrong result in regards to the given answers at the end of the book.

Conclusion:

  • Which approach (if any) was correct?
  • If no approach was correct, which concept do I need to understand better?

Any help/hint is very much appreciated

\$\endgroup\$
5
  • \$\begingroup\$ I don't think any of your approaches makes sense and also you didn't explain anything how you draw these equivalent circuits \$\endgroup\$
    – user215805
    Commented Oct 3, 2020 at 17:35
  • \$\begingroup\$ Take the delta, transform into a star. You have two start in parallel which is easy to simplify. Or take the star. transform into a delta. Two deltas in parallel. Or both, transform the final delta into a star (or vice versa) to check you get the same answer. \$\endgroup\$
    – user16324
    Commented Oct 3, 2020 at 18:33
  • \$\begingroup\$ @BrianDrummond this is what it tried(first picture), i guess i made some computational error, will try again \$\endgroup\$
    – Stamatis
    Commented Oct 4, 2020 at 7:16
  • \$\begingroup\$ @BrianDrummond I have concluded that i have not made any computational mistake, the resulting Resistance from A to N turn out to be 0.98 ohm with this method, which by the book is wrong, it should be 1.45 ohms \$\endgroup\$
    – Stamatis
    Commented Oct 5, 2020 at 9:14
  • 1
    \$\begingroup\$ @BrianDrummond it worked for part (a) however so I am clearly doing somethiing wrong \$\endgroup\$
    – Stamatis
    Commented Oct 5, 2020 at 9:29

2 Answers 2

Reset to default
0
\$\begingroup\$

To calculate equivalent resistance between A and N one approach would be -

1.look at NCB which is in Delta connected form

2.make NCB in star connection from delta by conversion formula

after that conversion you'll get a very simlified circuit which is very easy to solve

\$\endgroup\$
1
  • \$\begingroup\$ I see what you mean, and it worked, I posted the full solution as an answer here. Thank you so much! \$\endgroup\$
    – Stamatis
    Commented Oct 5, 2020 at 10:58
1
\$\begingroup\$

I figured it out, this is the answer for part(b) "Finding Equivalent resistance from A to N".

First of all, here is a nicer sketch:

enter image description here

Now we will focus at the delta connection defined by N , B , C :

enter image description here

And now if we go back to our original sketch and replace the converted circuit we can greatly simplify it:

enter image description here

Finally, we are ready to compute the equivalent resistance from A to N:

enter image description here

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.