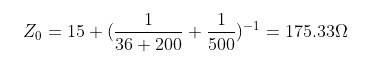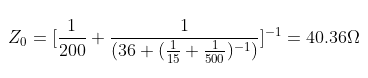Deriving the S-parameters, different Matched Loads possible?

Question

I guess this is more of a conceptual question but for the problem below Im having trouble getting started on how to derive the S parameters for the network.

I noticed that the network at 28.025 GHz is resonant, so the shunt capacitor and inductor are open, leaving the equivalent circuit at that frequency to be just the resistors

To derive the s parameters I understand that I have to terminate a port with a matched load, at either 1 or 2 to so that the Reflection Coefficient = 0

Now here is a my confusion, it seems to me that going from port 1 to 2 the characteristic impedance of the circuit (Z_0) would be different than going from port 2 to port 1 leading to different matched loads.

I think that they are different because if you inject a signal into port 1 and short port 2 to ground you encounter an impedance of:

But if you inject a signal starting at port 2 you get:

I believe there's a high chance of doing those resistance calculations wrong, but Im just not sure where to start or if im headed in the right path.

Which boils down my question to

Is it true that the Characteristic Impedance of this network changes depending on where you inject the signal, leading to different matching loads? If not how do you calculate the system impedance this network in particular?

My professor in class has always shown examples and given us homework of simple two port networks that look symmetrical from either port where the system impedance is the same whether looking into port one or port 2 matched loads will be equal so im pretty confused right now.

Answer 1

It is possible to define S-parameters for networks intended to be used between systems of different impedance, for instance transformers or pads intended to work between 50 Ω and 75 Ω systems.

However, when you are asked without further clarification to compute the S-parameters of a network, you should assume it's being used in a 50 Ω system.

There may be many practical reasons a network does not present a good match to a 50 Ω system. Perhaps it's actually intended as an antenna match.

Regardless of what actual impedance a 2-port is intended to present, and regardless of what terminates the other port, the S-parameters can be combined so that the measurement in the 50 Ω system can be used to design for other impedances.

You are not being asked to compute the system impedance of this particular network. What you are being asked to do is

a) Terminate port 2 in 50 Ω
b) Drive port 1 with a 50 Ω source
c) Compute S21 as the ratio output/input
d) Compute S11 as the ratio reflected/input
e) Swap ports 1 and 2, and do the same for S22 and S12

Answer 2

I think that they are different because if you inject a signal into port 1 and short port 2 to ground you encounter an impedance of:

First, you are not calculating \$Z_0\$ here. \$Z_0\$ is a given of the problem, typically 50 or 75 ohms, but in principle it could be chosen arbitrarily for your system. What you're calculating here is \$Z_{in}\$ the input impedance.

Second, if you short port 2, then the 200-ohm resistor is shorted, and shouldn't appear in the equation for \$Z_{in}\$. The actual equation with port 2 shorted would just be

$$Z_{in}=15+ (36||500)$$

Third, if you want to calculate the S-parameters, you should be terminating port 2 with the system characteristic impedance (again, usually 50 or 75 ohms, but possibly arbitrarily chosen by your instructor or the system designer), not with a short.

Is it true that the Characteristic Impedance of this network changes depending on where you inject the signal, leading to different matching loads?

No, the characteristic impedance is a system-level parameter that needs to be chosen before you design or analyze the elements in the system.

If the 2-port isn't well-matched to the system \$Z_0\$ then that just means the 2-port isn't well matched. It doesn't mean the system \$Z_0\$ has changed.

Answer 3

S11=0.236+j2.75u (50 ohm)

S12=0.53+j5.81u (50 ohm)

S21=0.53+j5.81u (50 ohm)

S22=-0.081+j12.4u (75 ohm)

Yes, it's reciprocal.