Deriving equivalent impedance of circuit with pi sections

Question

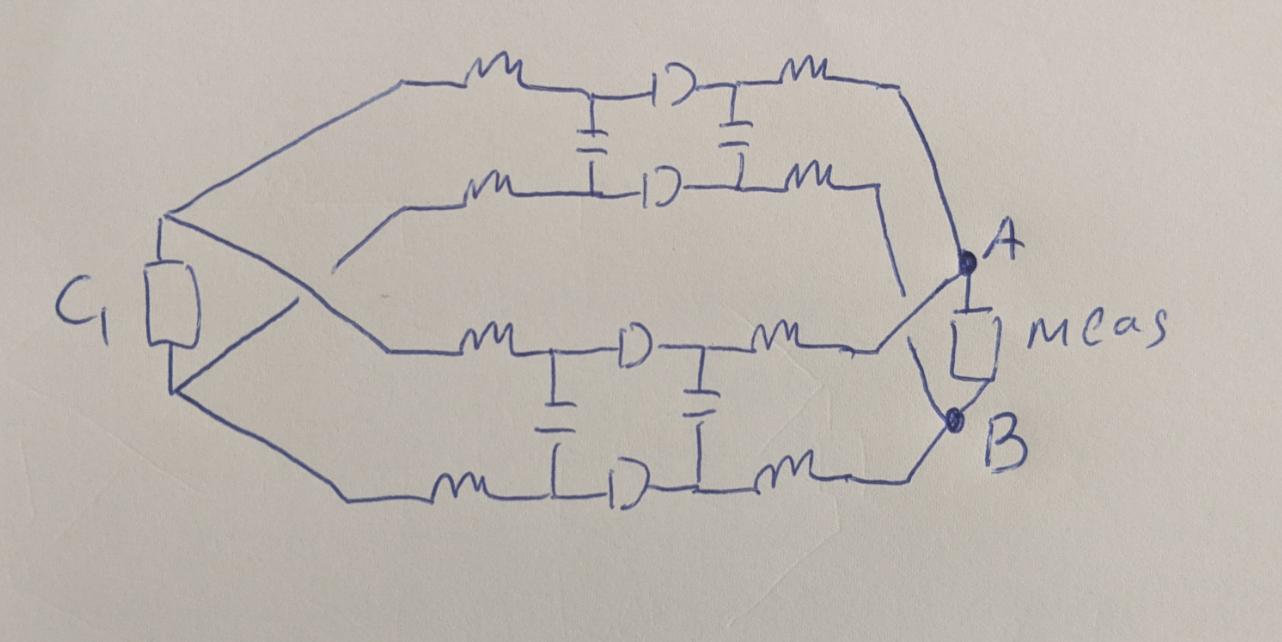
Hello everyone, I am currently trying to find the equivalent impedance of this circuit. The circuit consist of a load C1 which are connected to a two pole pi section with line inductors which are in parallel and at then end is just some sort of measurement block.

Was wondering if finding the equivalent impedance would be as simple calculating the parallel of the two line inductors and then adding in series with the load. After that the resulting impedance would be calculated in parallel with the capacitors from both pi sections.

Is this approach correct or should I do it differently?

Answer 1

It will work if you apply the following reasoning, provided the network has two equivalent, symmetrical branches:

• all inductances will be halvened
• all capacitances will be doubled

This is a random example consisting of symmetrical LCL input, series LC, parallel LC. If the branches are not symmetric, trying to use the parallel-series equivalences will not work, due to the different loading effect on each branch. Don't forget that you're dealing with a passive network, so all the elements influence each other (more or less).

---

To be more precise about the nature of the imbalance: if two networks are placed on each branch and the elements are symmetrical, then the transfer function ends up with doubled poles and zeroes, which can be transformed into a single, equivalent branch, due to the order of the transfer function ending up the same.

But if the two brances are different then the number of poles and zeroes are now doubled, and a single branch can only have half their numbers, therefore the equivalence is impossible.