Transfer function in ideal op-amp

Question

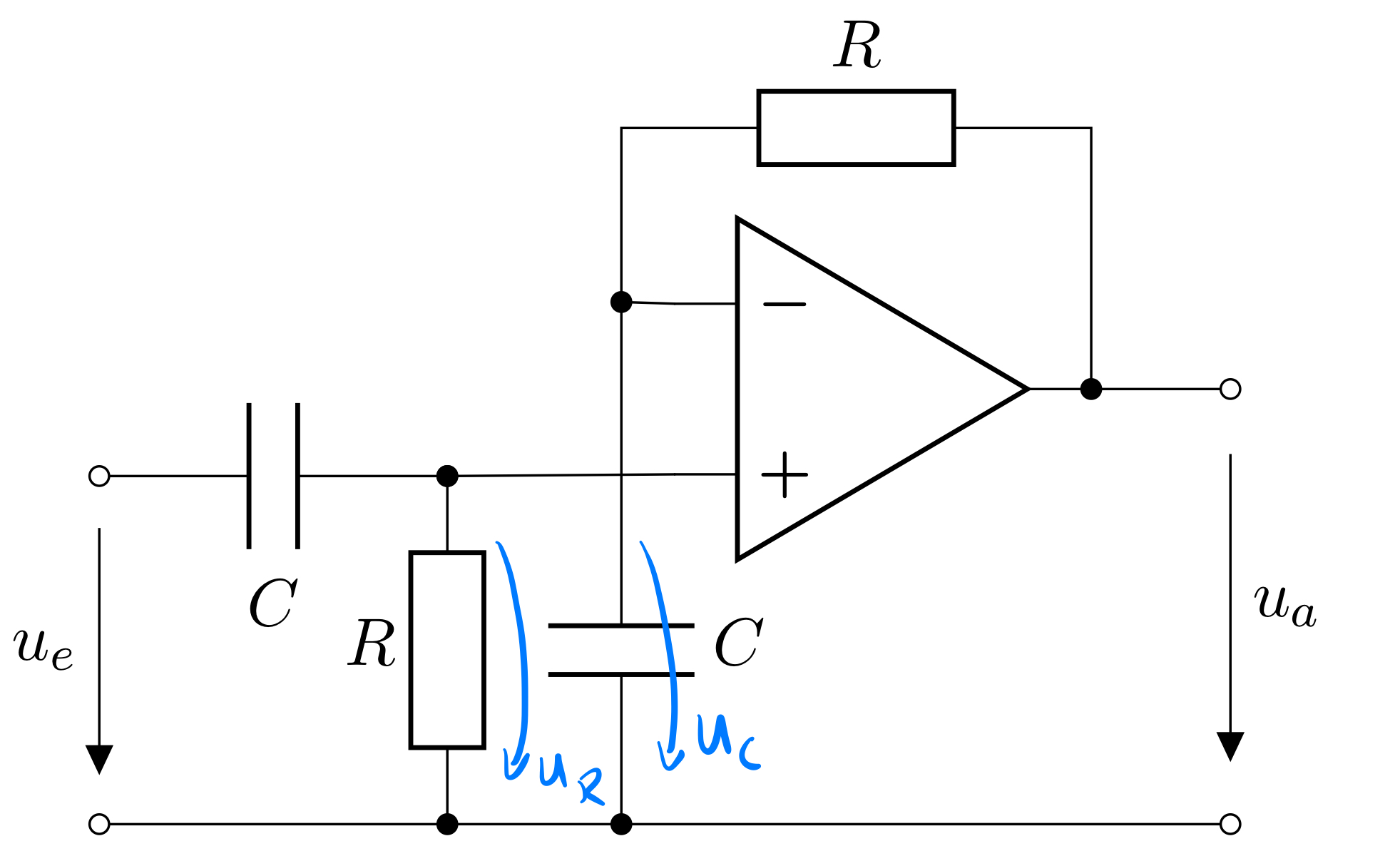
I am new to electrical engineering and came across one problem I could not solve:

I shall find the transfer function \$G(jw)\$ with \$G(jw) = \frac{U_A(jw)}{U_E(jw)}\$ of the ideal op-amp.

My solution:

$$U_{R(s)} = \frac{R}{(sC+R)} * U_{E(s)}$$

$$U_{C(s)} = \frac{sC}{(sC+R)} * U_{A(s)}$$

$$U_{C(s)} = U_{R(s)}$$

$$\frac{U_{A(s)}}{U_{E(s)}} = \frac{R}{sC}$$

with \$s = jw\$

The correct answer is \$G(jw) = jwRC\$.

What am I missing?

Answer 1

Well, using the voltage divider formula we can see that:

• $$\text{v}_+\left(\text{s}\right)=\frac{\displaystyle\text{R}}{\displaystyle\text{R}+\frac{1}{\text{sC}}}\cdot\text{v}_\text{in}\left(\text{s}\right)\tag1$$
• $$\text{v}_-\left(\text{s}\right)=\frac{\displaystyle\frac{1}{\text{sC}}}{\displaystyle\frac{1}{\text{sC}}+\text{R}}\cdot\text{v}_\text{out}\left(\text{s}\right)\tag2$$

For an ideal opamp we know that \$\text{v}_+\left(\text{s}\right)=\text{v}_-\left(\text{s}\right)\$, so:

$$\frac{\displaystyle\text{R}}{\displaystyle\text{R}+\frac{1}{\text{sC}}}\cdot\text{v}_\text{in}\left(\text{s}\right)=\frac{\displaystyle\frac{1}{\text{sC}}}{\displaystyle\frac{1}{\text{sC}}+\text{R}}\cdot\text{v}_\text{out}\left(\text{s}\right)\space\Longleftrightarrow\space\frac{\text{v}_\text{out}\left(\text{s}\right)}{\text{v}_\text{in}\left(\text{s}\right)}=\frac{\displaystyle\frac{\text{R}}{\displaystyle\text{R}+\frac{1}{\text{sC}}}}{\displaystyle\frac{\displaystyle\frac{1}{\text{sC}}}{\displaystyle\frac{1}{\text{sC}}+\text{R}}}=\text{CRs}\tag3$$