Is there a practical use for the Fibonacci sequence or similar fractal/sequence in Electrical Engineering?

Question

One use of Fractal Engineering is in Fractal Antennas:

An Overview of Fractal Antenna Engineering Research

Abstract:

Recent efforts by several researchers around the world to combine fractal geometry with electromagnetic theory have led to a plethora of new and innovative antenna designs. In this report, we provide a comprehensive overview of recent developments in the rapidly growing field of fractal antenna engineering. Fractal antenna engineering research has been primarily focused in two areas: the first deals with the analysis and design of fractal antenna elements, and the second concerns the application of fractal concepts to the design of antenna arrays. Fractals have no characteristic size, and are generally composed of many copies of themselves at different scales. These unique properties of fractals have been exploited in order to develop a new class of antenna-element designs that are multi-band and/or compact in size. On the other hand, fractal arrays are a subset of thinned arrays, and have been shown to possess several highly desirable properties, including multi-band performance, low sidelobe levels, and the ability to develop rapid beamforming algorithms based on the recursive nature of fractals. Fractal elements and arrays are also ideal candidates for use in reconfigurable systems. Finally, we provide a brief summary of recent work in the related area of fractal frequency-selective surfaces.

And another use of Fractals, this time Engineered as an improved cooling method is this MRI improvement:

Goodbye wires and formers: 3-D additive manufacturing and fractal cooling applied to construction of MRI gradient coils

Abstract:

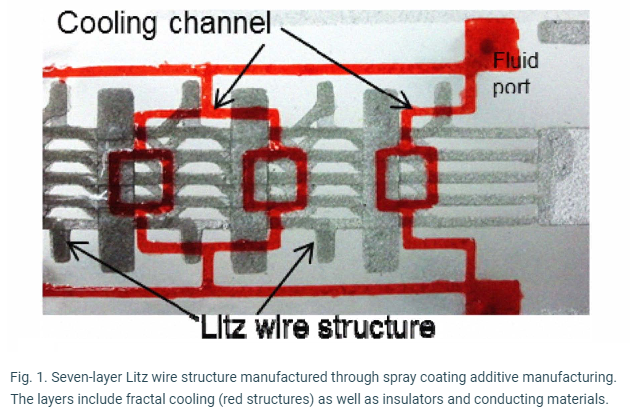
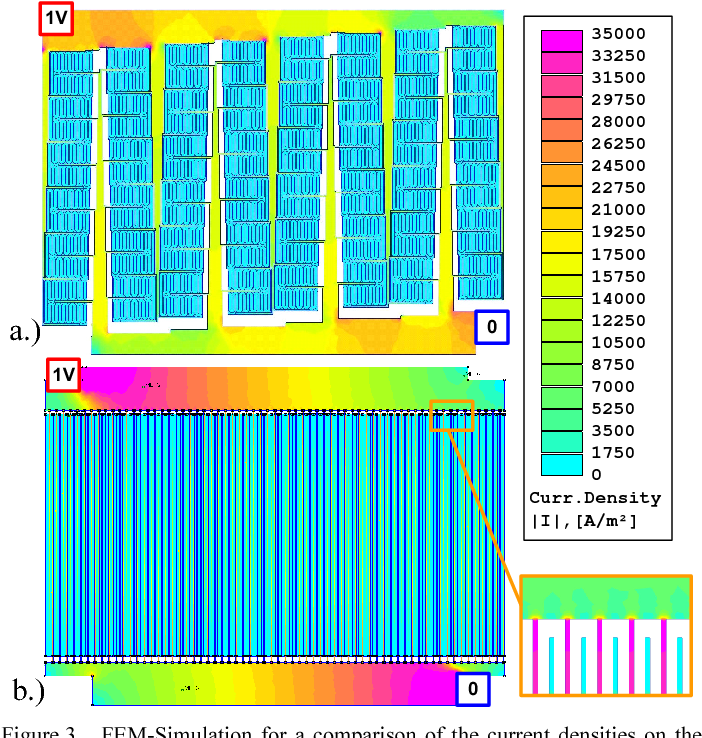
The high pulse frequencies employed in MRI gradient and RF coils call for the use of dedicated construction techniques involving special wires and cooling systems. These requirements are needed because conventional (e.g., solid-core) wires exhibit skin effects at frequencies above 10 kHz, which effectively concentrate all the current in the periphery of the wire, leading to heating losses due to high resistance. To mitigate the resistance problem due to skin-depth, many gradient coils (and some RF coils) employ cords of twisted and/or woven thin insulated wires (e.g., Litz wires) that force currents to traverse the entire wire cross-section. Litz wires are hard to configure into the complex designs required for gradient coils, due to a minimum turning radius of several millimeters and the asymmetric bending forces required for winding the wires onto formers. Another challenge in MRI gradient coil manufacturing is the ability to cool RF and gradient coils, especially at high pulse rates. Our approach to this problem has been to replace traditional wire-coil construction methodology with multi-layer additive manufacturing methods, which lend themselves to design and manufacture automation. Additive manufacturing can enable dramatic (i.e., nearly three-fold) improvement in cooling efficiency, through the use of bio-mimetic fractal approaches. Building gradient and/or RF coils layer by layer, we have added conductive, insulating and cooling elements with appropriate interconnects as necessary. A prototype multi-layer Litz wire structure was developed, with fractal cooling, which showed superior performance (in terms of 80% reduced resistive losses at high frequency) to the comparable non-Litz wire configuration.

Fractal cooling patter for MRI circuit:

I would like to know more practical uses for this technology, with a preferred focus on Fibonacci sequence, if possible.

Answer 1

It looks like this paper uses fractals to prevent current crowding in a high power MOSFET.

In high power transistor design, you want to maximize current by minimizing resistance.

If you start with a single basic transistor (fist order) with two metalization finger contacts, the wider the transistor is, the lower the transistor active region resistance is. Conversely, your metalization resistance increases because the fingers are getting longer as. You can increase the metal finger thickness to combat this, but then you're simply scaling the entire structure in both the X and Y direction to increase the current. Instead, if we add fingers to the initial two fingers (beginning the fractal), the active area resistance can drop because the transistor channels are now much wider than they are across. The author then extends this into a 2-D pattern for even more efficiency.

The issue is on-resistance would now be dominated by metalization and the current at any specific section of the transistor would become highly non-uniform as shown in their Figure 3.

In figure 3B, they show a comb-structure (2nd order) and show the highly non-uniform current crowding that occurs. To resolve that, they go further beyond 2nd order and add in metalization that's tapered to help support uniform current transfer between each side of the transistor in Figure 3a.

Answer 2

I thought I had stumbled on a way to use Fibonacci to filter the input/output of a power converter, by using LC filters according to a Fibonacci progression of values, but as was pointed out to me, my example did not necessarily prove anything.

Therefore, in place of my original answer, I have improved my answer by merely providing one good example of Fibonacci technology in Electrical Engineering, of which you may not be aware. I hope you like it.

Modified Fibonacci Search Based MPPT Scheme for SPVA Under Partial Shaded Conditions

Abstract:

This paper presents the modified Fibonacci search based MPPT scheme for a solar photo voltaic array (SPVA) under partial shaded conditions. The real Fibonacci search based MPPT fails to track the global peak (GP) under partial shaded conditions. This paper improves the method so that the proposed method tracks GP for all the shaded conditions. It is checked for different shading patterns through simulation and verified experimentally.